Convexity of distance-to-boundary function

Question

Let $\Omega\subset\mathbb{R}^{n}$ be an open, bounded convex domain. Denote $d_{\Omega}:\Omega\rightarrow\mathbb{R}$ the distance-to-boundary function, that is, $$ d_{\Omega}\left(x\right):=\inf\left\{ \left|x-y\right|:y\in\partial\Omega\right\} . $$ It is well-known that if $\partial\Omega$ is smooth then $d_{\Omega}$ is convex in a neighborhood of $\partial\Omega$ and $\left|\nabla d_{\Omega}\right|=1$ in this neighborhood.

I would like to reduce the smoothness assumption of the boundary. My question (suggestion) is: if $\partial\Omega$ is only Lipschitz, is it true that $d_{\Omega}$ is convex in a neighborhood of $\partial\Omega$ and $\left|\nabla d_{\Omega}\right|=1$ almost everywhere in this neighborhood?

Any reference is welcome.

Answer 1

The proof does not require smoothness.

Assume $B(x,r_x), B(y,r_y)\subset \Omega$. It is sufficient to show that $$B(\tfrac{x+y}2,\tfrac{r_x+r_y}2)\subset \Omega.$$ The latter follows from the convexity of $\Omega$. Indeed, the ball $B(\tfrac{x+y}2,\tfrac{r_x+r_y}2)$ is the set of midpoints between $B(x,r_x)$ and $B(y,r_y)$.

The second condition, $|\nabla \textrm{dist}_{\partial\Omega}|\stackrel{ae}{=}1$ holds for all open domains --- nothing is needed. Indeed, $\textrm{dist}_{\partial\Omega}$ is $1$-Lipschitz. Note that if $\textrm{dist}_{\partial\Omega}$ is differentiable at $x$, then $|\nabla_x \textrm{dist}_{\partial\Omega}|\le 1$. Now, by Rademacher's theorem, $\textrm{dist}_{\partial\Omega}$ is differentiable almost everywhere. Assume that $v$ is the unit vector in the direction of a shortest way from $x$ to the boundary. Then $(d\textrm{dist}_{\partial\Omega})(v)=-1$; therefore, $|\nabla_x \textrm{dist}_{\partial\Omega}|\ge 1$.

Answer 2

It is not true in general that $d_\Omega$ is convex, however smooth the boundary is. E.g., if $n\ge2$ and $\Omega$ is the unit ball centered at the origin, then $d_\Omega(x)=1-|x|$ is concave in $x\in\Omega$, and it is not convex in $x$ in any neighborhood of the boundary.

Answer 3

This is an extension of my comment to Anton Petrunin's answer, as requested by erz. Similar calculations are contained in most textbooks on PDEs (e.g. Gilbarg–Trudinger), and I guess one can find a detailed answer in some textbooks on convex bodies.

---

Claim 1: We have $|\nabla \operatorname{dist}(x_0, \partial \Omega)| = 1$ if the maximal ball $B(x_0,r)$ contained in $\Omega$ touches the boundary of $\Omega$ at one point only.

(Edited; the first version of the proof was simpler, but incomplete; the following one is much clearer if one makes a picture on the fly).

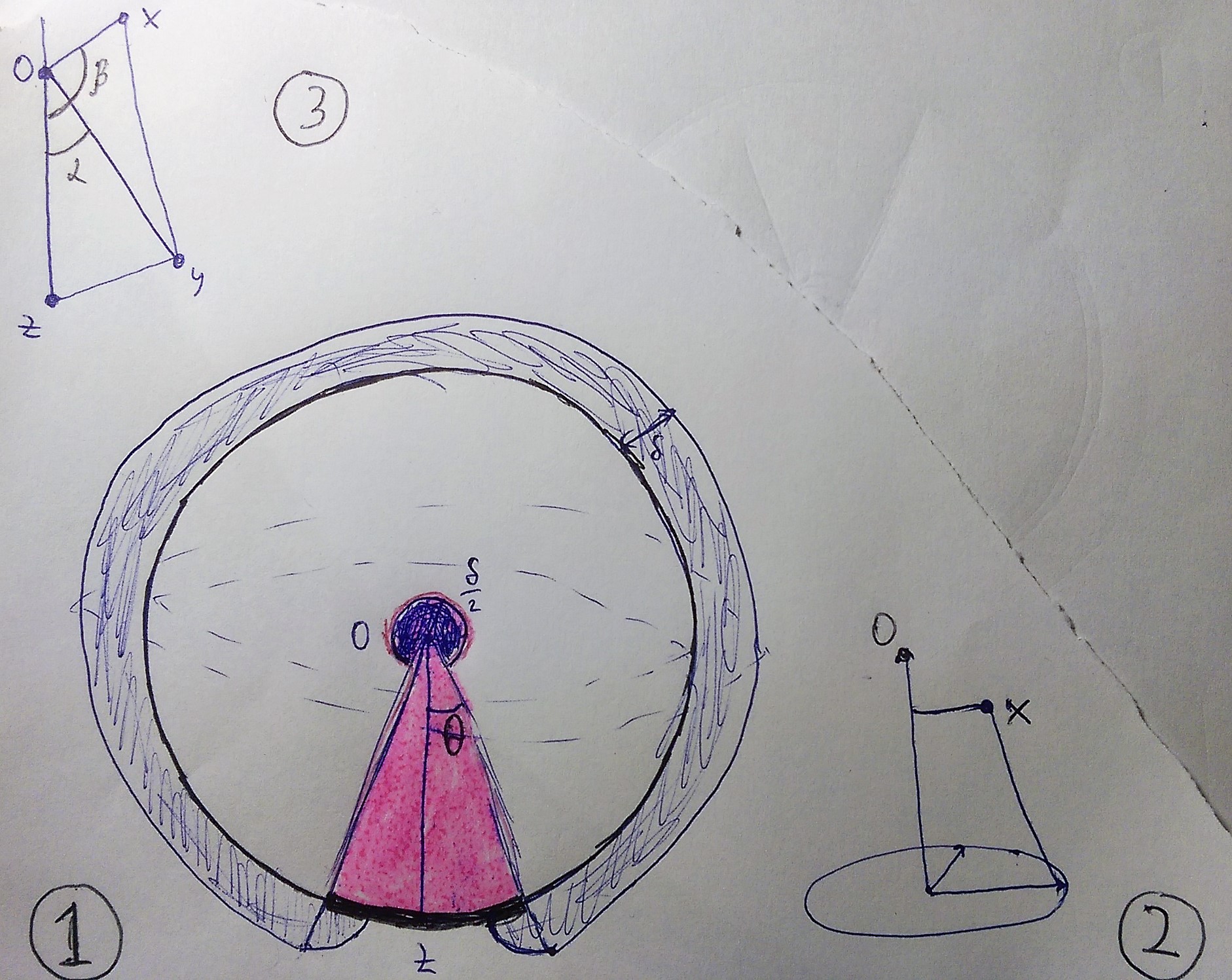
Let $x_0 \in \Omega$, let $B(x_0, r)$ be the largest ball centred at $x_0$ contained in $\Omega$, and denote by $z$ the unique common point of $\partial B(x_0,r)$ and $\partial \Omega$.

To simplify the notation, with no loss of generality suppose that $x_0$ is the origin $0$, and that $z = (0, \ldots, 0, -r)$. For $x \in \mathbb{R}^d$ we write $x = (\tilde{x}, x_n)$ with $\tilde{x} \in \mathbb{R}^{n-1}$, $x_n \in \mathbb{R}$. In particular, $z = (\tilde{0}, -r)$.

Necessarily $\Omega$ is contained in the upper half-space $\{x_n > -r\}$. Therefore, $$\operatorname{dist}(x, \partial \Omega) \leqslant x_n + r. \tag{1} $$ On the other hand, let $a = \sqrt{1 + \delta^2} > 1$ be a number close to $1$. We will later show that for $t \geqslant 0$ small enough, say $t \in [0, t_0]$, $\Omega$ contains $$B_t = B((\tilde{0}, a t), r + t) .$$ Note that these balls are tangent to the cone $$C_a = \{x \in \mathbb{R}^n : x_n > |\tilde{x}| - (a - 1) r\} .$$ Before we prove this claim, let us see how it implies Claim 1. For $x$ sufficiently close to $0$ we have $$\operatorname{dist}(x, \partial \Omega) \geqslant \operatorname{dist}(x, \partial B_t) = r + t - |x - (\tilde{0}, a t)| = r + t - \sqrt{|\tilde{x}|^2 + (x_n - a t)^2} $$ for all $t \in [0, t_0]$. If $x_n < 0$ and $|\tilde{x}| < -\delta x_n$, we choose $t = 0$ and we get $$\operatorname{dist}(x, \partial \Omega) \geqslant r - \sqrt{|\tilde{x}|^2 + x_n^2} \geqslant r - \sqrt{\delta^2 + 1} x_n = r - a |x_n| = r + a x_n. $$ Otherwise we choose $t = (a \delta)^{-1} (\delta x_n + |\tilde{x}|)$ (which is in $[0, t_0]$ if $|x|$ is small enough) and we get, after simplification, $$\operatorname{dist}(x, \partial \Omega) \geqslant r + \frac{x_n - \delta |\tilde{x}|}{a} $$ (the right-hand side is the distance to the boundary of $C_a$). Taking (1) into account, in both cases we get $$|\operatorname{dist}(x, \partial \Omega) - r - x_n| \leqslant \max\left\{a - 1, \frac{1}{a} - 1 + \frac{\delta}{a}\right\} |x| . $$ It follows that $$\limsup_{x \to 0} \frac{|\operatorname{dist}(x, \partial \Omega) - r - x_n|}{|x|} \leqslant \max\left\{a - 1, \frac{1}{a} - 1 + \frac{\delta}{a}\right\} . $$ Since $a > 1$ can be arbitrarily close to $1$, we get $$\lim_{x \to 0} \frac{|\operatorname{dist}(x, \partial \Omega) - r - x_n|}{|x|} = 0 , $$ which means that the gradient of $\operatorname{dist}(x, \partial \Omega)$ exists at $x = 0$ and it is equal to $(0, \ldots, 0, 1)$.

It remains to show that $\Omega$ contains $B_t$ for $t \geqslant 0$ sufficiently small. Note that $B_t$ is a ball tangent to the cone $C_a$, and the convex hull of $B_{t_1} \cup B_{t_2}$ contains $B_t$ for all $t \in [t_1, t_2]$. By assumption, $\Omega$ contains $B_0$, and so if $\Omega$ contains $B_{t_0}$ for some $t_0 > 0$, then it also contains $B_t$ for all $t \in [0, t_0]$. Therefore, it is sufficient to show that $\Omega$ contains $B_t$ for some $t > 0$. Suppose, contrary to this claim, that for each $t > 0$ there is a point $y_t \in B_t \setminus \Omega$. Choose any partial limit $y$ of $y_t$ as $t \to 0$. Since $y_t \notin \Omega$, we have $y \notin \Omega$. Similarly, $y_t \in \overline{B}_t$, and so $y \in \overline{B}_0$. Thus, $y \in \Omega^c \cap \overline{B}_0 = \{z\}$, that is, $y = z = (\tilde{0}, -r)$. On the other hand, $y_t \in B_t \setminus B_0$, and so the $n$-th coordinates of $y_t$ and $y$ are not less than $-a^{-1} \delta r$. But this is a contradiction, because $-r < -a^{-1} \delta r$. The proof is complete.

Claim 2: For almost every $x_0 \in \Omega$ the maximal ball $B(x_0, r)$ contained in $\Omega$ touches the boundary of $\Omega$ at a single point.

A a Lipschitz function, $f(x) = \operatorname{dist}(x, \partial \Omega)$ is differentiable almost everywhere. However, if $\partial B(x_0, r)$ has at least two points $z_1$, $z_2$ in common with $\partial \Omega$, then $f$ is not differentiable at $x_0$: by the proof of Claim 1, $\nabla f(x)$ is equal to $v_1 = (x_0 - z_1) / |x_0 - z_1|$ on $(x_0, z_1)$, and to $v_2 = (x_0 - z_2) / |x_0 - z_2|$ on $(x_0, z_2)$. Thus, if $\nabla f(x_0)$ existed, it would be equal to $v_1$ and to $v_2$ at the same time, which is impossible, because $v_1 \ne v_2$. This proves that $\nabla f(x_0)$ does not exist, and so Claim 2 follows.

---

By the way, is there an elementary proof of Claim 2, which does not rely on a.e. differentiability of Lipschitz functions?

Answer 4

Here is another answer to the convexity (or more precisely concavity) part of the question, which I think is even more simple than the one Anton gave:

Let $p\in\Omega$, and $q$ be one of its closest points on $\partial\Omega$. Since $\Omega$ is convex, there exists a support hyperplane $H$ of $\Omega$ which passes through $q$. Furthermore $H$ is orthogonal to $pq$, since it supports the sphere $S_p$ centered at $p$ and passing through $q$. So $d_{\partial\Omega}(p)=d_H(p)$. Clearly $d_H(p)$ is not bigger than $d_{H'}(p)$ for any other support plane $H'$ of $\Omega$, because all these planes lie outside $S_p$. So $d_{\partial\Omega}$ is the infimum of the distance functions to support hyperplanes of $\Omega$. These functions are linear and therefore concave. So $d_{\partial\Omega}$ is concave.

Answer 5

This is not an answer to the original question, but an alternative elementary proof of Claim 1 in the answer by Mateusz Kwaśnicki. Note that it works for any open set $\Omega$.

Let $\Omega$ be an open set in $\mathbb{R}^n$. Define $d$ by $d(x)=d(x, \mathbb{R}^n\backslash\Omega)$.

Assume that $0\in \Omega$ and $z$ is the only element of $\overline{B}(0,\|z\|)\backslash\Omega$. Our goal is to show that $\nabla d (0)= -\frac{z}{\|z\|}$, i.e. $$\lim\limits_{x\to 0}\frac{d(x)-\|z\|+\left<x,\frac{z}{\|z\|}\right>}{\|x\|}=\lim\limits_{x\to 0}\left(\frac{d(x)-\|z\|}{\|x\|}+\cos\beta\right)=0,$$ where $\beta$ is the angle between $x$ and $z$.

Step 1. Let $f(x)=\|x-z\|$ and note that $d(x)\le f(x)$ and $\nabla f (0)= -\frac{z}{\|z\|}$. Hence, we only need to estimate the behaviour of $\frac{d(x)-\|z\|}{\|x\|}+\cos\beta$ from below. Also, $d(x)\ge \|z\|-\|x\|$, for every $x\in\overline{B}(0,\|z\|)$, and so $\frac{d(x)-\|z\|}{\|x\|}+\cos\beta\ge \cos\beta-1$.

Fix $\varepsilon>0$. It is clear that $\frac{d(x)-\|z\|}{\|x\|}+\cos\beta>-\varepsilon$ if $\beta<\varepsilon$. We will show that if $\beta\ge\varepsilon$ then for sufficiently small $\|x\|$ we will have $\frac{d(x)-\|z\|}{\|x\|}+\cos\beta>-2\varepsilon$.

Step 2. For every point $y$ on the sphere $\partial B(0,\|z\|)$, other than $z$ there is $\delta>0$ such that $B(y,\delta)\subset\Omega$. For $\theta\in[0,\pi]$ consider all points on this sphere whose angle with $z$ is at least $\varepsilon$. This is a compact set, and so there is $\delta>0$ such that $\delta$-thickening of this set (see the blue contour on picture 1) is contained in $\Omega$.

Then for every $x$ in the little blue "packman" (whose radius is $\frac{\delta}{2})$) in the centre, $d(x)$ is greater or equal to the distance from $x$ to the part of the sphere $\partial B(0,\|z\|)$, painted with the thick black. Indeed, if $w$ is a closest point in $\mathbb{R}^n\backslash\Omega$ to $x$, then the segment $[x,w]$ has to cross either the thick black part of the $\partial B(0,\|z\|)$, or the outer boundary of the picture, which should give more than $1+\frac{\delta}{2}$ (I don't know how to explain this rigorously, but it seems clear from the picture), while $\|x-z\|\le 1+\frac{\delta}{2}$.

Step 3. For any circle in $\partial B(0,\|z\|)$ and any $x$, the closest to $x$ point $w$ on the circle is the intersection of the circle with the half-space obtained from the axis of the circle and $x$ (see picture 2). Moreover if the angles between $z$ and $w$ and $z$ and $x$ are $\alpha$ and $\beta$, then $\|x-w\|^2=\|x\|^2+\|z\|^2-2\|x\|\|z\|\cos (\beta-\alpha)$, which is minimized when $\alpha$ is maximal, i.e. $\alpha=\varepsilon$. Since $d(x)\ge \|x-w\|$, using conjugates we get $$\frac{d(x)-\|z\|}{\|x\|}+\cos\beta\ge \frac{\|x\|-2\|z\|\cos (\beta-\varepsilon)}{\|z\|+\sqrt{\|x\|^2+\|z\|^2-2\|x\|\|z\|\cos (\beta-\varepsilon)}}+\cos\beta.$$ Now if $\|x\|$ is sufficiently small we have $\frac{d(x)-\|z\|}{\|x\|}+\cos\beta\ge -\cos (\beta-\theta)-\varepsilon+\cos\beta\ge -2\varepsilon.$