1
$\begingroup$

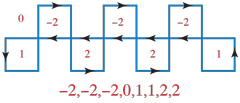
This is something of a followup to an earlier question Can any sequence of consecutive integers be realized as winding numbers?, whose answer is Yes.

Now I would like to define a local winding number for a plane curve as follows. For any region $R$ delimited by arcs of a plane curve $C$, define the local winding number as the number of counterclockwise arcs bounding $R$ minus the number of clockwise arcs bounding $R$. It might be easiest to assume that two arcs that meet cross there, so tangencies are excluded.

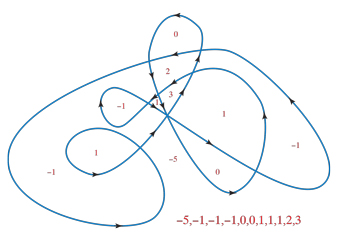
          LocalWindingNumber
As @ZachTeitler observed in a preliminary (now deleted) version of the question, "the sum of all the local winding numbers [must be] $0$, because each arc counts $+1$ on one side and $-1$ on the other." Indeed in the example above, the sum is zero.

My question is:

Q. Are there any other constraints to the sequence of local winding numbers defined by a plane curve?

I've explored a bit without seeing clear patterns, except for classes of regimented curves, e.g.:

          Local888

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Without allowing the curve to retrace itself, I doubt you could ever get $-2,2$. With only one curve, I doubt you could ever get $-1,-1,2$ (though it's easy enough with two curves). $\endgroup$
    – Will Brian
    Commented Jan 3, 2018 at 21:58
  • $\begingroup$ I think that more regularity conditions need to be introduced to rule out degenerate cases; my suggestion would be to require that in a periodic parametric equation of the curve there should be a bijection between $t$ and $(x(t),y(t),x'(t),y'(t))$ because otherwise qualitatively new behavior can be encountered e.g. a set of circles with a common point and at least one of them on each side of the common tangent - then one could trace out the circles in any randomly assignable set of direction, which will have interesting consequences for the local winding numbers. $\endgroup$
    – Manfred Weis
    Commented Jan 4, 2018 at 11:28

0

Reset to default

You must log in to answer this question.