4
$\begingroup$

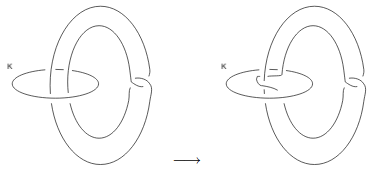
Take any link $L_1$ with an unknotted component $K$, cut along the disk bounded by K (which usually intersects some of the other components of $L_1$ transversally ), twist n times, and reglue. Let us denote by $L_2$ the resulting link.

It is easy to see that this operation of twisting may be described diagrammatically in the following manner : take a diagram $D_1$ of $L_1$, where the unknoted circle representing $K$ in $D_1$ is denoted $D_K$, take a disk which do not intersect $D_K$ and which contains the strands intersecting $D_K$ in $m$ points and replace that with a disk representing the diagram of an $m$-integer tangle which consists of $n$ twists to get a new diagram $D_2$.

enter image description here

My question is the following : if we start with two different diagrams $D_1$ and $D'_1$ of $L_1$ where $D_K$ and $D'_K$ are unknoted circles and perform the diagramatic twisting to the two diagrams to get $D_2$ and $D'_2$. Are these resulting diagrams equivalent (do they represent the same link $L_2$) ?

The question may be also put as : twisting two equivalent links along the same trivial component does it produce two equivalent links ?

Improve this question
$\endgroup$
1
  • $\begingroup$ Maybe you want to add the condition something like $\partial D_{K}$ and $\partial D_{K}'$ are in the same class in the fundamental group of the link complement. Otherwise it is clearly false, take one Disk that does not change the link (say it only intersects $L_{1}$ in one point), and one where the operation is non-trivial, such as your example above. $\endgroup$
    – Nick L
    Commented Oct 26, 2017 at 20:48

1 Answer 1

Reset to default
2
$\begingroup$

The result does not depend on the diagram, because the twisting operation does not depend on the disc $D$ with $\partial D = K$ that you choose. You can see this $n$-th twist as a self-diffeomorphism $\varphi$ of the solid torus $S^3 \setminus K$. If you pick two distinct discs $D, D'$, these are isotopic in this solid torus and hence the two self-diffeomorphisms $\varphi, \varphi'$ that they produce are isotopic. Therefore there is an isotopy relating the two links $\varphi(L\setminus K)$ and $\varphi'(L\setminus K)$ in the solid torus $S^3 \setminus K$, which extend to an isotopy of the whole twisted links in $S^3$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thaks @BrunoMartelli. I'm wondering if the existence of such an isotopy means that if we twist two equivalent links in $S^3$ along two disks which meet the links in the same number of points at theire interiors yields isotopic links. $\endgroup$
    – Mohammed Sabak
    Commented Nov 5, 2017 at 17:15

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .