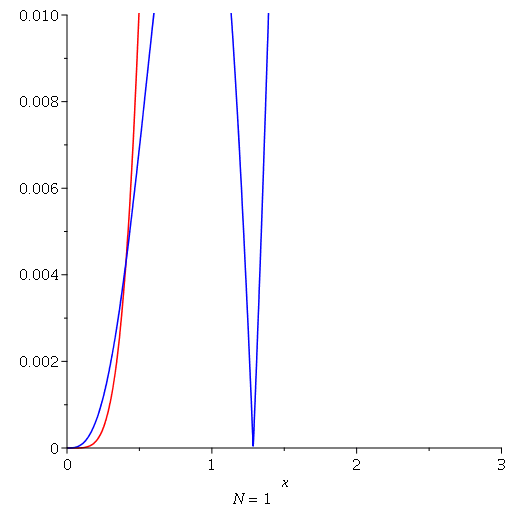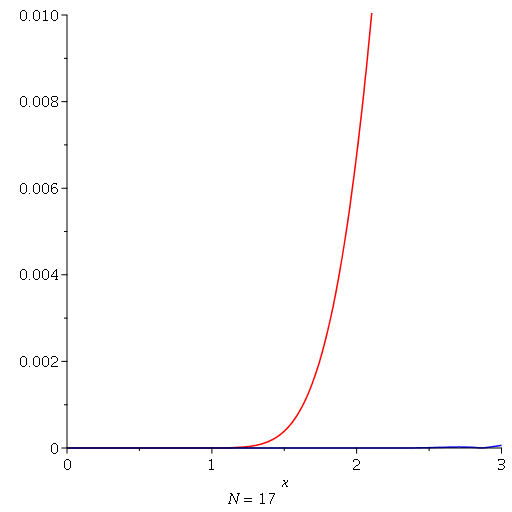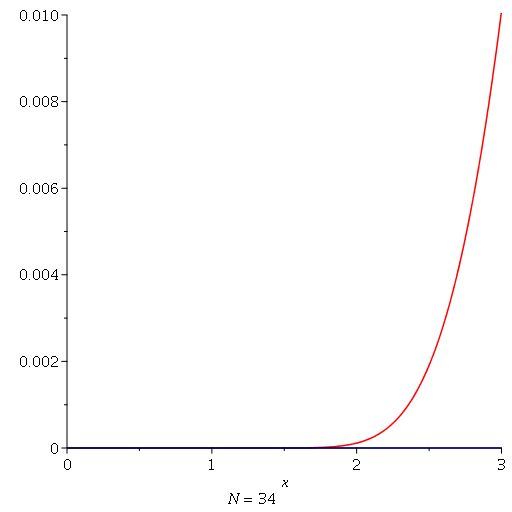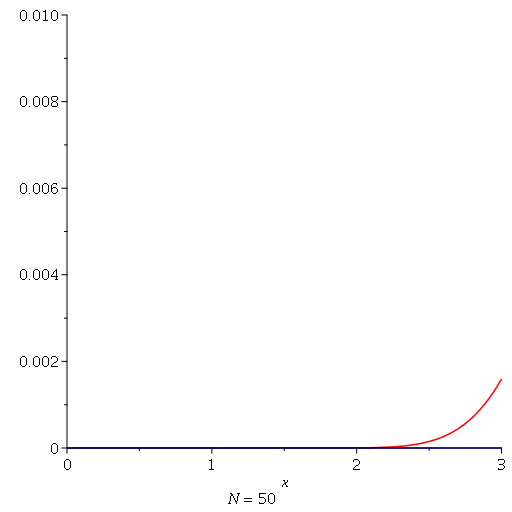
I want to compare two equations for the arctangent function: $$\tag{1}\arctan\left(x\right)=\sum\limits_{n=0}^{\infty}{\frac{{2^{2n}}{\left(n! \right)}^{2}}{\left(2n+1\right)!}\frac{x^{2n+1}}{{\left(1+x^2\right)}^{n+1}}}$$ and $$\tag{2}\arctan\left(x\right)=i\sum\limits_{n=1}^{\infty}{\frac{1}{2n-1}}\left(\frac{1}{\left(1+2i/x\right)^{2n-1}}-\frac{1}{\left(1-2i/x\right)^{2n-1}}\right).$$
Which of them is faster in convergence?