Convergence of Newton series for sin ax

Question

Let's define half discrete-analytic function as a function whose Newton series converges to that function for each $x>0$:

$$f(x)=\sum_{k=0}^\infty \binom{x}k \Delta^k f\left (0\right)=\sum_{m=0}^{\infty} \binom {x}m \sum_{k=0}^m\binom mk(-1)^{m-k}f(k)=\lim_{n\to\infty}\frac{\sum_{k=0}^{n} \frac{(-1)^k f(k)}{(x-k)k!(n-k)!}}{\sum_{k=0}^{n} \frac{(-1)^k }{(x-k) k!(n-k)!}}$$

Let's define weak discrete-analytic a function a function whose bi-directional Newton expansion converges to that function at any real $x$:

$$f(x)=\lim_{n\to\infty}\frac{\sum _{k=-n}^n \frac{(-1)^k f(k)}{(x-k) (k+n)! (n-k)!}}{\sum _{k=-n}^n \frac{(-1)^k}{(x-k) (k+n)! (n-k)!}}$$

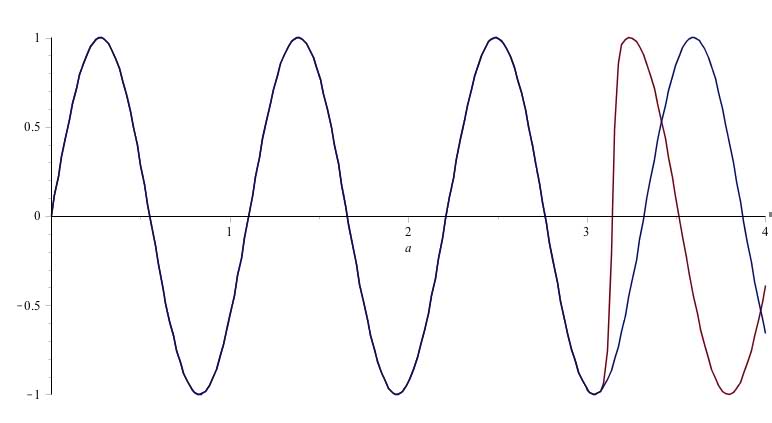
It seems that the function $\sin x$ is both half discrete-analytic and weak discrete-analytic. But the function $\sin \frac{\pi x}2$ is only weak discrete-analytic.

So what is the maximum $a$ such that $\sin ax$ is half discrete-analytic?

I also interested to know at which $a$ $\sin ax$ is weak discrete-analytic. For example, is $\sin 3x$ weak discrete-analytic or not?

This question is motivated by my old search for a natural fractional integration and integration constant (integral analog of Ramanjuan sum). I want to find a natural generalization of Newton series to functions whose Newton series normally diverges. Once that accomplished it would be possible to find Newton expansions for consecutive derivatives of a function and by analytically continuing them into negative domain, get natural integral. Unfortunately finding natural fractional integral of even such simple function as $\sin x$ requires building Newton expansion for $\sin \frac{\pi x}2$ which diverges.

Answer 1

half-discrete analytic

First do the formal calculation, then discuss its validity. $$\begin{align} &\sum_{m = 0}^{\infty} \binom{x}{m} \sum_{k = 0}^{m} \binom{m}{k} (-1)^{(m - k)} \operatorname{sin} (a k) \cr &=\sum_{m = 0}^{\infty} \frac{-i}{2}\binom{x}{m} \biggl(\bigl(\operatorname{e} ^{i a} - 1\bigr)^{m} - (-1)^{m} \Bigl(\operatorname{e} ^{(-ia)}-1\Bigr)^{m}\biggr) \cr &=-\frac{i}{2}\biggl(\sum_{m = 0}^{\infty}\binom{x}{m} \bigl(\operatorname{e} ^{i a} - 1\bigr)^{m} - \sum_{m = 0}^{\infty} \binom{x}{m} \Bigl(\operatorname{e} ^{(-ia)}-1\Bigr)^{m}\biggr) \cr &= -\frac{i}{2}\Biggl(\left(\operatorname{e}^{ia}\right)^x - \left(\operatorname{e}^{-ia}\right)^x\Biggr) \cr &= -\frac{i}{2}\left(\operatorname{e}^{iax} - \operatorname{e}^{-iax}\right) = \sin(ax) \end{align}$$ There are two places where the computation may be invalid. First, convergence of the binomial series, we require $$ \left|\operatorname{e}^{ia}-1\right| < 1, \qquad \left|\operatorname{e}^{-ia}-1\right| < 1 $$ Second, we need to do this: $$ \left(\operatorname{e}^{ia}\right)^x = \operatorname{e}^{iax}, $$ where that $x$ exponent is the sum from the binomial series.

Both of these will be OK for $-\pi/3 < a < \pi/3$.

Answer 2

weak discrete-analytic

Closed form here involves some ${}_2F_1$ functions, so I cannot provide proofs. Numerically, though, it seems that $\sin(ax)$ is weak discrete-analytic for $a$ up to some value just above $3$. Maybe $\pi$, I guess.

In this diagram we have $\sin(ax)$ in blue (for $x=5.678$); and 5000 terms for the Newton series in red. They agree for $a$ up to about $3.1$ or $3.2$...