Please give references for the integral transform of the next kind: $$ F_3(f(x))(t)=\int_{-\infty}^{\infty} \exp(Q_3(x,t)) f(t)\,dt , $$ with $Q_3(x,t)$ - a cubic polynomial of its arguments. Special cases are interesting, and of course a general case. It seems such transforms are used in PDO theory, not so?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ Have you tried Google search? $\endgroup$– user64494Commented Apr 20, 2017 at 7:57
-
$\begingroup$ The key word is Airy function. What exactly do you want to know? $\endgroup$– Alexandre EremenkoCommented Apr 20, 2017 at 16:03
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
7
Do you mean the Airy transform?
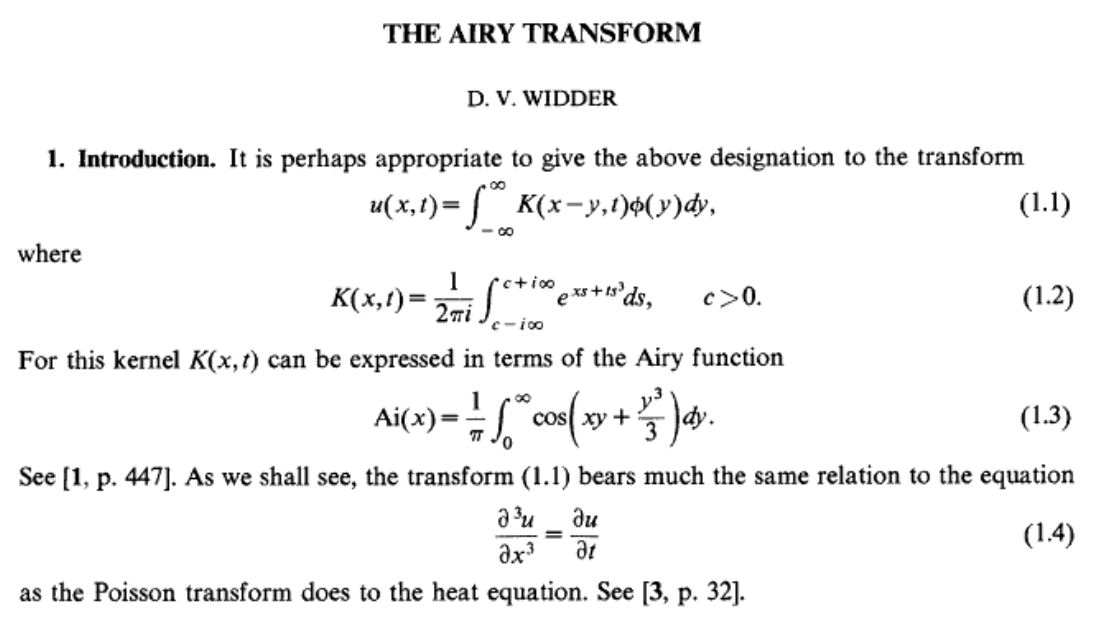
A more recent reference is The Airy transform and the associated polynomials (2010).
In your notation the function $F_3$ is the Airy transform of the Fourier transform $\hat{f}$ of $f$:
$$F_3(x)=\int_{-\infty}^\infty dt\,\exp\left(\tfrac{1}{3}it^3+itx\right)f(t)$$ $$\qquad=\frac{1}{2\pi}\int_{-\infty}^\infty d\xi\,\int_{-\infty}^\infty dt\exp\left(\tfrac{1}{3}it^3-it(\xi-x)\right)\hat{f}(\xi)$$ $$\qquad=\int_{-\infty}^\infty d\xi\, {\rm Ai}(\xi-x)\hat{f}(\xi).$$
answered Apr 20, 2017 at 8:28
-
$\begingroup$ Could you base your words "In your notation the function $F_3$ is the Airy transform of the Fourier transform of $f$"? @Carlo Beenakker $\endgroup$ Commented Apr 20, 2017 at 9:14
-
$\begingroup$ @user64494 --- I've added the requested steps. $\endgroup$ Commented Apr 20, 2017 at 9:57
-
$\begingroup$ @ Carlo Beenakkeer: Sorry, but this is not it bacause $Ai(\xi−x)$ does not have a form $\exp(Q_3(x,t))$. $\endgroup$ Commented Apr 20, 2017 at 10:01
-
$\begingroup$ you should look at the first line: $Q_3(x,t)=\frac{1}{3}it^3+itx$ $\endgroup$ Commented Apr 20, 2017 at 10:02
-
$\begingroup$ Sorry, I see $\tfrac{1}{3}it^3-it(\xi-x)$ . @Carlo Beenakker $\endgroup$ Commented Apr 20, 2017 at 10:04