29
$\begingroup$

In general, we might ask when we can find interesting spaces $X, Y$ such that $X$ is homeomorphic to $[X, Y]$. By the Lawvere fixed point theorem $Y$ must have the fixed point property. Happily, $Y = [0, 1]$ does in fact have the fixed point property (e.g. by the intermediate value theorem), so this is not an obstruction.

I know that computer scientists have constructed spaces $X$ such that $X$ is homeomorphic to $X^X$ in domain theory, but I don't know enough about it to tell whether the techniques are relevant here.

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Functions = continuous functions, I assume? $\endgroup$
    – Igor Rivin
    Commented Mar 17, 2017 at 2:06
  • $\begingroup$ Yes, I suppose that's worth clarifying. Edited. $\endgroup$
    – Qiaochu Yuan
    Commented Mar 17, 2017 at 2:36
  • 1
    $\begingroup$ I guess you are considering the compact open topology on $[X,Y]$? Or is the question is also about finding the topology on $[X,Y]$? $\endgroup$
    – Cusp
    Commented Mar 17, 2017 at 5:20
  • 3
    $\begingroup$ There are two candidates one might consider as a first approximation: the direct limit of $C^k(I)\to C^{k+2}(I)\to C^{k+4}(I)\to...$ or the inverse limit of $...\to C^{k+4}(I)\to C^{k+2}(I)\to C^k(I)$ (the latter is $C(\_)$ of the former) $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Mar 17, 2017 at 5:52
  • 3
    $\begingroup$ I'd pause before decreeing it should be the compact-open topology; I'd say it should be the exponential topology first and foremost, i.e., the topological structure such that there is a natural isomorphism $Top(-, [X, Y]) \cong Top(- \times X, Y)$. This need not be the compact-open topology (although it will be if $X$ is say locally compact Hausdorff). $\endgroup$
    – Todd Trimble
    Commented Mar 17, 2017 at 12:18

1 Answer 1

Reset to default
33
$\begingroup$

There cannot be such a homeomorphism, because Lawvere's fixed point theorem would give us something too constructive: a continuous map $[0,1]^{[0,1]} \to [0,1]$ that assigns a fixed point to each continuous map $[0,1] \to [0,1]$.

Following the proof of the Lawvere fixed point theorem, given a homeomorphism $f: X \cong [0,1]^X$ and a map $g: [0,1] \to [0,1]$, we construct a map $h: X \to [0,1]$ by $h(x) = g(f(x)(x))$, and then we get an element $x^* = f^{-1}(h)$ of $X$. Then,

$$ f(x^*)(x^*) = h(x^*) = g(f(x^*)(x^*)), $$

so we have found a fixed point of $g$. This construction is continuous in $g$, since we're working with the exponential topology on $[0,1]^{[0,1]}$, so $g \mapsto f(x^*)(x^*)$ gives a continuous fixed-point-finding map $[0,1]^{[0,1]} \to [0,1]$.

There is a lot of work on the nonconstructivity of Brouwer's fixed point theorem for different senses of constructivity. To give a quick visual proof that no continuous map of the type considered here exists, it is sufficient to consider a path through $[0,1]^{[0,1]}$ along which such a map cannot be continuously defined. Consider linearly deforming green to red to purple in the below diagram; a fixed point (i.e. a point where the curve crosses the identity depicted in blue) cannot be continuously chosen.

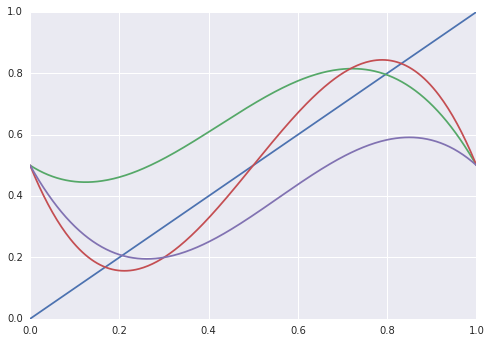
Three curves showing Brouwer is nonconstructive

It's interesting that this works because we have a homeomorphism $X \cong [0,1]^X$ rather than just a continuous surjection. A continuous surjection $X \to [0,1]^X$ would be enough to deduce the intermediate value theorem using Lawvere's fixed point theorem, but not in a constructive enough way to similarly rule out.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Aha, nice construction! $\endgroup$
    – Qiaochu Yuan
    Commented Mar 22, 2017 at 8:08
  • 4
    $\begingroup$ In fact for this argument to work, we don't need that $[0, 1]^X$ is homeomorphic to $X$, just that it is a retract of $X$. This observation is put to use in an answer on the related thread, here: mathoverflow.net/a/267603/2926 $\endgroup$
    – Todd Trimble
    Commented Apr 19, 2017 at 12:07

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .