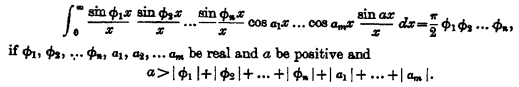7
$\begingroup$

Let $a_1,\dots,a_n, b$ be positive real numbers.

*Question.** Is this true? $$\int_{-\infty}^{\infty}\frac{\sin(bx+a_1x+\cdots+a_nx)}{x}\prod_{j=1}^n\frac{\sin(a_jx)}{a_jx}\,\,dx=\pi.$$ My most immediate quest is "why is it independent of $b$, in particular?"

Improve this question
$\endgroup$
5
  • $\begingroup$ I cannot immediately see the independence of the "b", but I am aware of an apparently not-so-widely-known fact, that "sinc"'s convolved with each other behave remarkably. Namely, up to constants and normalizations, ${\sin x\over x}$ is the Fourier transform of the char fcn of an interval symmetrical about the origin. Thus, the convolution of several sinc's (with constants inserted) is the Fourier transform of the product of several char fcns of symmetrical intervals, which is just the FT of the char fcn of the smallest among them. If this is in the right direction for you, I could elaborate. $\endgroup$
    – paul garrett
    Commented Mar 1, 2017 at 23:11
  • 1
    $\begingroup$ This fact about convolutions of sincs is, I would say, widely known. Certainly it is at least to harmonic analysts and anyone doing signal processing. This is also how you define B-splines. $\endgroup$
    – Keaton Hamm
    Commented Mar 1, 2017 at 23:17
  • 1
    $\begingroup$ @KeatonHamm, ah, doesn't surprise me that signal-processing people would know this, but/and I didn't know how lively and considered-mathematics such a subject was/is! My comment is based on the palpable fact that such useful and poignant facts haven't made it into many standard "real analysis" texts, so the vast majority of grad students, hence, mathematicians, have no idea that such a thing is/could-be true. I used to live in that land myself! :) $\endgroup$
    – paul garrett
    Commented Mar 1, 2017 at 23:29
  • $\begingroup$ Paul and Keaton:Thank you both for the added discussion. The Fourier transform of $sinc(x)$ gives a square signal. If you take increasing powers $sinc^n(x)$ and compute its Fourier, the resulting signal gets smoother and smoother and eventual yielding a normal distribution curve. $\endgroup$
    – T. Amdeberhan
    Commented Mar 1, 2017 at 23:50
  • 2
    $\begingroup$ This result and a more general one is due to Stoermer, see Whittaker and Watson, Modern Analysis, chapter 6, example 6 at page 122. $\endgroup$
    – Nemo
    Commented Mar 2, 2017 at 7:00

2 Answers 2

Reset to default
10
$\begingroup$

Let $s(x) = \frac{\sin(\pi x)}{\pi x}$ be the normalized sinc function, $a_j' = \frac{a_j}{\pi}$ and $c = \frac{b+\sum_j a_j}{\pi}$. Then you want to compute $\pi\int_{-\infty}^\infty c s(cx)\prod_j s(a_j' x)dx$. This is equal to the value of the Fourier transform of the integrand at zero. The Fourier transform of the integrand is \[ r\left(\frac{f}{c}\right) \ast \left[\pi\cdot\frac{1}{a_1'}r\left(\frac{f}{a_1'}\right) \ast \cdots \ast \frac{1}{a_n'}r\left(\frac{f}{a_n'}\right)\right], \] where $r$ denotes the indicator function of the interval $[-1,1]$.

Note that the bracketed term has compact support contained in the interval $\left[-\sum_j a_j', \sum_j a_j'\right]$, so in particular contained in $[-c,c]$ regardless of $b$. So the value at zero of this outermost convolution is just the integral of the bracketed term. We can compute this integral by Fourier transforming again and evaluating the result at zero. But this is just $\pi\cdot\prod_j s(a_j' \cdot 0) = \pi$.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ This is cool. I took the liberty to insert the missing $\pi$, so now all is well. $\endgroup$
    – T. Amdeberhan
    Commented Mar 2, 2017 at 0:00
11
$\begingroup$

A more general result is due to C. Störmer (Acta Mathematica December 1895, Volume 19, Issue 1, pp 341–350)

enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .