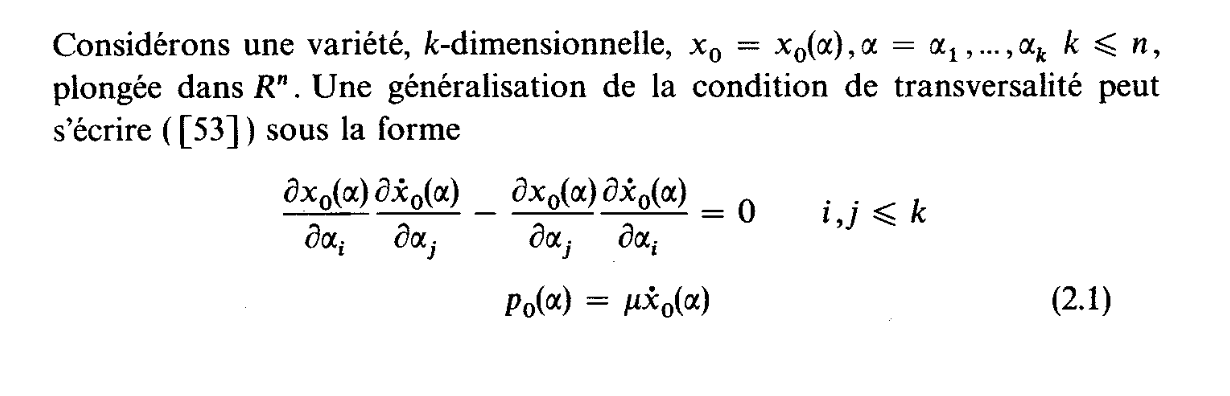
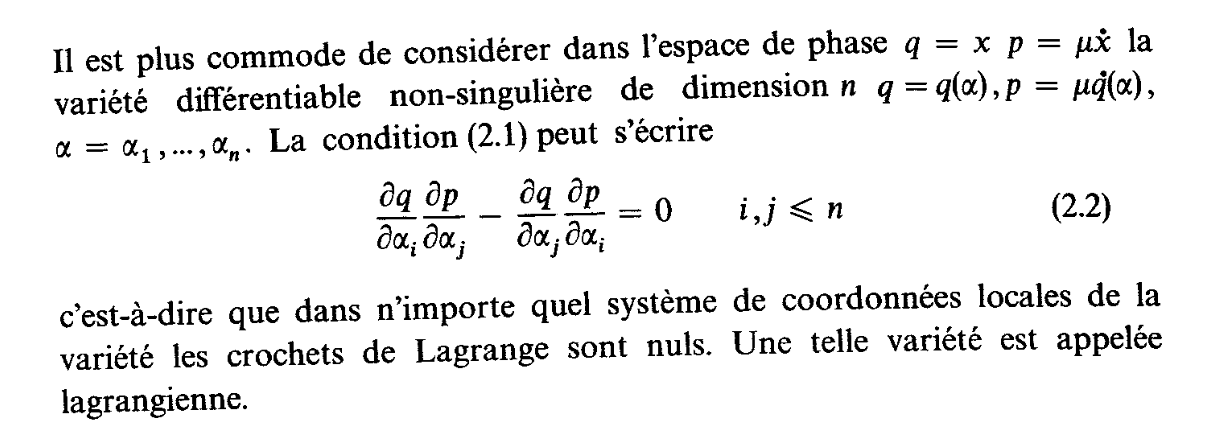
Much of the terminology in symplectic geometry comes from classical mechanics: the symplectic manifold is modeled on a cotangent bundle $T^*N$ of some configuration space $N$ with local position coordinates $q_i$ and momentum coordinates $p_i$ such that $\omega = \sum_i dq_i \wedge dp_i$. Then, a Hamiltonian - a.k.a. a smooth "energy" function - on this phase space induces a flow satisfying Hamilton's equations from classical mechanics: $$\frac{dp_i}{dt} = -\frac{\partial H}{\partial q_i} \hskip{1 in} \frac{dq_i}{dt} = \frac{\partial H}{\partial p_i}$$ In this physical interpretation, what are Lagrangian submanifolds? In particular, why are they named Lagrangian? Is there a relationship between this notion and the Lagrangian formulation of mechanics?
(Note: this question has a lot of great answers providing some physical or geoemtric intuition for Lagrangian submanifolds - in a cotangent bundle, both fibers and the images of closed sections (closed $1$-forms on $N$) are motivating examples of Lagrangian submanifolds - but it does not address the source of the terminology.)