This echos the 2017 comments, but since the question has now been bumped to the front page it might be helpful to give the actual source in Maslov's book [1].
[1] V.P. Maslov, Perturbation Theory and Asymptotic Methods (in Russian, Moscow, 1965); Théorie des pertubations et méthodes asymptotique (French translation, Paris, 1972).
The Lagrangian manifold (variété lagrangienne) is introduced on page 114-115:
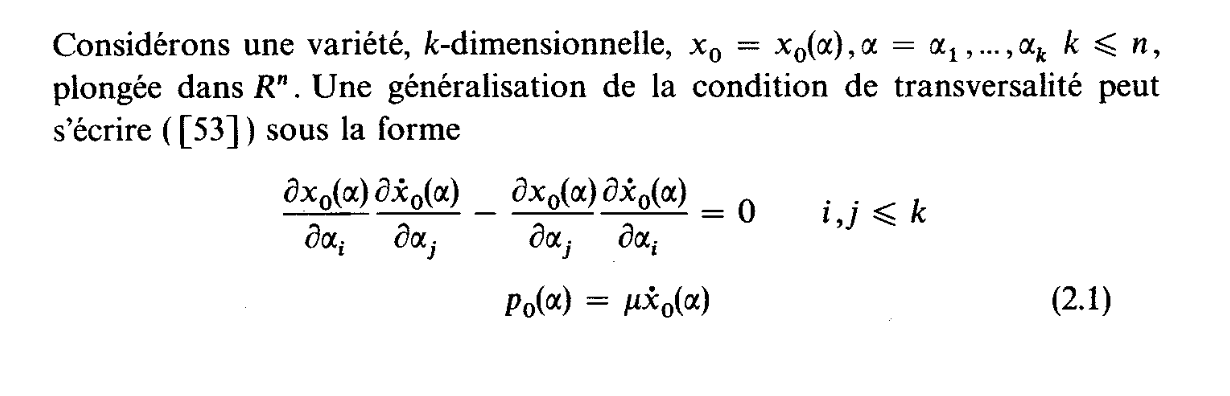
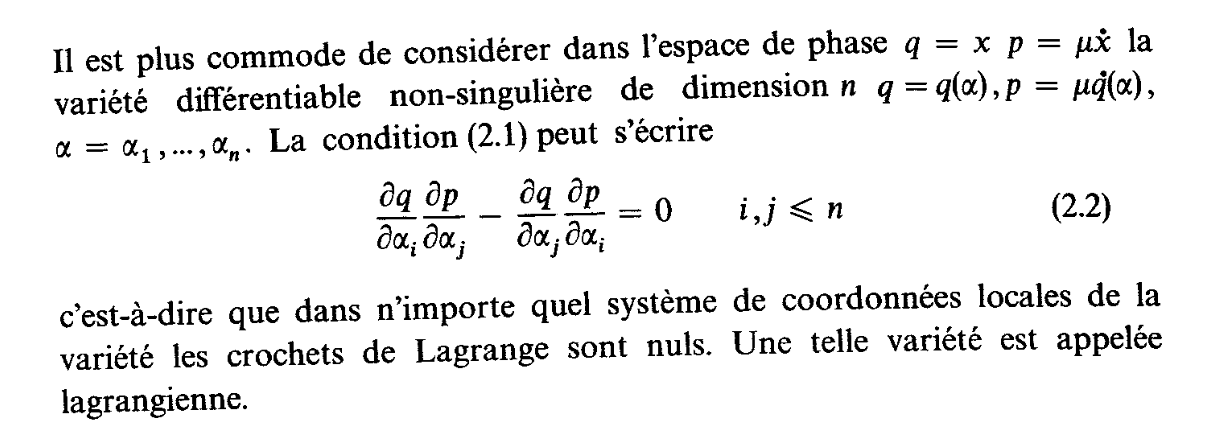
The Lagrangian submanifold (sous-variété lagrangienne) follows on page 147

