Appropriate morphisms and 2-morphisms in Ind(C)

Question

As I was trying to understand the category $Ind(C)$ of diagrams of the form $I \to C$, where $I$ is a small filtered $(0,1)$-category, I wondered whether it is possible to define morphisms directly, without the limit-colimit construction (see https://ncatlab.org/nlab/show/ind-object), and then try to show that its equivalent to the common definition as $\text{lim$_i$ colim$_j$}\,C(F(i),G(j))$. A natural choice would be to define a morphism $(F:I\to C) \to (G:J \to C)$ as a pair $(f,g)$, where $f:I \to J$ is a functor in the $(1,2)$-category of all poset-categories, and $g:F \Rightarrow G \circ f$ is a natural transformation. Then, the functor $C \hookrightarrow Ind(C)$ is clearly fully faithful. While trying to show that each object in $Ind(C)$ is a colimit of itself, I recognized that I should rather look at 2-colimits, but then I should define what a 2-morphism is. I tried several things but I'm not an expert for higher categories, so unfortunately I lack the necessary intuition... do you know a canonical choice for 2-morphisms in $Ind(C)$?

Answer 1

On a more general note, you're (gradually) building a subcategory of the 2-fiber $\textbf{Cat}/C$. An excellent reference for those is the following paper, which helped me a lot when I was looking into this stuff:

A Bullejos and M Cegarra, On the geometry of 2-categories and their classifying spaces, K-Theory 29 (2003) 211-229.

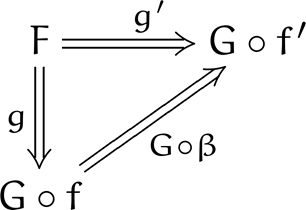
The journal where this paper appeared is now famously defunct, but you can find a pdf of the article here. The explicit answer to your question regarding 2-morphisms is as follows. Let $F$ and $G$ be as in your question and consider a pair of 1-morphisms $(f,g)$ and $(f',g')$, also as in your question. A 2-morphism $(f,g) \Rightarrow (f',g')$ is a natural transformation $\beta:f \Rightarrow f'$ (i.e., a 1-morphism in the category of functors from $I$ to $J$) so that the following holds: $$(G\circ\beta) * g = g'$$

Here $*$ stands for vertical composition while $\circ$ is horizontal composition. Pictorially, we have: