On a more general note, you're (gradually) building a subcategory of the 2-fiber $\textbf{Cat}/C$. An excellent reference for those is the following paper, which helped me a lot when I was looking into this stuff:
A Bullejos and M Cegarra, On the geometry of 2-categories and their classifying spaces, K-Theory 29 (2003) 211-229.
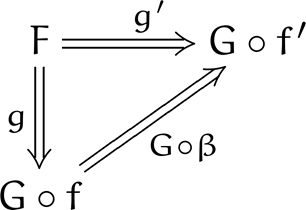
The journal where this paper appeared is now famously defunct, but you can find a pdf of the article here. The explicit answer to your question regarding 2-morphisms is as follows. Let $F$ and $G$ be as in your question and consider a pair of 1-morphisms $(f,g)$ and $(f',g')$, also as in your question. A 2-morphism $(f,g) \Rightarrow (f',g')$ is a natural transformation $\beta:f \Rightarrow f'$ (i.e., a 1-morphism in the category of functors from $I$ to $J$) so that the following holds: $$(G\circ\beta) * g = g'$$
Here $*$ stands for vertical composition while $\circ$ is horizontal composition. Pictorially, we have: