3
$\begingroup$

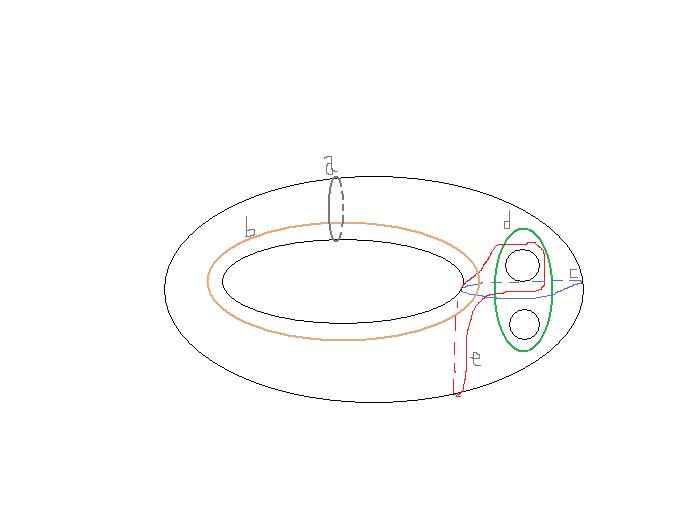
It is well-known that in the picture below we have $t_d=(t_at_b)^6$ as elements in the mapping class group of a two-holed torus, ($t_\gamma$ represents positive Dehn twist about the curve $\gamma$). Is it possible to get another factorization of $t_d$ in terms of the curves $a$,$b$,$c$ and $e$ below?

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Does "factorization" mean "expression for" or does it mean "root of"? $\endgroup$
    – Sam Nead
    Commented Feb 27, 2016 at 11:01

1 Answer 1

Reset to default
2
$\begingroup$

Let's define the mapping class group to be homeomorphisms of the surface, up to isotopy. Thus a Dehn twist about a boundary component is isotopic to the identity map. Now the twist about $d$ can be expressed in terms of those about $c$, $e$, and $a$, using the lantern relation.

Improve this answer
$\endgroup$
4
  • $\begingroup$ How does the boundary parallel dehn twists vanish in your setting? I meant a relation other than the one coming from lantern. for intance for $e$ we have:$e=b^{-1}a^{-2}cbc^{-1}a^2b$. I'm looking for sth similar for the curve $d$. $\endgroup$
    – nikita
    Commented Feb 27, 2016 at 14:35
  • $\begingroup$ 1) They are isotopic to the identity. 2) I don't see why you are ruling out the lantern relation. Perhaps you could add some details to your question, so I can understand what you are asking? 3) The curves $a$, $b$, $c$, and $e$ are all non-separating, so yes, the Dehn twists on those curves are conjugate to each other. The curve $d$ is separating, so it cannot be conjugate to any of the others. $\endgroup$
    – Sam Nead
    Commented Feb 27, 2016 at 15:30
  • $\begingroup$ this is the problem I am thinking about: the problem is comparing two contact structures on the Brieskorn manifold (2,3,11). One induced by the Milnor fiber and the other one by a plumbing tree. According to a result of Ghiginni-Shoenberger:arxiv.org/abs/math/0201099 the tight contact structures on brieskorn manifold (2,3,11) are realized by two possible Legendrian realizations of the -3-knot at the tail of the plumbing. I want to check that open book from the Milnor fiber with monodromy=(ab)^11 is equivalent to the open book obtained by "rolling up" the plumbing diagram. $\endgroup$
    – nikita
    Commented Feb 27, 2016 at 16:44
  • $\begingroup$ Here the rolling up method is explained: arxiv.org/abs/math/0607344. The problem is I could not get the from $\psi=(ab)^{11}=(ab)^5 d$ to the open book obtained by rolling up the diagram, which does not involve the curve $d$. $\endgroup$
    – nikita
    Commented Feb 27, 2016 at 16:52

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .