2
$\begingroup$

Definition. A magic tesseract is a four-dimensional array, equivalent to the magic cube and magic square of lower dimensions, containing the numbers 1, 2, 3, …, m^4 arranged in such a way that the sum of the numbers in each of the m^3 rows, m^3 columns, m^3 pillars, m^3 files and in the eight major quadragonals passing through the center and joining opposite corners is a constant sum S, called the magic sum, which is given by: S = m(m^4+1)/2 and where m is called the order of the tesseract.

I found a magic tesseract of order 3 of distinct positive integers. Now I want to find a magic tesseract of order 3 of distinct primes.

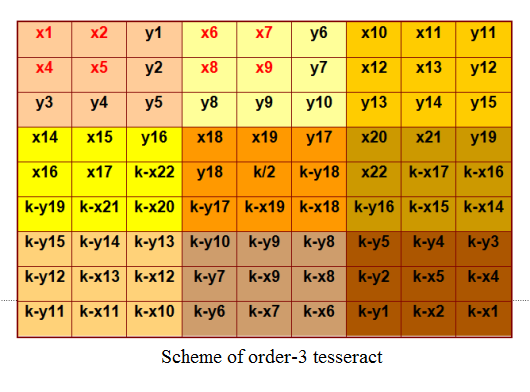
I got the general formula magic tesseract of order 3:

x10=s-x1-x6, x11=s-x2-x7, x12=s-x4-x8, x13=s-x5-x9, x14=(10*s)/3-2*x1-x2-x4-x5-x6-x7-x8-x9, x15=-((2*s)/3)+x5+x7+x9, x16=-((2*s)/3)+x5+x8+x9, x17=(4*s)/3-2*x5-x9, x18=-((2*s)/3)+x7+x8+x9, x19=(4*s)/3-2*x7-x9, x20=-((5*s)/3)+2*x1+x2+x4+x5+x6, x21=s/3-x5+x7, x22=s/3-x5+x8,

y1=s-x1-x2, y2=s-x4-x5, y3=s-x1-x4, y4=s-x2-x5, y5=-s+x1+x2+x4+x5, y6=s-x6-x7, y7=s-x8-x9, y8=s-x6-x8, y9=s-x7-x9, y10=-s+x6+x7+x8+x9, y11=s-x10-x11, y12=s-x12-x13, y13=s-x10-x12, y14=s-x11-x13, y15=-s+x10+x11+x12+x13, y16=s-x14-x15, y17=s-x18-x19, y18=4s/3-2*x18-x19, y19=s-20-x21,

The formula obtained for this scheme: enter image description here

s=3k/2

k/2 - prime number

Improve this question
$\endgroup$
1
  • $\begingroup$ I am afraid this is probably possible to solve with an exhaustive computer search. There are unlikely quick methods for solving systems of linear equations, where solutions are required to be distinct primes. $\endgroup$
    – Per Alexandersson
    Commented Dec 12, 2015 at 0:13

1 Answer 1

Reset to default
7
$\begingroup$

According to http://mathworld.wolfram.com/MagicTesseract.html, there exists a magic tesseract $T$ of order three with entries $1,2,\dots 81$. By the Green-Tao theorem on arbitrarily long arithmetic progressions of prime numbers, there exists an arithmetic progression $a+b,a+2b,\dots,a+81b$ of distinct prime numbers. Replace the number $k$ in $T$ with $a+kb$ to obtain a magic tesseract of order three of distinct primes.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Computer search reveals that there are no solutions with $a,b \leq 2000$. $\endgroup$
    – Per Alexandersson
    Commented Dec 12, 2015 at 1:28
  • $\begingroup$ I know this method. Let there be given an arithmetic progression an = 3n + 5 (n-1), n = 1,2, ... 81 Compose magic tesseract of order 3 of the numbers of the arithmetic progression: 333 38 238 143 388 78 133 183 293, 98 208 303 358 3 248 153 398 58, 178 363 68 108 218 283 323 28 258, 128 193 288 343 33 233 138 383 88, 163 393 53 93 203 313 353 13 243, 318 23 268 173 373 63 118 213 278, 148 378 83 123 188 298 338 43 228, 348 8 253 158 403 48 103 198 308, 113 223 273 328 18 263 168 368 73 I believe that a solution can be found without an arithmetic progression of 81 primes. $\endgroup$
    – Natalia Makarova
    Commented Dec 12, 2015 at 1:41
  • $\begingroup$ As far as I know, found an arithmetic progression of 26 primes. $\endgroup$
    – Natalia Makarova
    Commented Dec 12, 2015 at 1:49
  • $\begingroup$ Sorry. Clarification. Let there be given an arithmetic progression an = 3 + 5 (n-1), n = 1,2, ... 81. $\endgroup$
    – Natalia Makarova
    Commented Dec 12, 2015 at 1:55
  • 2
    $\begingroup$ @Per, in order for $a+b,a+2b,\dots,a+81b$ to be distinct prime numbers, $b$ has to be divisible by the product of all the primes less than 81. $\endgroup$
    – Gerry Myerson
    Commented Dec 12, 2015 at 3:57

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .