"Fractally self-similar" numbers

Question

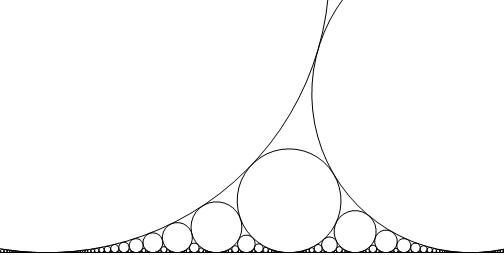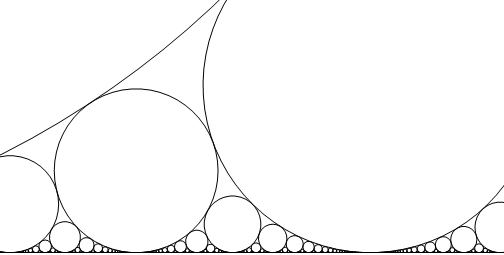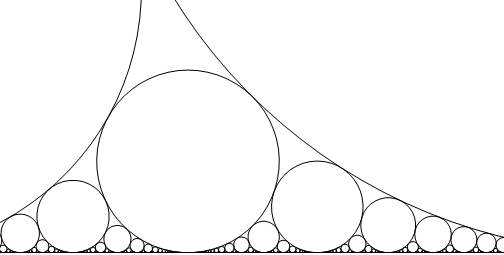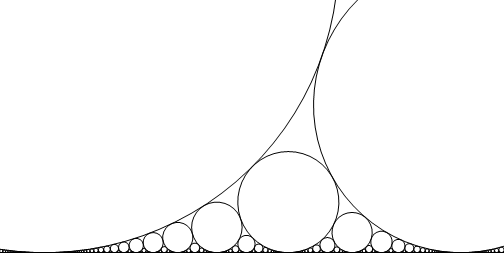
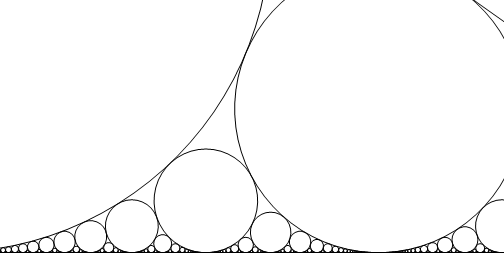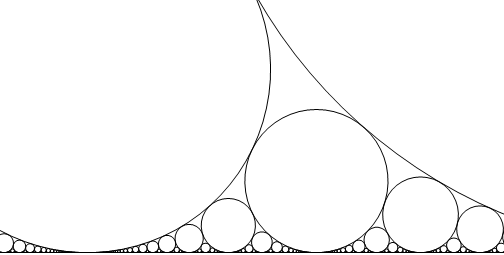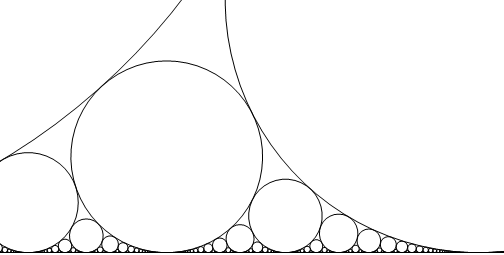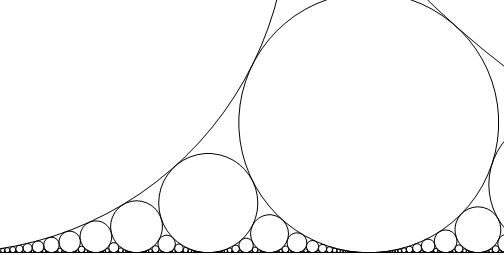
This is another question about visualization of Ford circles, the previous one being Confusion with practically implementing rational approximations. Here is an output of zooming into Ford circles at $\frac1{\sqrt2}$

Empirically I found that the pattern repeats periodically and arranged the number of frames so that one gets impression of a continuous infinite zoom (however if one looks very attentively there is a slight jump where the animation restarts).

What I want to know is whether the pattern indeed repeats rigorously for quadratic irrationalities, and whether for other irrational numbers the pattern occasionally becomes "almost" the same (clearly if the number is rational then the picture eventually starts degenerating into the horizontal line).

Since I do not actually know how exactly to formulate it, let me ask the question in form that practically occurred to me: for a real $x$ denote by $P_r(c)$ the picture of Ford circles in the rectangle $(x-c,x+c)\times(0,c)$ with resolution $r$. That is, features of size less than $rc$ cannot be observed; in particular, only the Ford circles of radius $>rc$ are visible, and moreover a circle cannot be distinguished from another one if they are both contained in an annulus of width $<rc$.

For which $x$ do there for any resolution $r$ exist two different $c$ and $c'$ such that the pictures $P_r(c)$ and $P_r(c')$ cannot be distinguished? (Well, they must be "sufficiently different" - say, there is still another $c<c''<c'$ such that $P_r(c'')$ can be distinguished from both.)

And the question about this question - what is (if any) a rigorous mathematical statement behind it?

(Later - decided to add the zoom for the golden ratio, here the jump is almost impossible to notice

Circles closest to the center alternate between left and right, ratios of their sizes must be something like consecutive Fibonacci numbers...

Answer 1

Before you can formulate your question precisely, you need a better notion of distance between two pictures of a set $S \subset \mathbb{C}$ (where in this case, $S$ is the union of Ford circles). If $\iota_1, \iota_2$ are pictures (bijections from square subsets $A_1, A_2$ of $\mathbb{C}$ to the unit square), then we can define distance as the Hausdorff distance between $\iota_1(A_1 \cap S)$ and $\iota_2(A_2 \cap S)$.

(With your original definition, we can trivially find two indistinguishable pictures by the pigeonhole principle, since there are only finitely many distinguishable pictures.)

---

The entire tessellation of Ford circles can be reconstructed by specifying any two tangent circles together with the horizontal line. Hence any Moebius transformation which maps a pair of tangent Ford circles to another such pair must extend to an automorphism of the entire tessellation. Since the Ford circles do not possess scale-invariance, this answers your first question (about whether the self-similarity is exact) negatively.

There is, on the other hand, a rigorous way in which we can say that your zoom sequence about a quadratic irrational is 'asymptotically' scale-invariant.

---

Specifically, the Ford circles (viewed as living in the upper half-plane) are invariant under the modular group $PSL(2, \mathbb{Z})$ consisting of all fractional linear transformations:

$$ z \mapsto \dfrac{az + b}{cz + d} $$

where $a,b,c,d \in \mathbb{Z}$ and $ad - bc = 1$. Moreover, these are the only such conformal automorphisms of the Ford circles.

Now, for a quadratic irrational $\phi$ with Galois conjugate $\psi$, we can find a $2 \times 2$ matrix $A \in SL(2, \mathbb{Z})$ such that its eigenvectors are $(\phi, 1)$ and $(\psi, 1)$. This corresponds in the obvious way to a conformal automorphism $f$ of the Ford circles which fixes the two points $\phi$ and $\psi$.

Since $f$ is conformal, fixes $\mathbb{R}$ and fixes the point $\phi$, then to linear order it is given by a scaling $g$ about $\phi$. That is to say, if a point $x \in \mathbb{C}$ satisfies $|x - \phi| < \varepsilon$, then the displacement $|f(x) - g(x)| = o(\varepsilon)$.

---

You asked whether all other irrationals have the property that your zoom sequence contains two pictures that appear indistinguishable. If we instead pose the condition 'there exists an infinite convergent subsequence of pictures', then a sufficient condition is that if the sequence of continued fraction convergents is given by:

$\dfrac{a_1}{b_1}, \dfrac{a_2}{b_2}, \dfrac{a_3}{b_3}, \cdots$

then the sequence of ratios between successive denominators:

$\dfrac{b_2}{b_1}, \dfrac{b_3}{b_2}, \dfrac{b_4}{b_3}, \cdots$

contains a subsequence which converges, as then we have a sequence of automorphisms $f_i$ with the property that:

• $f_i(\dfrac{a_{k_i}}{b_{k_i}}) = \dfrac{a_{k_{i+1}}}{b_{k_{i+1}}}$
• $f_i(\dfrac{a_{k_i+1}}{b_{k_i+1}}) = \dfrac{a_{k_{i+1}+1}}{b_{k_{i+1}+1}}$

and for all sufficiently small balls around $\phi$, the automorphisms converge to a scaling about $\phi$ and the result follows as before.

On the other hand, if the ratios of successive denominators grow without bound, then you can actually find a sequence of pictures which converges to a zoomed-out picture of Ford circles. In your animation of $\frac{1}{\pi}$, you can see this because the next convergent after $\frac{355}{113}$ has a huge denominator.

Hence for every irrational real, you can find a convergent sequence of pictures.

Answer 2

Define the parent of a Ford circle to be the smaller of its two larger neighbors; then this parent relation defines the Stern–Brocot tree on the points of tangency of the circles. The path in this tree to a given real number is closely related to its continued fraction expansion. A path in the tree can be represented combinatorially by a binary sequence that determines whether to go left or right at each step, and this sequence is periodic exactly for the real numbers that have periodic continued fractions. That is, for the quadratic irrationals. It seems very likely to me that this periodicity in left-right steps is what is leading to your perceived periodicity in the relative sizes and positions of the circles, and that (once you determine an appropriate measure of shape similarity) this can be proven more rigorously: the shapes become approximately periodic when the left-right sequence in the tree is (after finitely many steps) exactly periodic.