Classical electromagnetism (with no sources) follows from the actions$$S = \int d^4x\left(-{1\over4}F_{\mu\nu}F^{\mu\nu}\right),\text{ where }F_{\mu\nu} = \partial_\mu A_\nu - \partial_\nu A_\mu.$$The Lagrangian for $A_\mu$, including a gauge fixing term, is$$\mathcal{L} = -{1\over4}F^2 - {\lambda\over2}(\partial_\mu A^\mu)^2.$$Could anyone show or supply me a reference for the computation of the equal time commutators $[\dot{A}_\mu(\textbf{x}), A_\nu(\textbf{y})]$ and $[\dot{A}_\mu(\textbf{x}), \dot{A}_\nu(\textbf{y})]$ for general $\lambda$ and the fact that they simplify for $\lambda = 1$? I need this result, but I am not a physicist by training.
1 Answer
$\begingroup$
$\endgroup$
3
the answer is on page 189 of Field Quantization by Greiner & Reinhardt (their $\zeta$ is your $\lambda$):
answered Oct 10, 2015 at 17:33
-
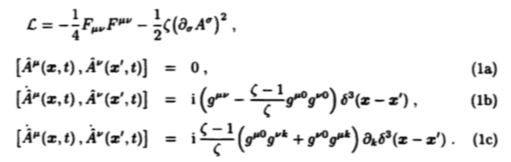
$\begingroup$ the Lagrangian in the first line of the posted scan is their Equation 7.2 on page 172 and in Eq. 7.88 on page 189; it has the form you want, doesn't it? $\endgroup$ Commented Oct 10, 2015 at 18:18
-
$\begingroup$ Yes, I see that it is their Equation 7.88. But on page 190 where the book carries out the computation to show (1a), (1b), and (1c), they use the Lagrangian $\mathcal{L}'' = -{1\over2}\partial_\mu A_\nu \partial^\mu A^\nu - {{\zeta-1}\over2}(\partial_\nu A^\nu)^2$, which confuses me, as this is different from Equation 7.88. $\endgroup$– user78817Commented Oct 10, 2015 at 18:20
-
$\begingroup$ I would think the two forms are equivalent upon an integration by parts in the action, see page 173. $\endgroup$ Commented Oct 10, 2015 at 18:23