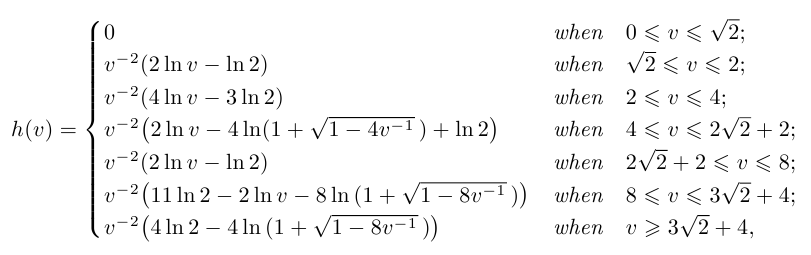Number of height-limited rational points on a circle

Question

Consider origin-centered circles $C(r)$ of radius $r \le 1$. I am seeking to learn how many rational points might lie on $C(r)$, where each rational point coordinate has height $\le h$. For example, these are the rationals in $[0,1]$ with $h \le 5$: $$ \left( 0,\frac{1}{5},\frac{1}{4},\frac{1}{3},\frac{2}{5},\frac{1}{2},\frac{3}{5}, \frac{2}{3},\frac{3}{4},\frac{4}{5},1 \right) $$ Rational points of height $\le h$ have both coordinates from this list, multiplied by $\pm 1$.

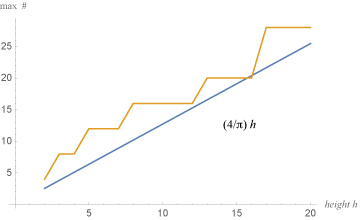
Q. What is the growth rate of the maximum number of rational points of height $\le h$ on $C(r)$, $r \le 1$, as a function of $h$?

Here is a bit of data up to $h=20$:

---

         

---

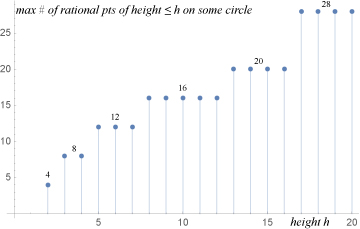
For example, for $h=7$, $C(\frac{5}{7})$ passes through these $12$ points: $$ \left( -\tfrac{4}{7} , -\tfrac{3}{7} \right), \left( -\tfrac{3}{7} , -\tfrac{4}{7} \right), \left( 0 , -\tfrac{5}{7} \right), \left( \tfrac{3}{7} , -\tfrac{4}{7} \right), \left( \tfrac{4}{7} , -\tfrac{3}{7} \right), \left( \tfrac{5}{7} , 0 \right), $$ $$ \left( \tfrac{4}{7} , \tfrac{3}{7} \right), \left( \tfrac{3}{7} , \tfrac{4}{7} \right), \left( 0 , \tfrac{5}{7} \right), \left( -\tfrac{3}{7} , \tfrac{4}{7} \right), \left( -\tfrac{4}{7} , \tfrac{3}{7} \right), \left( -\tfrac{5}{7} , 0 \right) $$ If I've calculated correctly, no circle passes through more than $12$ points of height $\le 7$. Circles that achieve these maxima are illustrated below.

---

         
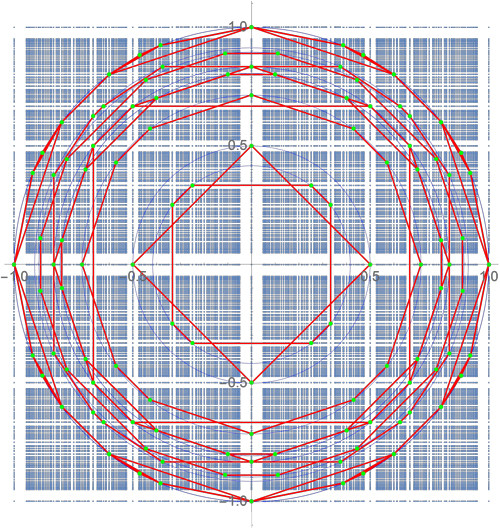
          ^{Background points are the rational points of height $h \le 20$.}

---

Added. Since the radii that achieve the maxima I found for $13 \le h \le 20$ are all exactly $1$, it may be that the question can be reduced to counting the number of rational points of height $\le h$ on just specifically $C(1)$.

---

Answered. Lucia's answer matches even the small-$h$ data I gathered:

---

         

---

Answer 1

I'll content myself with counting the number of points on $C(1)$ (which should surely be close to the maximum) -- the answer is quite nice, it is about $ \frac{4}{\pi } h$.

To see this, note that we are counting essentially Pythagorean triples $u^2-v^2, 2uv, u^2+v^2$, with $u^2+v^2\le h$ and we may suppose that $u$ and $v$ are non-negative, that $u$ and $v$ are coprime, and that $u^2+v^2$ is odd. The lattice point count we need is four times this number, since we must also count the lattice point $(2uv/(u^2+v^2),(u^2-v^2)/(u^2+v^2))$ (in addition to $((u^2-v^2)/(u^2+v^2),2uv/(u^2+v^2))$, and we must also allow the $2uv/(u^2+v^2)$ coordinate to be negative).

Thus to summarize we want $$ 4 \sum_{n\le h, n \text{ odd }} R(n), $$ where $R(n)$ is the number of ways of writing $n$ as $u^2+v^2$ with both $u$ and $v$ non-negative and coprime (taking care to set $R(1)$ to be $1$). A little number theory, going back to Fermat, gives that $R(n)$ is a multiplicative function with $R(2^k)=0$ (so we don't have to worry about $n$ odd anymore), $R(p^k)=2$ for $p\equiv 1\pmod 4$ and $k\ge 1$, and $R(p^k)=0$ if $p\equiv 3\pmod 4$. For example if $h=20$, then $R(1)=1$, $R(5)=2$, $R(13)=2$, and $R(17)=2$ and the rest are zero, and the number here is $28$ as in the numerics.

From here a standard argument (or one can do this via counting lattice points in a circle) leads to the asymptotic $$ 4 \sum_{n\le h} R(n) \sim 4 \frac{1}{2} \prod_{p\equiv 1 \pmod 4} \Big(1+\frac{2}{p}+\frac{2}{p^2}+\ldots \Big) \Big(1-\frac 1p\Big) \prod_{p\equiv 3\pmod 4} \Big(1-\frac 1p\Big) h, $$ and the above simplifies (using $1-1/3+1/5-1/7+\ldots =\pi/4$ and $1/1^2+1/3^2+1/5^2+\ldots = \pi^2/8$) to give $$ \sim 2 \frac{\pi/4}{\pi^2/8} h = \frac{4}{\pi} h. $$

One should be able to refine this to count lattice points on other circles as well, and thus show that radius $1$ does achieve the maximum.

Answer 2

Another nice question is about the distribution of the lengths of arcs connecting rational points on the unit circle. Let $Q \ge 3$ and let $$ (u_{0},v_{0}) = (1,0), \quad (u_{1},v_{1}), \quad (u_{2},v_{2}),\quad \ldots, \quad (u_{N},v_{N}), \quad (u_{N+1},v_{N+1}) = (0,1) $$ be all the rational points on the unit circle in the first quadrant, in increasing order of the polar angle $\varphi_{j} = \arctan{(v_{j}/u_{j})}$, and such that the denominators of all the fractions $u_{j}, v_{j}$, $1\le j\le N$, do not exceed $Q$. Further, let $\theta_{j} = \varphi_{j}-\varphi_{j-1}$ be the length of an are with ends at neighbouring points. The problem is to find the limit distribution of the normalised quantities $\theta_{j}$ as $Q$ grows unboundedly. Since $$\sum\limits_{j=1}^{N+1}\theta_{j}=\frac{\pi}{2},$$ it follows that the mean value of the arc length $\theta_{j}$, which is equal to $\pi/(2(N+1))$, is of the order of $Q^{-1}$ in view of the asymptotic behavior $N = N(Q) \sim Q/\pi.$ Take an arbitrary positive $t > 0$ and let $N(Q; t)$ denote the number of arcs such that $\theta_{j}\le\frac{t}{Q}.$ Here is the result of the article Distribution of rational points on the circle of unit radius by M. A. Korolev and A. V. Ustinov.

Theorem. As $Q\to +\infty$, the following equation holds: $$N(Q;t)=N(Q)\int_{0}^{t}h(v)dv+O\bigl(t^{1/2}Q^{5/6}(\log{Q})^{4/3}\bigr),$$ where the distribution density $h(v)$ is given by the equations and the constant in the symbol $O$ is absolute.

The graph of the function $h$: