Let $\Omega^{n+1}S^{n+1}$ be the base-pointed $(n+1)$-iterated loop space on the $(n+1)$-sphere. In the paper The homology of $\mathcal{C}_{n+1}$-spaces, $n\geq 0$, F. Cohen, Lecture notes in mathematics Vol. 533, page 226, Theorem 3.2, it is proved that as a $AR_n\Lambda_n$-Hopf algebra, $$ H_*(\Omega^{n+1}S^{n+1};\mathbb{Z}_p)\cong GW_n H_*(S^0;\mathbb{Z}_p) $$ for primes $p\geq 2$.
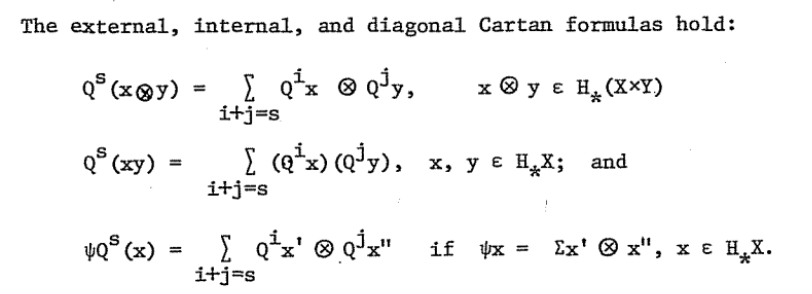
Question: In page 223, it is said that "the coproduct are determined by the diagonal Cartan formulas (page 213, (4))". I do not understand how to write the coproduct of $H_*(\Omega^{n+1}S^{n+1};\mathbb{Z}_p)$ explicitly?
In the paper On the homology of configuration spaces, Section 4, as an associative and commutative $\mathbb{Z}_p$-algebra, a generator set of $H_*(\Omega^{n+1}S^{n+1};\mathbb{Z}_p)$ is given. I want to know how to obtain the coproduct formulas of $H_*(\Omega^{n+1}S^{n+1};\mathbb{Z}_p)$ as a coalgebra.
-------------------------------------------------------------------------------------------------------------------------------------==========================================================================
For the answer below, I have a question.
In the paper On the homology of configuration spaces, Section 4.1, a basis for the graded vector space $H_*(\Omega^m S^m;\mathbb{Z}_2)$ is given as
$(*)$ $$ u_0, u_1, Q_I u_0, Q_I u_1 $$ where $0,1$ denote the two generators of $H_0(S^0;\mathbb{Z}_2)$ and $Q_I=Q_{i_1}Q_{i_2}\cdots Q_{i_r}$ for $I=(i_1,i_2,\cdots, i_r)$, $0<i_1\leq i_2\leq \cdots\leq i_r<m$.
By the Cartan formula (cf. The homology of $\mathcal{C}_{n+1}$-spaces, $n\geq 0$, F. Cohen, page 213)
In the answer below, we calculated that
$(**)$ $$\Delta_* Q_I u_0=\sum_{t_1=0}^{i_1}\sum_{t_2=0}^{i_2}\cdots \sum _{t_r=0}^{i_r}Q_{t_1}Q_{t_2}\cdots Q_{t_r}u_0\otimes Q_{i_1-t_1}Q_{i_2-t_2}\cdots Q_{i_r-t_r}u_0, $$
$(***)$ $$\Delta_* Q_I u_1=\sum_{t_1=0}^{i_1}\sum_{t_2=0}^{i_2}\cdots \sum _{t_r=0}^{i_r}Q_{t_1}Q_{t_2}\cdots Q_{t_r}u_1\otimes Q_{i_1-t_1}Q_{i_2-t_2}\cdots Q_{i_r-t_r}u_1. $$
Is this result correct or wrong?
Question: The problem is that $Q_{t_1}Q_{t_2}\cdots Q_{t_r}u_0$, $Q_{i_1-t_1}Q_{i_2-t_2}\cdots Q_{i_r-t_r}u_0$, $Q_{t_1}Q_{t_2}\cdots Q_{t_r}u_1$, $Q_{i_1-t_1}Q_{i_2-t_2}\cdots Q_{i_r-t_r}u_1$ are not included in the basis. It does not make sense. Why?
===============================================
I find the Adem relations for $p=2$
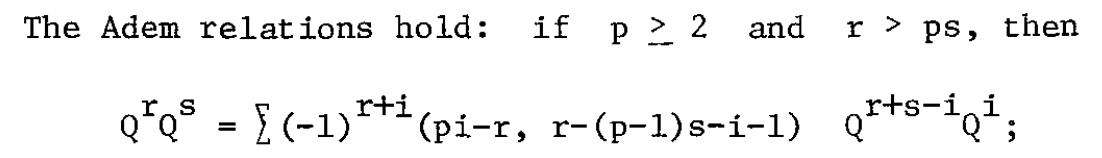
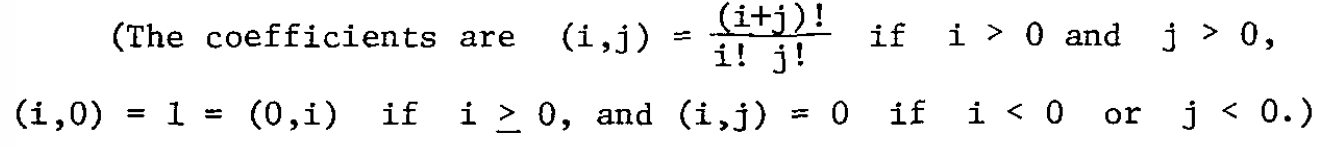
i.e.
for $t_1>2t_2$,
$$ Q_{t_1}Q_{t_2}=\sum_{t_1/2 \leq i\leq t_1-t_2-1}(-1)^{t_1+i}(2i-t_1,t_1-t_2-i-1)Q^{t_1+t_2-i}Q^i. $$
How to apply the Adem relation to simplify equations $(**)$, $(***)$ to sums of tensor products of basis given in $(*)$?
