Consider $$y_t - \Delta y = f$$ $$y(0) = y_0$$ with zero boundary condition. Let $a(t,.,.)$ be the bilinear form associated to $-\Delta$. We have two definitions of weak solutions:
We have $y \in L^2(0,T;H^{1}_0)$ with $y_t \in L^2(0,T;H^{-1})$ is a A-weak solution of problem (1) if $$\langle y_t(t), v \rangle + a(t,y(t),v) = \langle f(t), v \rangle$$ for all $v \in H^1_0$ and almost all $t \in [0,T]$.
and
We have $y \in L^2(0,T;H^{1}_0)$ with $y_t \in L^2(0,T;H^{-1})$ is a B-weak solution of problem (1) if $$\int_0^T \langle y_t(t), v(t) \rangle + \int_0^T a(t,y(t),v(t)) = \int_0^T \langle f(t), v(t) \rangle$$ for all $v \in L^2(0,T;H^1_0)$.
The claim is that these two notions of solution are the same. For one side, the proof is like this.
Let $y$ be an A-weak solution of (1). We shall show that $$ \langle y_t(t), v(t) \rangle + a(t,y(t),v(t)) = \langle f(t), v(t) \rangle \tag{1.61}$$ for all $v \in L^2(0,T;H^1_0)$ for a.a. $t$. This is because:
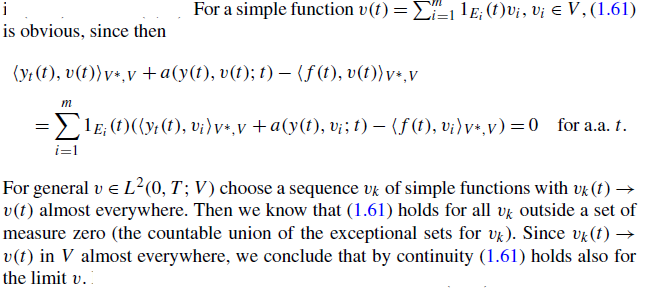
So he proves (1.61) for all simple functions, and hence for the simple functions $v_k$ that converge to an arbitrary $v$, and the null set does not depend on $v_k$. Then he passes to the limit $v_k(t) \to v(t)$ for a.e. $t$. So in the end the null set does depend on $v$ (as it must do). So why the whole fuss about getting rid of the null set and making it independent of $v_k$ in the first place? What gets messed up if he didn't do that? (This is on page 43 of "Optimization with PDE constraints" by Hinze, Pinnau, Ulbrich.)
(This question was originally posted on MSE many months ago by CGuy. Reposted here with permission).
