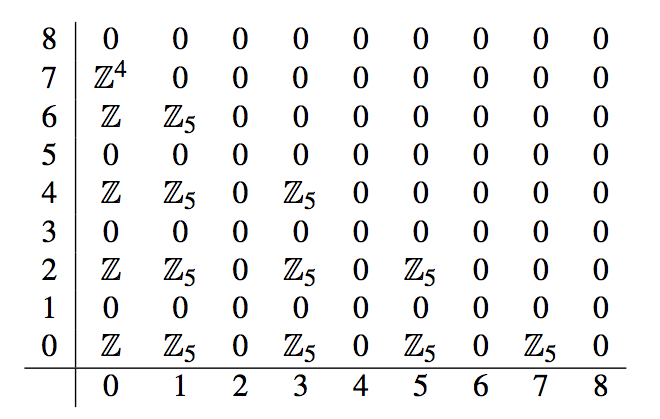
$Z_p$:=cyclic group of order $p$.
I want to understnd $H_\ast(\mathbb{C} P^{n}/Z_{n+1};Z)$ with $(n+1)$ being a prime number,and the action is given by permuting the homogeneous coordinates.
For example,let's take $n=4$.The fixed point set $(\mathbb{C} P^{4})^{Z_5}$is 5 points.The action is free out of the the fixed point set (since $Z_5$ has no nontrivial subgroups)
My strategy is:
1.The quotient map restrictied to the free part,which is $\mathbb{C} P^{4}-(\mathbb{C} P^{4})^{Z_5}\to \mathbb{C} P^{4}/Z_5-(\mathbb{C} P^{4})^{Z_5}$, is a covering ,so we could compute $H_\ast(\mathbb{C} P^{4}/Z_5-(\mathbb{C} P^{4})^{Z_5})$ using spectral sequence $$H_i(BZ_5,H_j(\mathbb{C} P^{n}-(\mathbb{C} P^{n})^{Z_5}))\Rightarrow H_{i+j}(\mathbb{C} P^{4}/Z_5-(\mathbb{C} P^{4})^{Z_5})$$
2.$\mathbb{C} P^{4}=(\mathbb{C} P^{4}/Z_5-(\mathbb{C} P^{4})^{Z_5})\cup$ 5 copies of cone$(L^7$) ($L^7=S^7/Z_5$ is the lens space) .Use MV sequence 4 times to obtain the homology of the orbit space.
In step 1,there is an extension issue in the spectral sequence.In step 2,similar issue arises when i am in the situation $0\to A\to ?\to B\to 0$.
Is it possible to overcome this extension issue using some geometric observation?What's that.
and
Have people already computed the homology of orbit space of linear cyclic group action over $\mathbb{C} P^{n}$?
where the action is given by $$g\cdot[z_0,\cdots,z_n]:=[\zeta^{a_0}z_0,\cdots,\zeta^{a_n}z_n]$$ where $g$ is the generator of $Z_p$ and $\zeta$ is $p$-th root of unity,and $a_i$ s are some given nonnegative integers.For my problem above,I believe the action is equivalent to $$g\cdot[z_0,z_1,z_2,z_3,z_4]:=[z_0,\zeta^{}z_1,\zeta^{2}z_2,\zeta^{3}z_3,\zeta^{4}z_4]$$ by noticing that the regular representation of $Z_5$ over $\mathbb{C}^5$ is direct sum of the irreducible representations.