3
$\begingroup$

I am testing some numerical algorithms for computing the Laplace-Beltrami eigenvalues on the sphere. One thing that came up was computing the first eigenvalue of the "equilateral" spherical triangle which realizes the $4$-tilling of the sphere. I could find some numerical computations giving the value $5.189..$. I found values close to this with my numerical method. In order to test my accuracy I wanted to find a more precise approximation of this eigenvalue.

I am interesting to know if an analytical formula for the first eigenvalue of such a triangle can be found.

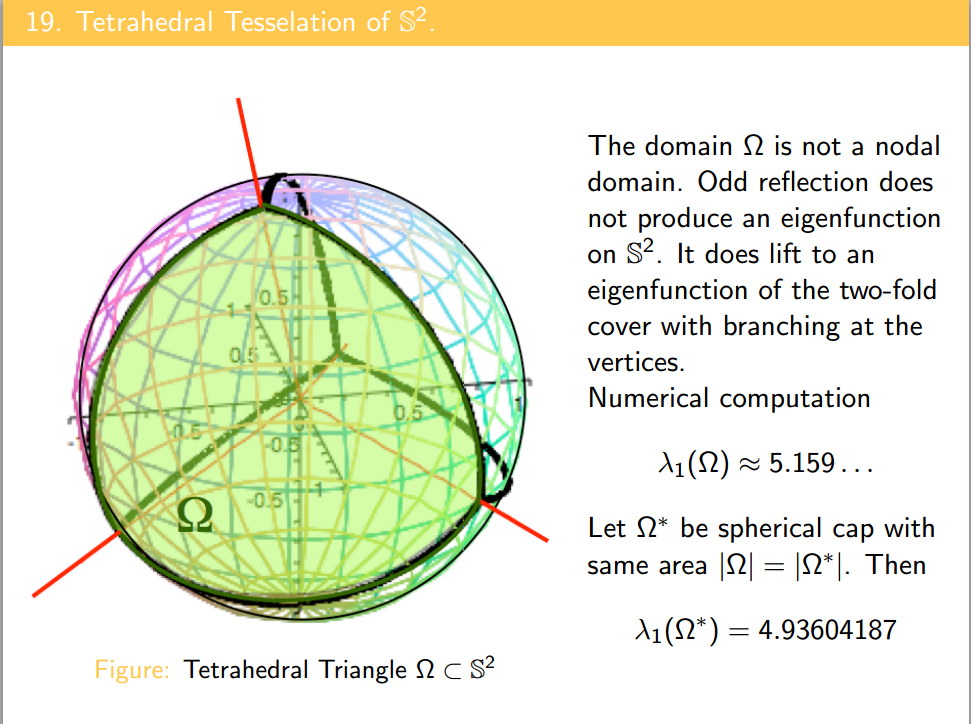
Here is a picture representing one such triangle: enter image description here

The slide is taken from this presentation: http://www.math.utah.edu/~treiberg/EigenvalCapture.pdf

The article in which the corresponding numerical computation is done can be found here: http://www.math.utah.edu/~treiberg/drunker-submit.pdf

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

There is a closed formula for the eigenvalues of the right angled spherical triangle, which can be found in Vilenkin's book (Special Functions and the Theory of Group representations). For (a little) more, see The spectra of the spherical and euclidean triangle groups, by Mark Harmer (arxiv.org/abs/math/0702479).

Improve this answer
$\endgroup$
1
  • $\begingroup$ Where do I look in Vilenkin's book for this? I don't see it. $\endgroup$
    – Dustin G. Mixon
    Commented Nov 16, 2023 at 18:42

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .