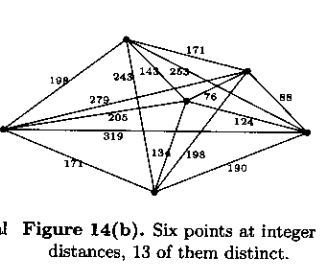
Richard Guy has shown that there are six points in the plane—no three collinear, no four cocircular—such that all interpoint distances are rational.
Guy, Richard. Unsolved Problems in Number Theory. Vol. 1. Springer, 2004. D20. Six general points at rational distances. p.185ff:
My question is:
Q. Is it known that six is the maximum possible? Or have examples been found in the intervening decade that supersede Guy's result?
Update. This question is essentially a duplicate of "Integer-distance sets." Apologies.
