I am trying to study mixing of diffusing particles like it was done by E. Ben-Naim On the Mixing of Diffusing Particles.
The picture below shows the idea how permutations and inversion numbers reflect the mixing of diffusing particles.
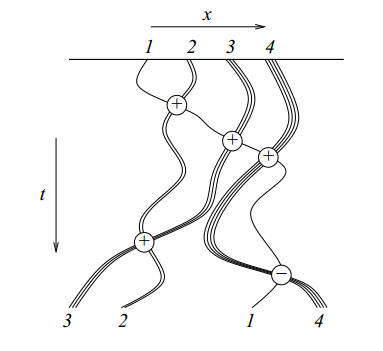
However, I am very intrested in effects we could discover from diffusion equation while taking into account that the number of mixing particles is very large but it is finite. If you look at Ben-Naim's approach you can see a Gaussian for normal distribution. This is quite good, but what about 'hidden information' for the finite number $N$ of mixing particles? So, the new distribution function ( Gaussian, where we know the error of approximation) looks like:
$F_N (x)=Φ(x)+\frac{3}{50\sqrt{2π}} e^{-x^2/2} (x^3-3x) \frac {6N^3+21N^2+31N+31} {N(2N+5)^2 (N-1)}+O(\frac{1}{N^2} )$
In other words it is about $\frac{3}{2N}+O(\frac{1}{N^2})$ We get some neat formula. But I still wonder a) how to construct the diffusion equation and b) what information we get from it?
Any help are highly welcomed. Thank you.
