Consider a pair of disjoint, congruent helices $H_1$ and $H_2$ passing through one another in the following sense. (Caveat lector: This question is not of general interest! It is also long.)
$H_1$ is a section of a unit-radius helix along the $z$-axis:
$$x = \cos \theta ,\; y = \sin \theta ,\; z = h \frac{\theta}{2 \pi} \;.$$
$H_2$ is congruent to $H_1$, but first twisted about $z$ a bit, and then passing
through the origin at a different orientation: left figure below.
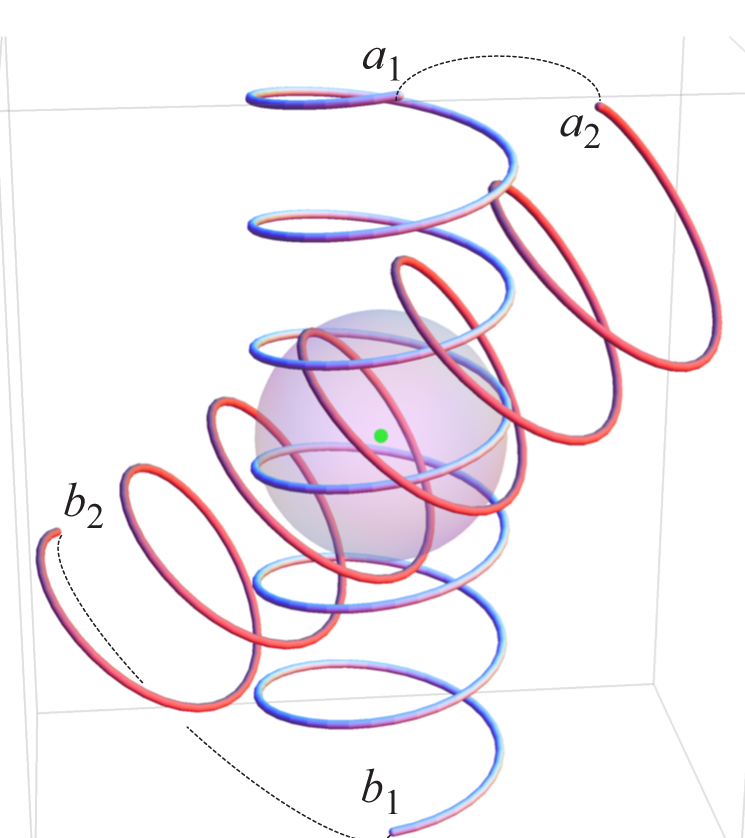
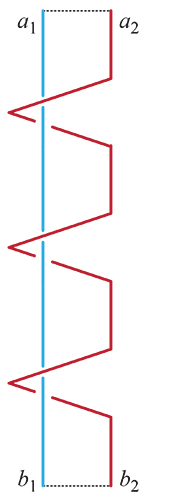
The twisting ensures that the two helices do not intersect.
Both are tangent to the unit-radius sphere centered on the origin.
Now connect up their ends as illustrated to form a loop.
I believe this forms an unknot independent of the height-tightness $h$
of the helix (sometimes called its "pitch"), as illustrated to the right above.
My general question is:
Q1. How complex is the knot formed by $n$ such helices, of height-tightness $h$?
To make this question more precise, define the complexity of a knot as, say, its crossing number. The answer to Q1 may depend on how the ends are tied together. I would be interested in bounds over all possible tyings-together. For example, assume no helix axis lies in the $xy$-plane, and label the endpoints above the $xy$-plane $a_i$ and those below $b_i$. For $n$ odd, connect $(a_1,a_2), (a_3,a_4), \ldots, (a_n, b_1)$, and connect $(b_2,b_3),\ldots,(b_{n-1},b_n)$; $n=3$ is illustrated below. For $n$ even, the connections are among the $a_i$'s and among the $b_i$'s. Now the possible "tyings-togethers" can be captured by permuting the label subscripts.
Q2. What is the smallest crossing number that can result (over all possible tyings-together) of knots formed by $n$ such helices, of height-tightness $h$?
Maybe this still isn't a sharp question, if the result depends on the orientations
of the helices. In that case, I would still be interested in the least-complex
knot achievable. Or the most-complex achievable.
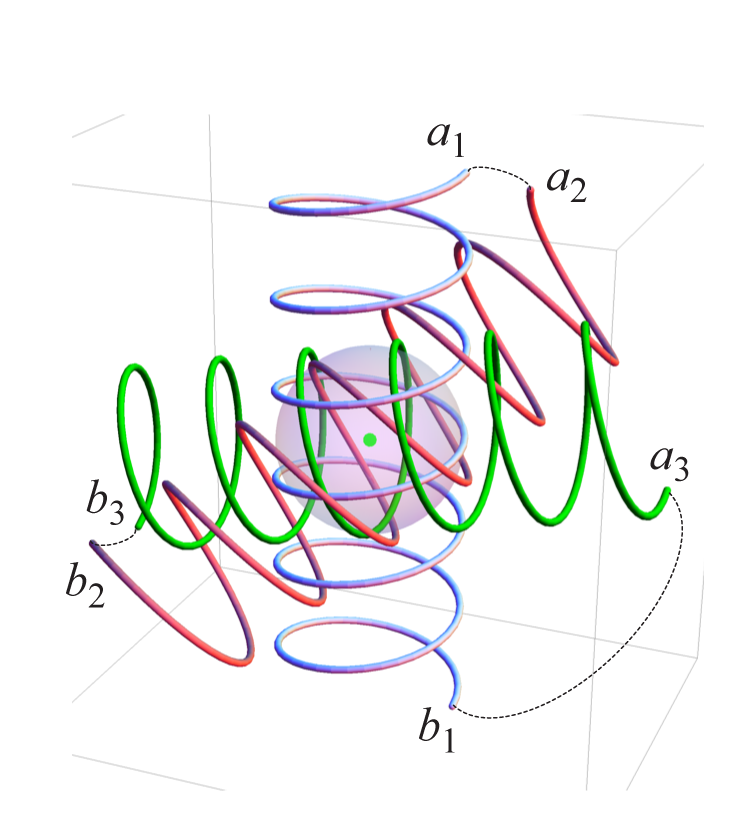
Motivation. I realize this is a strange question of little interest to anyone but me. :-) My interest derives from the following consideration. If the helices are considered rigid bodies (e.g., rigid slinkys), then the collection is highly tangled, in the sense that quite a bit of work would be need to separate them (and "quite a bit" can be formalized). What I am wondering is if the rigid entanglement is mirrored by topological complexity. It would be especially interesting if it is not, i.e., if the knot complexity can be low even when the rigid entanglement is high.
However, I am having difficulty seeing what knots result from this construction.
