1
$\begingroup$

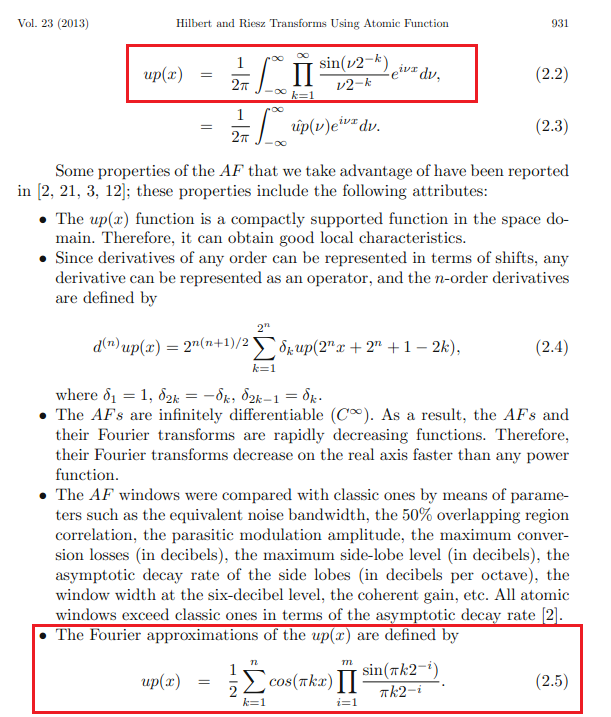
We have

$$A=\frac{1}{2\pi}\int_{-\infty}^{\infty}\prod_{i=1}^{\infty}\frac{\sin(v2^{-k})}{v2^{-k}}e^{ivx}dx$$

$$B=\frac{1}{2}\sum_{k=1}^{n}\cos (\pi kx)\prod_{i=1}^{m}\frac{\sin(\pi k2^{-i})}{\pi k2^{-i}}$$ where B is the Fourier approximation of A (However, I don't know how to verify this).

My question is:Are the two functions equal(update:if $n,m \to \infty$)? If not, what relation are they, such as $A\approx B$?

The question comes from(Page 931, see the picture) More details: https://www.evernote.com/shard/s192/sh/480adb68-2ced-4839-8710-c770db5020ff/bc595875559ad0125ebd79976d7c3fb3

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ They're certainly not equal since $B$ depends on $n,m$ and $A$ does not. $\endgroup$
    – Nate Eldredge
    Commented Dec 2, 2013 at 16:36
  • $\begingroup$ I guess the question is whether they become equal in the limit $n,m\rightarrow\infty$, which at least formally seems to be the case. $\endgroup$
    – Carlo Beenakker
    Commented Dec 2, 2013 at 17:05

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .