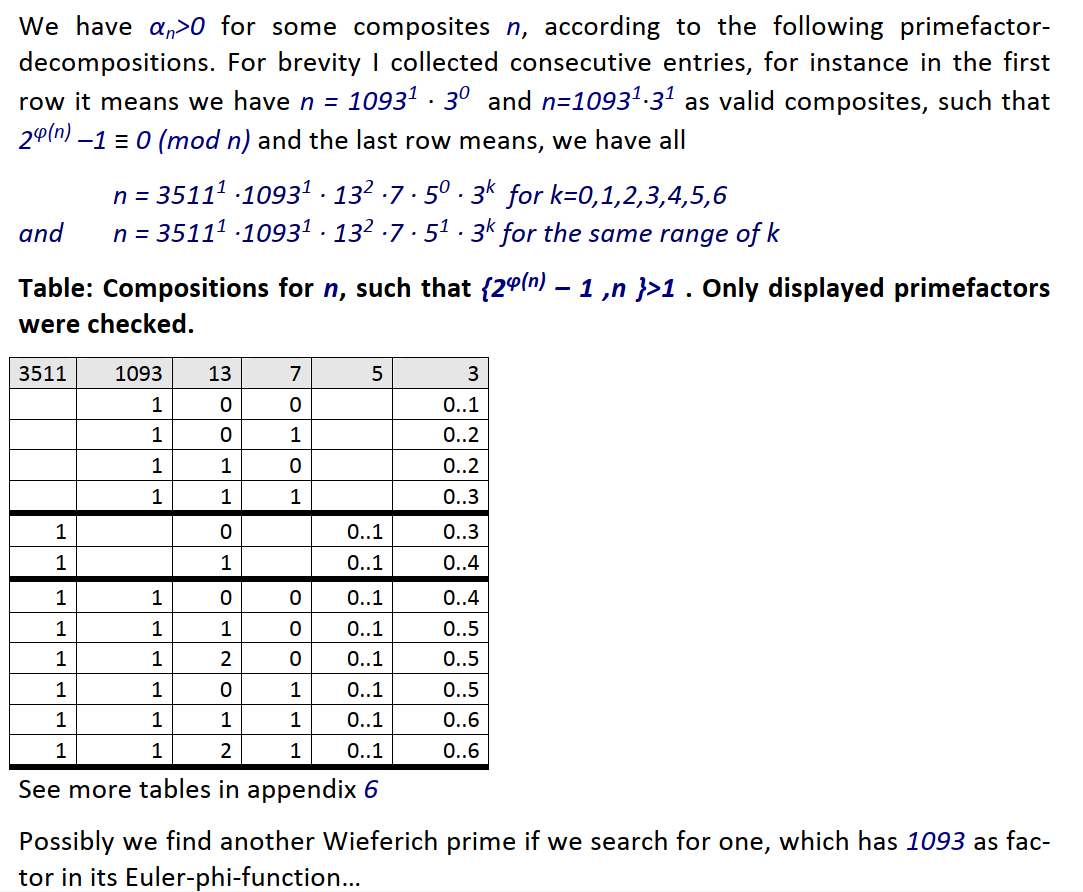
While studying a certain Diophantine equation in the squarefree integer $k \ge 2$, I believe I have proven the necessary restriction
$$2^{k-1} \equiv 1\!\!\pmod{k^2}. \qquad(\star)$$
Based on what I read about Wieferich primes on Wikipedia (http://en.wikipedia.org/wiki/Wieferich_prime), if $k$ is a prime, it must be a Wieferich prime. So far, so good.
However, I haven’t found anything — on Wikipedia or elsewhere — that proves there are no composite solutions to the congruence ($\star$). Is that statement true? If so, what’s an easy proof? If not, what's an easy disproof?
Many thanks,
Kieren.
n.b. I asked this same question on MSE earlier today (https://math.stackexchange.com/questions/497810/are-wieferich-primes-the-only-solutions-to-2n-1-equiv-1-pmodn2), but now realize it might be more suitable here due to its possible complexity. Apologies if that's inappropriate.