Can Reidemeister 3 be weakened?

Question

If you take the diagram of the Reidemeister 3 move and "shortcircuit" two ends, you get (click https://i.sstatic.net/gfOKy.jpg if Imgur hotlink doesn't work):
          
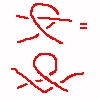
I have circumstantial evidence that this weaker version is actually equivalent to R3. (Only in a computational sense! My hypothesis: If A and B are two diagrams of the same knot, while it might not be actually possible to go from A to B by applying weak R3 moves (+R2+R1, of course), the assumption that weak R3 holds forces invariant(A)=invariant(B) for any Lie group derived invariant. Likewise, in Kauffmans abstract tensor framework, just assume weak R3, solve and get the rest of the Yang-Baxter equation for free.)
Thus: Is there work on "alternative moves"? Can you construct a counterexample? (I.e. an pseudo-invariant which is constant under weak R3+R2+R1, but not under R3? The example must have a skein equation, though.)

Answer 1

I am very very skeptical that you can recover R3 from your weak R3. Instead here's what I expect is happening in your examples. Let V be the vector space spanned by diagrams with 6 boundary points. There's a pairing on V given by connect up all the boundary points and apply your invariants. You might as well treat as 0 anything in V that's in that's in the radical of this form, it won't change your invariants. So what we want to know is whether the difference of the two sides of R3 (which I'll call v) is in the radical of this form for your particular examples. When you take inner products of v with various other vectors in V you do the calculation by applying whatever skein relations you have. It's quite possible that in the presence of your other specific skein relations your weak R3 is all you need in order to show that v has inner product 0 with everything. But this would be because you've imposed enough other special skein relations.