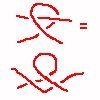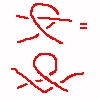If you take the diagram of the Reidemeister 3 move and "shortcircuit" two ends, you get (click https://i.sstatic.net/gfOKy.jpg if Imgur hotlink doesn't work):

I have circumstantial evidence that this weaker version is actually equivalent to R3. (Only in a computational sense! My hypothesis: If A and B are two diagrams of the same knot, while it might not be actually possible to go from A to B by applying weak R3 moves (+R2+R1, of course), the assumption that weak R3 holds forces invariant(A)=invariant(B) for any Lie group derived invariant. Likewise, in Kauffmans abstract tensor framework, just assume weak R3, solve and get the rest of the Yang-Baxter equation for free.)
Thus: Is there work on "alternative moves"? Can you construct a counterexample? (I.e. an pseudo-invariant which is constant under weak R3+R2+R1, but not under R3? The example must have a skein equation, though.)

If you take the diagram of the Reidemeister 3 move and "shortcircuit" two ends, you get (click https://i.sstatic.net/gfOKy.jpg if Imgur hotlink doesn't work):
          
I have circumstantial evidence that this weaker version is actually equivalent to R3. (Only in a computational sense! My hypothesis: If A and B are two diagrams of the same knot, while it might not be actually possible to go from A to B by applying weak R3 moves (+R2+R1, of course), the assumption that weak R3 holds forces invariant(A)=invariant(B) for any Lie group derived invariant. Likewise, in Kauffmans abstract tensor framework, just assume weak R3, solve and get the rest of the Yang-Baxter equation for free.)
Thus: Is there work on "alternative moves"? Can you construct a counterexample? (I.e. an pseudo-invariant which is constant under weak R3+R2+R1, but not under R3? The example must have a skein equation, though.)

Another thing I "know" since 30 years but only recently I found a clue:
Assume a R matrix (graphic: %) can be decomposed into "projectors" (graphic: >=< ). (See the conditions here: Matrix decomposition the other way). It seems to me that this is always possible if it's the R matrix of a Lie group, but may work even if not (i.e. just a random Yang-Baxter solution). In any case, you can apply the usual trick - if you can pull a string over all nodes >= , i.e. Reidemeister 3 for nodes holds, Reidemeister 3 for crossings follows. Of course your projectors must obey the usual 6j rules either. Now I found something fascinating: The diagrams for Biedenharn-Elliott and Reidemeister 3 for nodes have five open ends. I played with "quadratic" graphs with only four open ends, and the results are exactly the same - the consistency rules following from the diagrams are exactly Biedenharn-Elliott and Reidemeister 3 for nodes again.
After "undecomposing" everything again, the following weakened Reidemeister 3 version results: 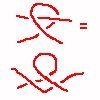(hope the image comes through - https://i.sstatic.net/gfOKy.jpg)
The above musings are not even a handwaving proof, so I won't dare to state "For Lie groups, Reidemeister 3 follows from weak Reidemeister 3". Still, it would be epic if one could replace the annoying 3+3-tangle condition with 2+2. And when I solve abstract tensor equations, I know not a single counterexample either. So my question: Do you know a counterexample - any construction where weak Reidemeister 3 holds but Reidemeister 3 is violated?

If you take the diagram of the Reidemeister 3 move and "shortcircuit" two ends, you get (click https://i.sstatic.net/gfOKy.jpg if Imgur hotlink doesn't work):

I have circumstantial evidence that this weaker version is actually equivalent to R3. (Only in a computational sense! My hypothesis: If A and B are two diagrams of the same knot, while it might not be actually possible to go from A to B by applying weak R3 moves (+R2+R1, of course), the assumption that weak R3 holds forces invariant(A)=invariant(B) for any Lie group derived invariant. Likewise, in Kauffmans abstract tensor framework, just assume weak R3, solve and get the rest of the Yang-Baxter equation for free.)
Thus: Is there work on "alternative moves"? Can you construct a counterexample? (I.e. an pseudo-invariant which is constant under weak R3+R2+R1, but not under R3? The example must have a skein equation, though.)