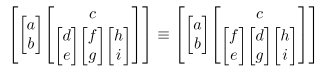8
$\begingroup$

Could anyone explain what higher dimensional algebra is?

I tried to look on the web but I couldn't find a satisfactory definition, the ones that I found are too vague. What I'm looking for is a good definition of higher dimensional algebra, what it deals with and some references about the subject.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ "Higher dimensional" can be taken as a synonym of "categorified". Does that help? $\endgroup$
    – André Henriques
    Commented Jun 19, 2011 at 21:20
  • 5
    $\begingroup$ Have you looked at the nLab at all? You might try searching for higher-dimensional categories, etc., on the nLab; this should keep you busy for quite a while. $\endgroup$
    – Todd Trimble
    Commented Jun 19, 2011 at 21:29
  • 4
    $\begingroup$ Th introductions of any of the papers titled "Higher Dimensional Algebra N: Blah" by John Baez and coauthors should give you a sense of the field. But note that JB is by no means the instigator of such study. Ronnie Brown is a strong proponent of the idea, especially in the area of algebraic homotopy. One could argue that some of the basic notions (well, 2-groups) were known to Grothendieck, Verdier and Sinh (and others) in the 60s and 70s. $\endgroup$
    – David Roberts ♦
    Commented Jun 19, 2011 at 23:32

3 Answers 3

Reset to default
14
$\begingroup$

My definition of higher dimensional algebra is that it is the study and application of algebraic theories with partial algebraic structures whose domains are defined by geometric conditions. I think the first study of such partial algebraic structures was in Higgins, P.~J. "Algebras with a scheme of operators". Math. Nachr. 27 (1963) 115--132.

The intuitive idea is also: do mathematical formulae have to be confined to a line? Can we have and use formulae in 2 or 3 or many dimensions? and can this be useful? If so we can expect to need rewriting of such formulae, and indeed this exists and is useful. You can find many 2-dimensional rewrites in the new book Nonabelian Algebraic Topology (European Mathematical Society, 2011), and with a pdf available. See for example the rotations in section 6.4, used in this mathoverflow answer on computing homotopies.

Those who have read Flatland will remember that the Linelanders had a rather limited existence! Can we really think that the brain works in one dimension only?

I mention that the idea of all this occurred to me in 1965 when I thought that the proof I had written out many times for the van Kampen Theorem for the fundamental groupoid should generalise to higher dimensions, if one had the right gadgets, seen as higher homotopy groupoids. 9 years later: whoopee! (work with Chris Spencer and with Philip Higgins). The first joint papers with Philip were published in 1978 and 1981.

Note that group objects in the category of groups are just abelian groups. But double groupoids, which are groupoid objects in the category of groupoids, are much more complicated than groups, and can model $2$-dimensional homotopical notions.

You can find presentations on some of these ideas on my preprint page, and including a presentation Theoretical Neuroscience in Delhi in 2003, which went down well! arXiv:math/0306223.

For my purposes I have needed a cubical context, which is not commonly followed, but in which it was easier to conjecture and prove the theorems I was thinking of. But the definition above seems quite general, and allows for a melding of algebra and geometry.

I also believe that Henry Whitehead's term `Combinatorial homotopy' and his actual work show he was thinking of generalising some methods of combinatorial group theory to higher dimensions. His notion of crossed module (1946) has proved an important feature of such ideas, and crossed modules later appeared in a key relations with $2$-groups and more generally with double groupoids.

In another direction the following diagram

inv

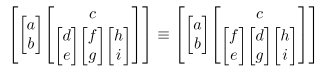
occurs in this paper on double semigroups.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ With regard to the origins of higher dimensional algebras, the answers to this question are relevant: mathoverflow.net/questions/168888/… $\endgroup$
    – Daniel Moskovich
    Commented Mar 20, 2016 at 12:12
  • $\begingroup$ One should also mention Charles Ehresmann. See my article discussing three themes in his work: groupoids, local-to-global,higher dimensions: arxiv.org/abs/math/0602499 $\endgroup$
    – Ronnie Brown
    Commented Mar 27, 2016 at 21:55
19
$\begingroup$

Ordinary algebra (monoids and their linear cousins rings) involves an operation that's written on a line. That is, you can multiple on the left or on the right. It's 1-dimensional algebra.

Higher dimensional algebra is algebra where it's more natural to write your operations on a higher dimensional manifold. For example, the Temperley-Lieb algebra is naturally 2-dimensional. You can multiple on the left, right, top, or bottom. Similarly Hopf algebras can be thought of as 2-dimensional, with the coproduct being related to multiplication in a different dimension.

Asking for a rigorous definition of "higher dimensional algebra" is counterproductive. It's a subject, not a mathematical object. But one way of making it more precise would be to say that higher dimensional algebra is the study of n-categories. Again, I think that last sentence is misleading, but at least n-categories are something you can look up definitions of.

A good place to start reading about higher dimensional algebra is going through back issues of this week in mathematical physics.

Improve this answer
$\endgroup$
6
  • 3
    $\begingroup$ Noah means this: math.ucr.edu/home/baez/TWF.html. A major, perhaps the predominant theme, is higher-dimensional algebra. By the way, have you looked at the series of papers by Baez and assorted co-authors which have the words Higher-Dimensional Algebra followed by a Roman numeral? Again, these should keep you busy for a while. $\endgroup$
    – Todd Trimble
    Commented Jun 19, 2011 at 21:41
  • 3
    $\begingroup$ A specific paper to start at might be math.ucr.edu/home/baez/rosetta.pdf . $\endgroup$
    – Qiaochu Yuan
    Commented Jun 19, 2011 at 22:45
  • $\begingroup$ This is exactly what am I looking for, thanks a lot. $\endgroup$
    – Giorgio Mossa
    Commented Jun 20, 2011 at 7:40
  • 1
    $\begingroup$ It may be also worth looking at Ronnie Brown's page: pages.bangor.ac.uk/~mas010/hdaweb2.htm since he was one of the first to coin the term higher dimensional algebra and in particular higher dimensional group theory. $\endgroup$
    – Tim Porter
    Commented Jun 20, 2011 at 18:10
  • $\begingroup$ (I will add the diagram: pages.bangor.ac.uk/~mas010/RonBrown.pdf) $\endgroup$
    – Tim Porter
    Commented Jun 20, 2011 at 18:11
8
$\begingroup$

I'll use this question as an opportunity to advertise a notion of "two-dimensional algebra" from my paper http://arxiv.org/pdf/0912.5307v2.

Definition:
A 2-algebra consists of a vector space $A$, two bilinear operations $$ a,b\mapsto ab\qquad\qquad\qquad\text{and}\qquad\qquad\qquad a,b\mapsto\, \begin{matrix} a\\ b\end{matrix} $$ and three distinguished elements $1$, $v$, and $v^{-1}$, subject to the axioms
• $\qquad 1a=a=a1$
• $\qquad (ab)c=a(bc)$
• $\qquad \begin{matrix}\stackrel{\displaystyle (ab)}{(cd)}\end{matrix} =\big(\begin{matrix}\stackrel{\displaystyle a}{c}\end{matrix}\big)\big(\begin{matrix}\stackrel{\displaystyle b}{d}\end{matrix}\big) $
• $\qquad v\Big(\begin{matrix}(\begin{matrix}\stackrel{\displaystyle a}{b}\end{matrix})\\\ c\end{matrix}\Big)v^{-1}=\Big( \begin{matrix}a\\\ (\begin{matrix}\stackrel{\displaystyle b}{c}\end{matrix})\end{matrix}\Big)$
• $\qquad vv=(\begin{matrix}\stackrel{\displaystyle 1}{v}\end{matrix})v(\begin{matrix}\stackrel{\displaystyle v}{1}\end{matrix})$
• $\qquad vv^{-1}=v^{-1}v=1$

Improve this answer
$\endgroup$
3
  • 4
    $\begingroup$ I'm impressed that you got all that to typeset. $\endgroup$
    – Noah Snyder
    Commented Jun 19, 2011 at 23:11
  • 3
    $\begingroup$ Note that this seems to be nothing but a $k$-monoidal structure on the $k$-category with one object associated to an algebra $A$ over the field $k$ connecting it with Noah's answer. $\endgroup$
    – Torsten Ekedahl
    Commented Jun 20, 2011 at 8:59
  • 2
    $\begingroup$ @Torsten: You are perfectly right... more precisely, it's a non-unital monoidal structure. Related: The above-described structure on $A$ induces a non-unital monoidal structure on $Rep_A$. However, it is not true that any (non-unital) monoidal structure on $Rep_A$ comes from such a structure on $A$. $\endgroup$
    – André Henriques
    Commented Jun 20, 2011 at 9:25

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .