Computing homotopies

Question

Oftentimes, in the standard algebraic topology books (May, Switzer, Whithead, for instance), there are tricky little proofs that depend on proving that two maps are homotopic. This is comparable to the way we build homotopies, lifts, etc. combinatorially in simplicial homotopy theory, but for some reason I never really acquired the skill-set (maybe the intuition?) to come up with these homotopies in the topological case. I'm just mystified how these little formulas are pulled out of thin air.

Am I missing a key technique that's often taught early-on in an algebraic topology course? Is it tricky even with practice? Have there been any papers that focus on systematic ways of generating these things?

I also noticed that in May's book, he oftentimes writes out explicit formulas for his homotopies, sometimes in a way that obscures the issue at hand (for instance, there is a homotopy that is described by an explicit formula, but it's nothing more than an explicit "representative of the natural homotopy" between the identity map and the constant map on a contractible based space.) How often can these seemingly arbitrary formulas be replaced with more canonical descriptions? (This last question is a soft question to people with experience in topology)

Answer 1

Sometimes easy geometric pictures have awkward seeming algebraic descriptions. On pages 6 and 7 of Concise, I gave examples where I both gave a geometric picture and explicit formulas to make the idea of such translation clear. In other cases, (as in cofiber homotopy equivalence) I just found it quick and easy to write down the homotopies (in terms of other homotopies). Sometimes it is just way too laborious to draw the pictures, other times it is too laborious to write the homotopies out. One should learn to be happily eclectic and absorb all techniques available.

Added by PLC: in the second sentence above, Professor May is referring to his text A Concise Course in Algebraic Topology. (When he taught me the course, the title of the draft copy he handed out to us was A Rapid Course..., but I guess the publishers didn't like that so much!)

Answer 2

The basic phenomenon is that often the best way to think about "little homotopies" is to use the geometric parts of your brain --- to use primarily your GPU (geometry processing unit), with your arithmetic processing unit, logic processing unit and lexical processing units all in the background, so to speak. However, when writing down a proof, it's customary, and usually easier to transcribe it into symbolic form. This tends to be a one-way process --- it's much harder to start from symbolic formulas and regenerate the geometric intuiton than to start from the geometric intuition and transcribe it into symbolic formulas.

It has become much easier to create reasonable figures illustrating geometric ideas than it used to be (say 20 or 30 years ago), but it's still hard. It's especially hard to directly convey geometric intuition in higher dimensions --- word portraits of geometric ideas can be good, but most mathematical writing neglects them.

I think the best strategy for learning is to avoid reading symbolic definitions of these little homotopies until you have spent some effort thinking about them for yourself, primarily in your head. (Sketches can be good too, but they're often another layer of difficulty. Geometric imagination is not predominantly visual; it's a learned, tricky skill to be able to draw an image on paper that adequately represents a geometric mental model.)

In my experience, the symbolic descriptions often actively interfere with geometric understanding; at first, only use them as hints, for times after you've thought hard and are stuck. It takes time and concentration to build good mental images, but geometric imagination does improve with practice, and it's worth the effort. Eventually, you learn to read the formulas and evoke the geometric images.

Answer 3

Harry, the expression "an explicit representative of the natural homotopy between the identity map and the constant map on a contractible based space" doesn't mean anything to me. Homotopies don't haven't "representatives", and a contractible space doesn't have a "natural" homotopy between the identity and a constant map. I suppose you mean that May could have completed his proof by using the existence of some homotopy, without actually naming a particular one? Or something like that.

I'd say that when I need to make a homotopy, most often I either make it by moving in straight lines or else I make it from another homotopy. How's that for a soft answer to a soft question.

Answer 4

In my experience, the vast majority of homotopies come from some combination of (1) homotopies guaranteed by cofibrations or fibrations and (2) straight-line homotopies.

For example, a standard approach to cellular approximation reduces to the case of a map from a cell to $X \cup D^n$, and then uses the linear structure in the interior of $D^n$ to make sense of straight-line homotopies.

Answer 5

@Harry Gindi: I had the same problem in the 1960s when writing the first 1968 edition of my book now Topology and Groupoids. Then I was looking at papers of Puppe, and found I had no idea how to construct some of their "diagrammatic" homotopies. with different formulae in each bit. This was part of the motivation for finding the gluing theorem for homotopy equivalences, which is now a standard result in abstract homotopy. My answer to another question illustrates how one gets by the methods there explicit homotopies.

There was the same motivation for using double groupoids in homotopy theory. Using the double groupoid defined here one can enrich the category of Hausdorff spaces over the category of these double groupoids with connection (though this has not been written up in detail) and this can be applied to construct homotopies. For some calculations in double groupoids with connections, see Chapter 6 of Nonabelian Algebraic Topology, particularly on rotations (p.169).

One can also enrich the category of filtered spaces over the symmetric monoidal closed category of crossed complexes, and this again enables calculations of homotopies. As said on p.322 of Nonabelian Algebraic Topology (pdf available), this requires further study.

I am not so sure that the work on model categories helps so directly with the calculation of homotopies, unles the cylinder object is used explicit;ly. The use of homotopies is central to Homological Perturbation Theory, and they are used in Graham Ellis' Homological Algebra Programs, in particular for constructing resolutions by induction with a contracting homotopy.

This paper uses higher homotopy groupoids to discuss Toda brackets.

My own work has largely used cubical methods as a background for conjectures and proofs, because of the ease of describing multiple compositions, and homotopies.

June, 2014 I remember a comment of Raoul Bott which I overheard at the 1958 ICM: "Grothendieck was prepared to work very hard to make things tautological!"

March 2016 The following picture

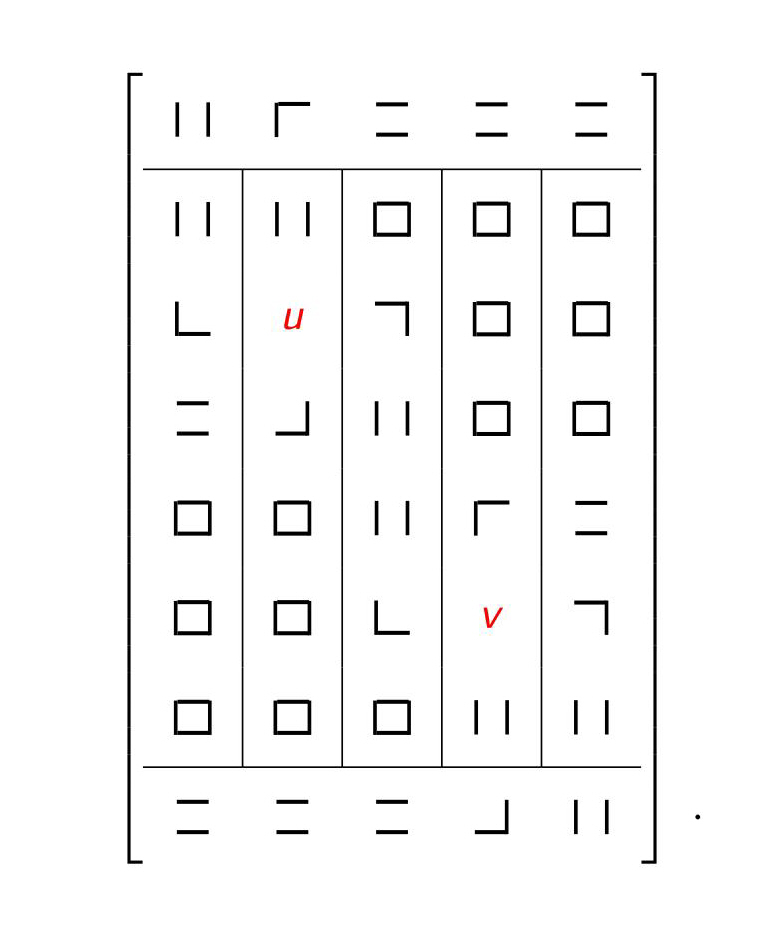
is part of the argument for proving a formula $\sigma (u +_2 v)= \sigma u +_1 \sigma v$ where $u,v$ are homotopy classes rel vertices of maps $I^2 \to X$ which take the edges to a subspace $A$ and the vertices to a set $C$ of base points, $+_1, +_2$ denote respectively compositions in the vertical and horizontal directions, and $\sigma$ is what is called a "rotation". The diagram is reinterpreted by using the interchange law and rules among the peculiar symbols called "connections". The formula implies the existence of a homotopy and in principal gives it explicitly, but that would be very difficult to write out in terms of the usual type of formulae.