6
$\begingroup$

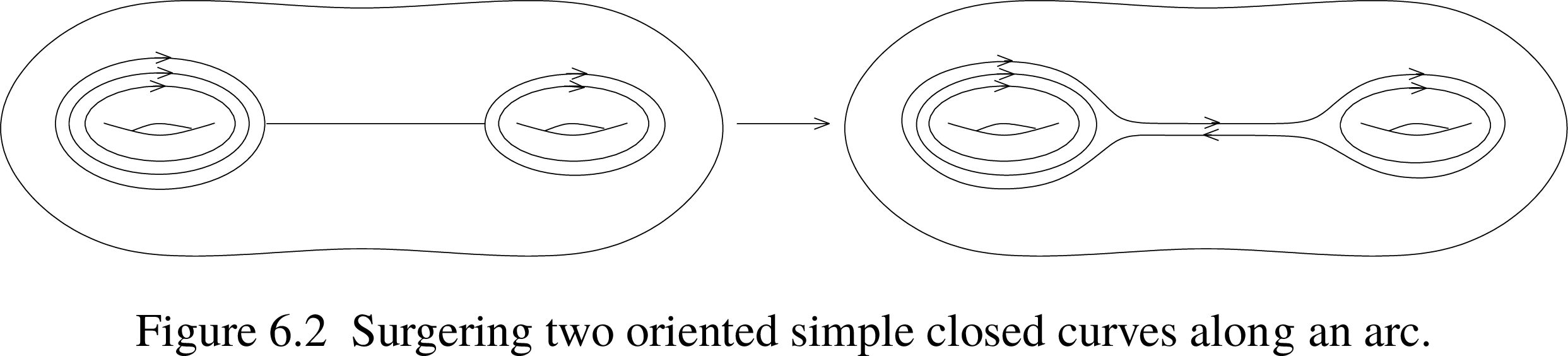
In the proof of Proposition 6.2 in Farb & Margalit, "A primer on mapping class groups", an analog of the Euclidean algorithm is used to construct a simple, closed representative (oriented) curve for a primitive element of $H_{1} (S_{g} ; \mathbb{Z})$, where $S_{g}$ is a surface of genus $g$. This was shown earlier (Theorem 1.5 ibid.) to hold for $\mathbb{T}^{2} =: S_{1}$.

For a primitive $[x] = (v_{1}, w_{1}, \ldots, v_{g}, w_{g}) \in H_{1} (S_{g} ; \mathbb{Z})$, the result for the $\mathbb{T}^{2}$ is used to write $[x] = \sum_{j = 1}^{g} \mathrm{gcd} (v_{j}, w_{j}) [\gamma_{j}]$. The proof proceeds by using a Euclidean-algorithm-type process to combine these $\mathrm{gcd} (v_{j}, w_{j}) [\gamma_{j}]$ together, starting for $j = 1, 2$ and iterating further across $j$, always obtaining homologous simple, closed curves.

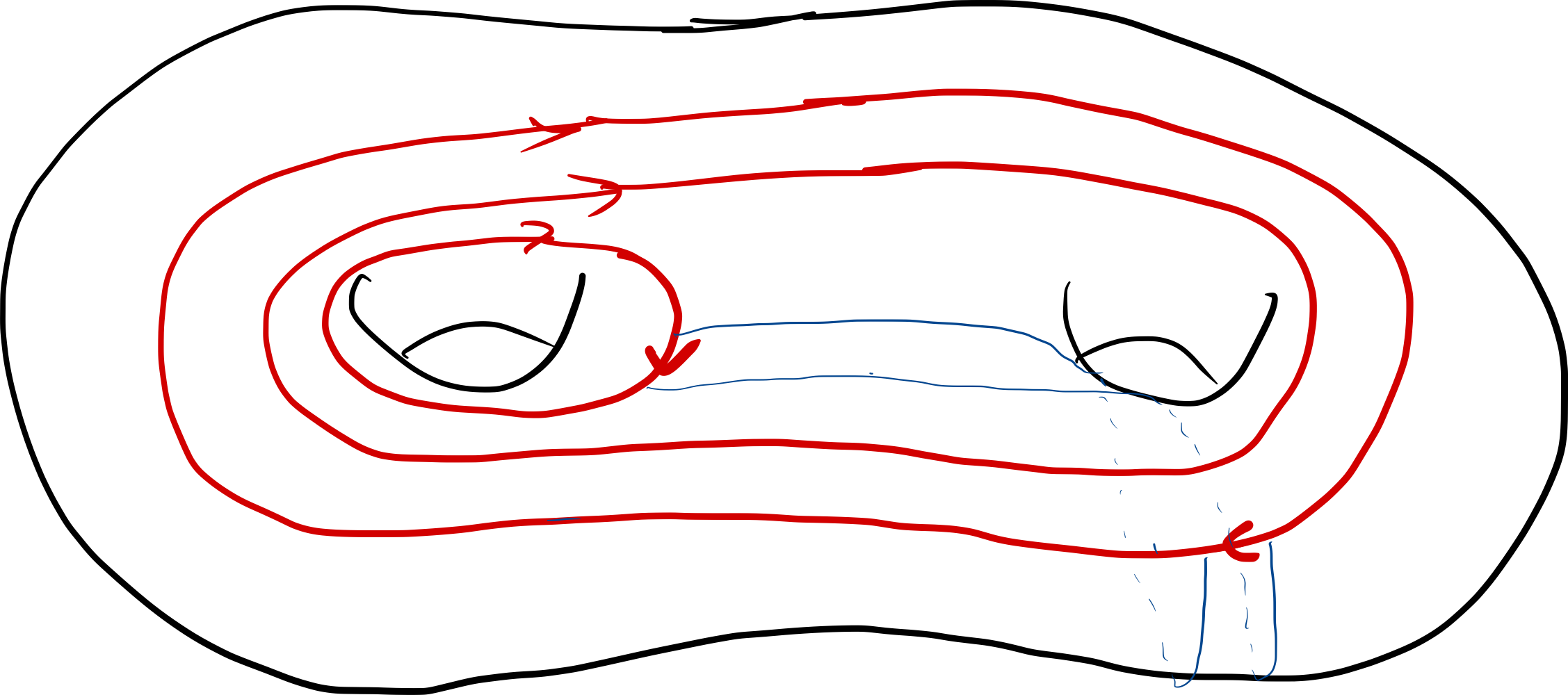
My question is about this combining process. Here is the figure from the proof in question; it shows how the first step in the process works for $g = 2$ with $[x] = 3 [\gamma_{1}] + 2 [\gamma_{2}]$, wherein one copy of $[\gamma_{1}]$ (left) is "surgered" to a copy of $[\gamma_{2}]$ (right).

enter image description here

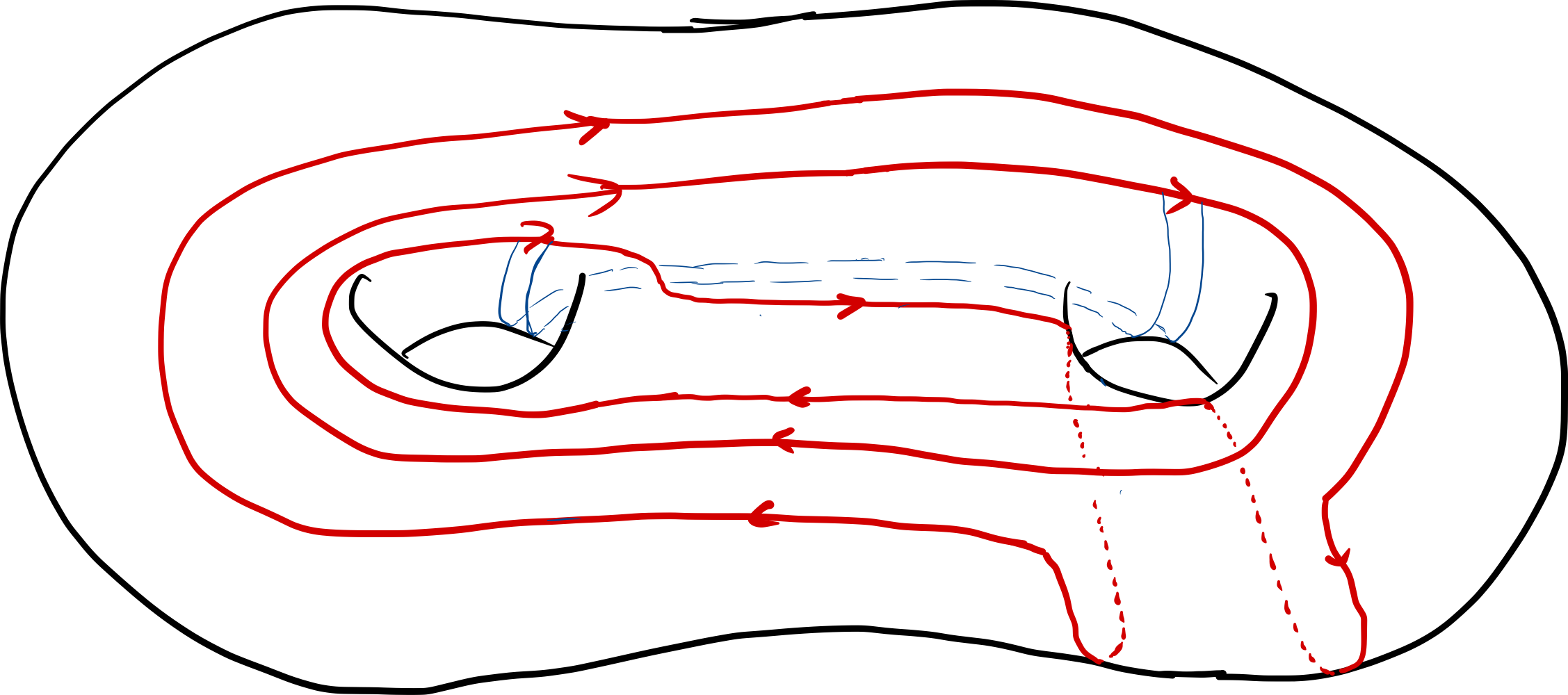
As described in the proof, this process should be repeated until there are only $\mathrm{gcd} (v_{1}, w_{1}, v_{2}, w_{2}) = \mathrm{gcd} (3 , 2) = 1$ curve(s) left. It is easy to see the at least one more "surgering" is possible of one curve on the left to one on the right, leaving a total of $3$ curves.

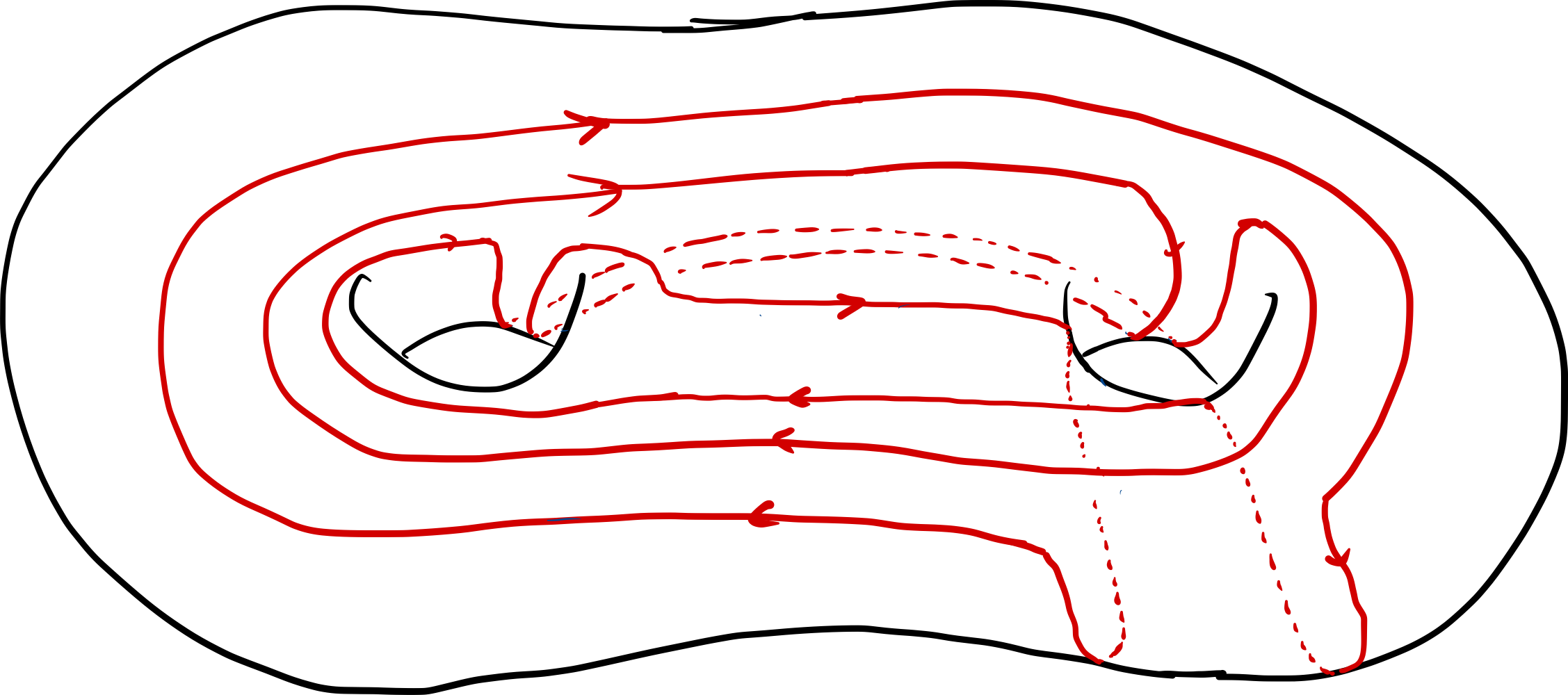
However, continuing this process does not seem so straightforward. Those $3$ curves comprise one "leftover" copy of $[\gamma_{1}]$ and the two "surgered" curves. It is possible in a roundabout way to combine that leftover curve with the outer of the two "surgered" curves, but then it does not seem possible with the two remaining curves.

How should this last simple, closed curve obtained? What is a good way to think about this process generally? References?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
5
$\begingroup$

EDITED: The figures below now give a surgery sequence starting with three curves and ending with one.

surface with three curves and one surgery arc

surface with two curves and one surgery arc

surface with one curve

Here is an interesting question for you: in general, how long must the final curve be, as a function of the original integer vector?

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Right, this is what I meant by a "roundabout way to combine that leftover curve with the outer of the two 'surgered' curves". But how does one continue then to combine the the remaining two curves, as that trick does not seem to work. Your picture still has two curves. $\endgroup$
    – MRJ
    Commented Apr 18 at 5:27
  • $\begingroup$ @MRJ: If you draw the pictures carefully, you’ll see that you can indeed find an arc that allows you to continue the process. You really might prefer the exposition linked to in my answer, which arranges things so you only need to do surgery once (though it really is the same proof). $\endgroup$
    – Andy Putman
    Commented Apr 18 at 7:41
  • 1
    $\begingroup$ @MRJ - I have now given a full surgery sequence. Sorry about misreading your question previously. $\endgroup$
    – Sam Nead
    Commented Apr 18 at 9:07
  • $\begingroup$ @SamNead Thank you! It seems that I just found the description of the process in the proof to be a bit misleading. The steps in sequence can get quite complicated it seems, not just simply "combing the leftmost copies", as they say. However, the argument does fallback onto the fact that all the curves are non-separating so it is always possible to combine the curves in this way, right? As for your interesting question: I am guessing the length of $\gamma_{j} = \frac{v_{j} + w_{j}}{\gcd (v_{j}, w_{j})}$, so the length of the final curve is perhaps at least the sum of those? $\endgroup$
    – MRJ
    Commented Apr 19 at 4:52
4
$\begingroup$

I wrote a note that explains a slightly different way to think about this proof here. This note also gives references to a what I think are the original sources for this result.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .