I have come across the following lemma in several papers (for instance see lemma 2.2 in this) (and some authors state this the proof immediately follows from basic properties of the stereographic projection).
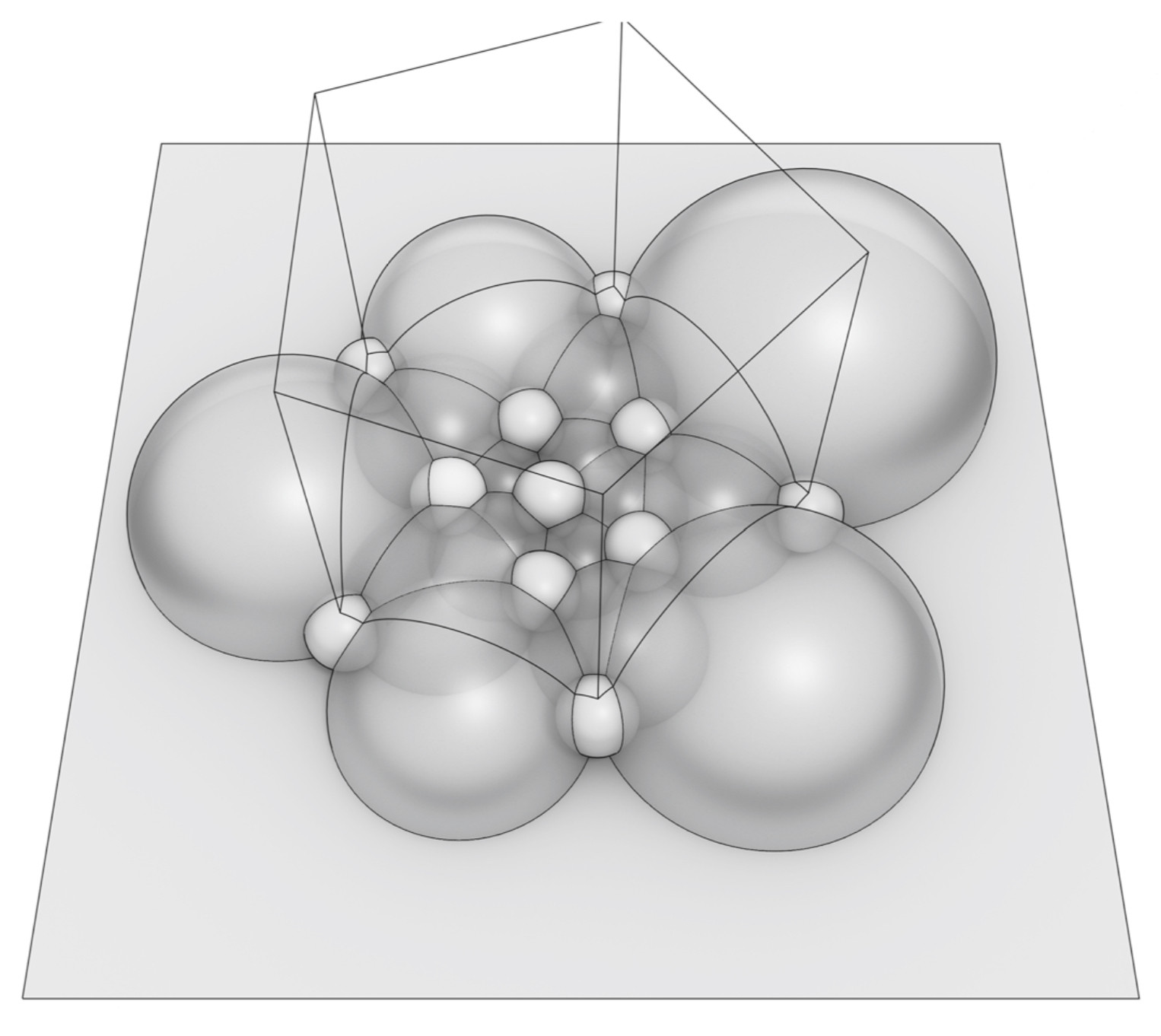
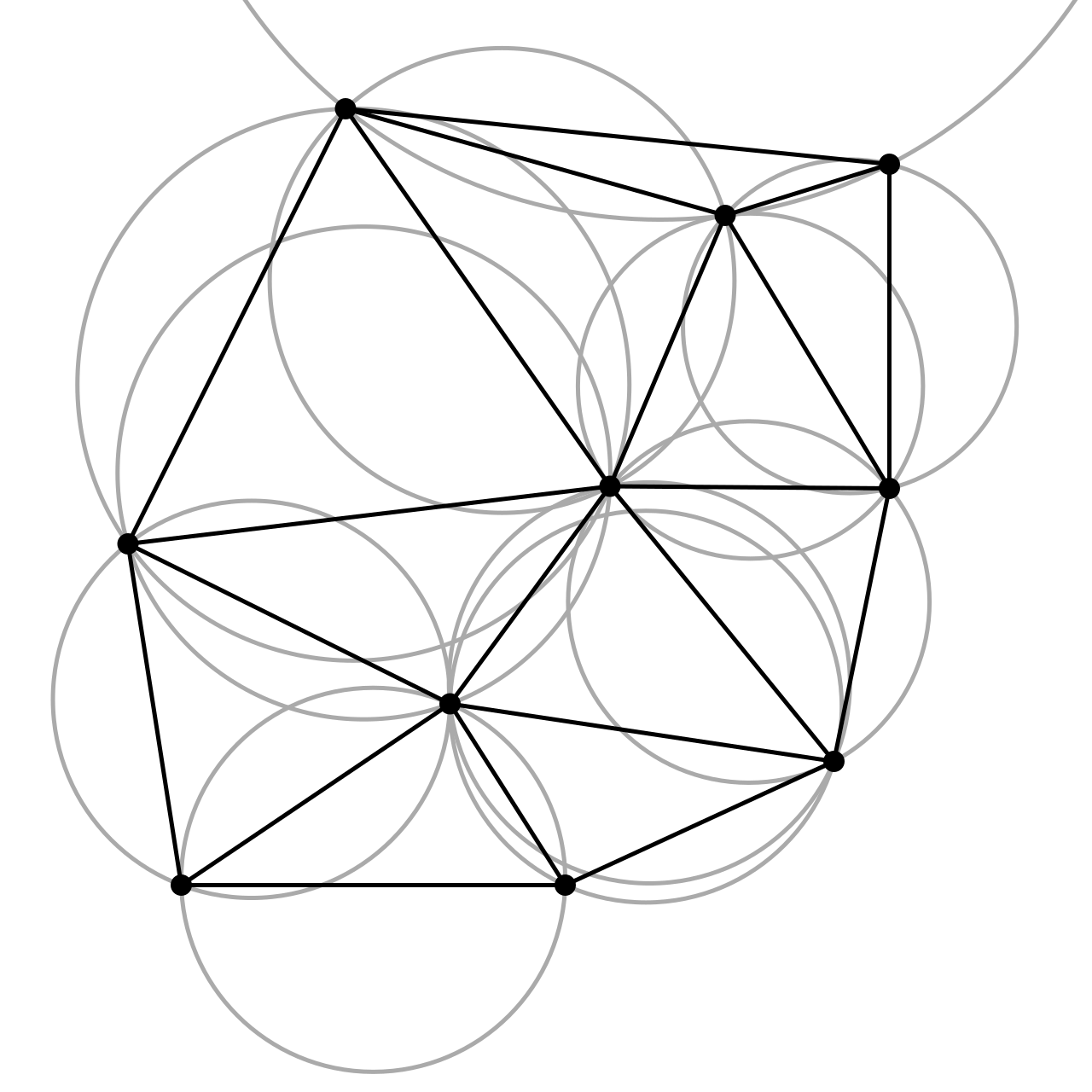
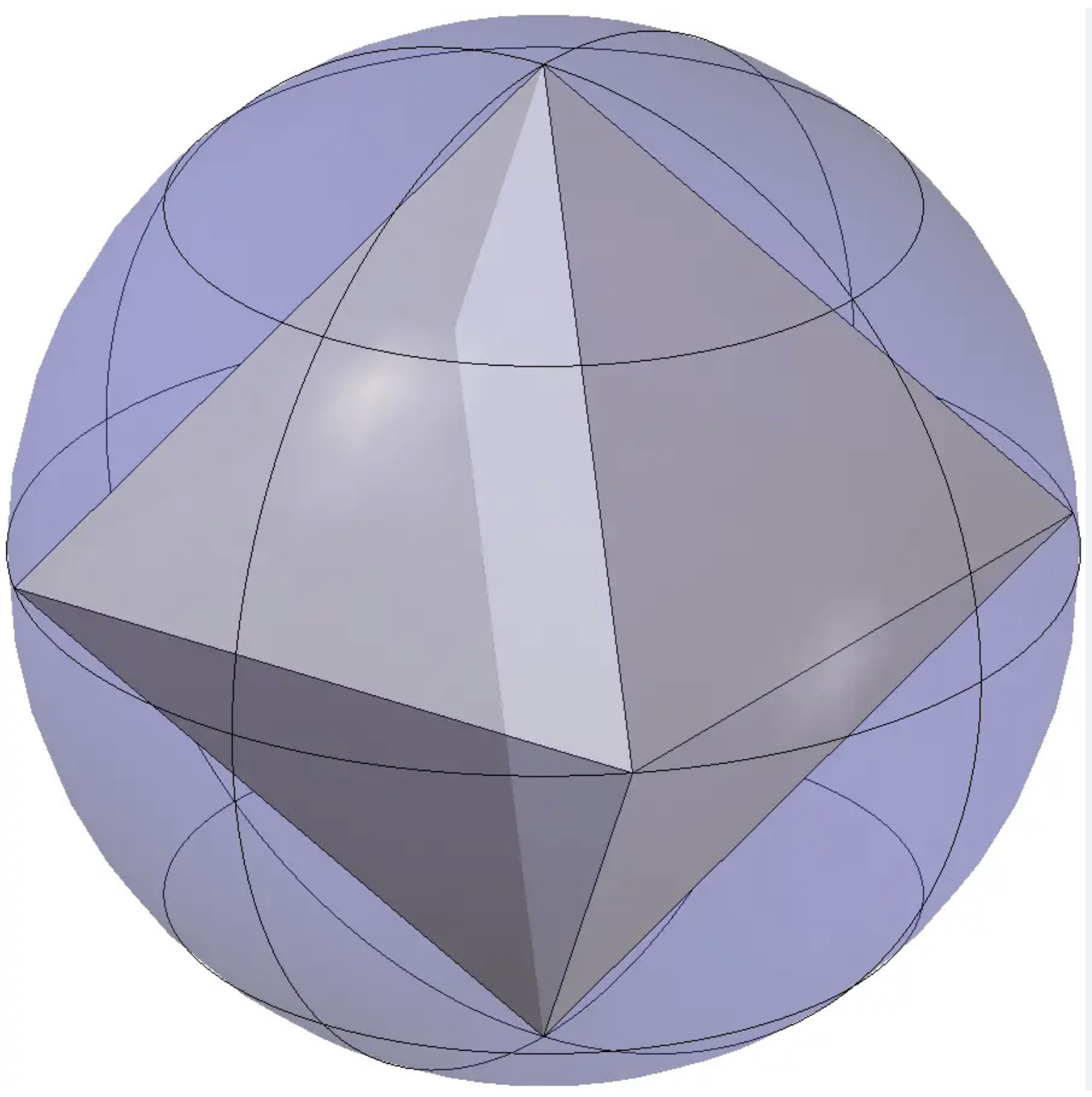
A plane graph $G$ is realizable as a Delaunay tessellation in the plane, with face $F$ as the unbounded face, if and only if the graph $G^{'}$ obtained by stellating face $F$ in $G$ is inscribable.
A graph is said to be inscribable if it is polyhedral (that is there is a set of point in $\mathbb{R}^3$ whose convex hull is combinatorially equal to the graph) and if there is a specific realization where the polyhedron is inscribed in a sphere (all points of polyhedron on a sphere).
Can someone provide a short proof or reference?
I know that if one parabolically lifts points, then the lower convex hull is combinatorically equal to the Delaunay tessellation - but I don't see why the above lemma is true, and why stellating a face is needed?