6
$\begingroup$

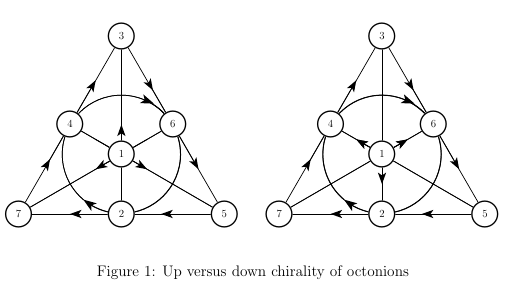
Octonion multiplication can be defined with respect to a set of triads. A set of such triads can be represented by a directed Fano plane diagram such as the following two diagrams.

Directed Fano planes with opposite chirality

This depicts two sets of triads for octonion multiplication in two distinct octonion algebras.

Each of the 480 possible sets of octonion triads can be placed in one of these directed Fano planes but not in the other. Furthermore, any set of seven distinct symbols place into one or the other of these two diagrams defines an octonion algebra.

Given that each octonion algebra is isomorphic to any other octonion algebra, can this partition into two mutually exclusive sets of octonions have any significance?

Context: I'm writing an html version of a scientific calculator for real octonions which is agnostic to whichever numbering system one chooses for the unit imaginaries. The user will initialize the calculator with their preferred set of triads. So it is necessary to check that the triads in fact define an octonion algebra. When programming the check, the issue of 'chirality' arose.

Note: Further reflection shows that 'chirality' is essentially the same issue as 'right hand system' versus 'left hand system' in coordinate systems. It is a feature of choice of unit basis elements in an octonion algebra, not a feature of the algebra itself.

Improve this question
$\endgroup$
3

2 Answers 2

Reset to default
6
$\begingroup$

Perhaps this is more a question about the Fano plane than about the (real) octonions. Notice that the automorphism group of the Fano plane is the simple group $\operatorname{GL}(3, \mathbb{F}_2) \cong \operatorname{PSL}(2, \mathbb{F}_7)$ of order $168$. The elements of order $7$ can be viewed as orientations of the Fano plane, and these elements fall into two conjugacy classes.

An explicit reference where the connection with octonions is worked out in more detail is de Traubenberg and Slupinski - Commutation relations of $\mathfrak g_2$ and the incidence geometry of the Fano plane, but I would not be surprised if this has already appeared much earlier in other sources, too.

Improve this answer
$\endgroup$
2
$\begingroup$

Negating the seven imaginary basis vectors of the octonions is equivalent to reversing every arrow in the oriented Fano plane. This operation swaps the two orientations, and displays the isomorphism between the corresponding octonion algebras.

More explicitly, in terms of your diagrams, just negating $e_1$ reverses the three arrows through $1$, and swaps your two diagrams.

So an orientation of the Fano plane corresponds to an equivalence class of chosen signs for the seven basis vectors, and an odd number of sign changes swaps the two orientations.

There is also another MathOverflow post about the same phenomenon here: What is the oriented Fano plane?

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ By the way the linked post gives a better numbering for the vertices, so that the lines are 124, 235, 346, 457, 561, 672. Then (1234567) is an automorphism, and the relations are $e_ie_{i+1}=-e_{i+1}e_i=e_{i+3}$ where the indices are taken mod 7. $\endgroup$
    – Dave Benson
    Commented Oct 5, 2023 at 7:53
  • $\begingroup$ The fact that the transformation $e_k\to e_k^*$ reverses the 'chirality' is actually what motivated me to call it 'chirality.' $\endgroup$
    – John Wayland Bales
    Commented Oct 5, 2023 at 14:54

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .