Let us consider the Riemann Zeta function non-trivial zeros $\rho_{n}=\frac{1}{2} + i\gamma_{n}$.
Let us now consider the following sum: $\tau (n)=\sum_{k=1}^{n}\sin(k)\sin(\gamma _{k})$
where:
$\gamma _{1}=14.1347251417346\ldots$
$\gamma _{2}=21.0220396387715\ldots$
$\gamma _{3}=25.0108575801456\ldots$
etc
This is the plot of $\tau (n)$ for $n$ between 1 and $10^4$:
My question is: does anyone know any articles where this periodicity is investigated?
Assume
$$N(t)=\sum_{\Im(\rho)\le t} 1\tag{1}$$
is the non-trivial zeta zero counting function which counts the number of non-trivial zeta zeros in the upper-half plane with $\Im(\rho)\le t$.
The periodicity in
$$\tau(n)=\sum_{k=1}^n \sin(k) \sin (\gamma_k)\tag{2}$$
where $\gamma_k=\Im(\rho_k)$ is probably related to the following estimate of $N(t)$:
$$\tilde{N}(t)=\frac{t}{2 \pi} \left(\log\left(\frac{t}{2 \pi}\right)-1\right)+\frac{1}{2}\, \log(2 \pi)\tag{3}$$
Consider the following approximation to $\tau(n)$
$$\tilde{\tau}(n)=\sum_{k=1}^n \sin(k) \sin (\tilde{\gamma}_k)\tag{4}$$
where $\tilde{\gamma}_k$ refers to the root of $\tilde{N}(t)-k=0$ which is an estimate of the imaginary part of the $k^{th}$ zeta zero in the upper-half plane.
The following plot illustrates $\tau(n)$ defined in formula (2) above in blue and $\tilde{\tau}(n)$ defined in formula (4) above in orange.
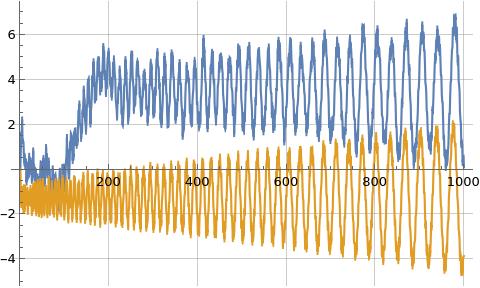
Figure (1): Illustration of formula (2) for $\tau(n)$ (blue) and formula (4) for $\tilde{\tau}(n)$ (orange)
While $\tilde{\tau}(n)$ may not be a particularly good approximation of $\tau(n)$, Figure (1) above illustrates the oscillation in $\tau(n)$ seems to be related to the approximate regularity of $\Im(\rho_k)$.
