Let $V$ be a body in $\mathbb{R}^3$ bounded by a smooth sphere with principal curvatures at most 1 (by absolute value). Is it true that $$\mathop{\rm vol} V\ge \mathop{\rm vol} B,$$ where $B$ denotes the unit ball?
Comments
I heard this question from Dima Burago.
In $\mathbb{R}^2$ an analogous statement follows from the Pestov–Ionin theorem.
One might think that $V$ contains a copy of $B$, but this is not true; an example was constructed by Lagunov (Sibirsk. Mat. Zh. 1961)
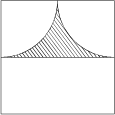
Postscript. There is a closed surface with normal curvatures at most 1 that bounds volume arbitrarily close to $\tfrac{22 }3\cdot\pi-2\cdot\pi^2\approx 3.3$. This volume is swapped by rotating the hatched figure in $2\times2$ square around its lower side.
This volume is smaller than the volume of unit ball which is $\tfrac43\cdot\pi\approx 4.2$.
However, the surface is homeomorphic to the sphere with two handles. (It is an optimized version of one of Lagunov's examples.) I tried to optimize the spherical example and was not able to get below $\tfrac43\cdot\pi$. Maybe one can prove that it is impossible, but most likely it is going to be tedious.