Let $\Delta_x:\ f(x)\rightarrow f(x+1)-f(x)$ be the forward difference operator then $\mathscr{A}=2I+\Delta_x$ where $I$ is the identity operator. Using formal expansion of operators $$\mathscr{A}^{-1}=(2I+\Delta_x)^{-1}=\frac{1}{2}\sum_{n=0}^\infty(−\frac{1}{2})^n\Delta_x^n$$ where $\Delta_x^0=I$ and $\Delta_x^n=\Delta_x[\Delta_x^{n-1}]$, $n\in\mathbb N$. By expanding $\Delta_x^n[f(x)]$ as $$ \Delta_x^n[f(x)]=\sum_{k=0}^n\binom{n}{k}(-1)^{n-k}f(x+k)$$ we get by replacing $$\mathscr{A}^{-1}[f(x)]=\sum_{n=0}^\infty\frac{1}{2^{n+1}}\cdot\sum_{k=0}^n\binom{n}{k}(-1)^kf(x+k)$$ $$\mathscr{A}^{-1}[f(x)]=\sum_{k=0}^\infty(-1)^kf(x+k)\cdot\sum_{n=k}^\infty\binom{n}{k}\frac{1}{2^{n+1}}$$ Since $\Delta_x^n$ annihilates polynomials $p_\ell(x)$ of degree $\ell$ lower than $n$, i.e. $\Delta_x^n[p_\ell(x)]=0$ for $0\le\ell<n$, we get finite sums' upper limits by taking $f(x)=p_\ell(x)$. In fact $$\mathscr{A}^{-1}[p_\ell(x)]=\frac{1}{2}\sum_{n=0}^\ell(−\frac{1}{2})^n\Delta_x^n[p_\ell(x)]$$ $$\mathscr{A}^{-1}[p_\ell(x)]=\sum_{k=0}^\ell(-1)^k\,p_\ell(x+k)\cdot\sum_{n=k}^\ell\binom{n}{k}\frac{1}{2^{n+1}}$$ I think this is the formula you are looking for. Indeed, for single powers $p_\ell(x)=x^\ell$ we obtain using the following PARI/GP code
g(L,x='x)=for(l=0,L,print("p(",l,",x) = ",sum(k=0,l,(-1)^k*(x+k)^l*sum(n=k,l,binomial(n,k)/2^(n+1)))))
this output
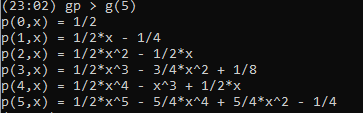
NOTE:
If $D_x: f(x)\rightarrow f'(x)$ @loup_blanc answer can be set under the same context. In this case, by using $\tanh(z)$ Taylor's series the following formal odd order differential operator expansion is obtained $$\mathscr{A}^{-1}=(I+e^{D_x})^{-1}=\frac{1}{2}I-\sum_{n=1}^\infty\frac{(4^n-1)\,B_{2n}}{(2n)!}\cdot D_x^{2n-1}$$ where $B_{2n}$ are Bernoulli numbers. For polynomials $p_\ell(x)$ we have $$\mathscr{A}^{-1}[p_\ell(x)]=\frac{1}{2}p_\ell(x)-\sum_{n=1}^{\lfloor\frac{\ell+1}{2}\rfloor}\frac{(4^n-1)\,B_{2n}}{(2n)!}\cdot D_x^{2n-1}[p_\ell(x)]$$ For single powers $p_\ell(x)=x^\ell$ we obtain by using the following PARI/GP code
f(L,x='x)=for(l=0,L,print("p(",l,",x) = ",x^l/2-sum(n=1,(l+1)\2,(4^n-1)/(2*n)!*bernfrac(2*n)*derivn(x^l,2*n-1))))
the same output that has been got from difference operators.
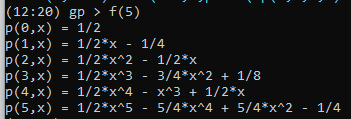

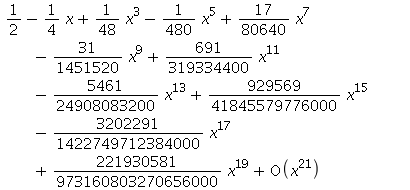


\operatorname{char}\mathbb{F}, not $\mathrm{char}\mathbb{F}$\mathrm{char}\mathbb{F}; note the difference in spacing. I have edited accordingly. $\endgroup$