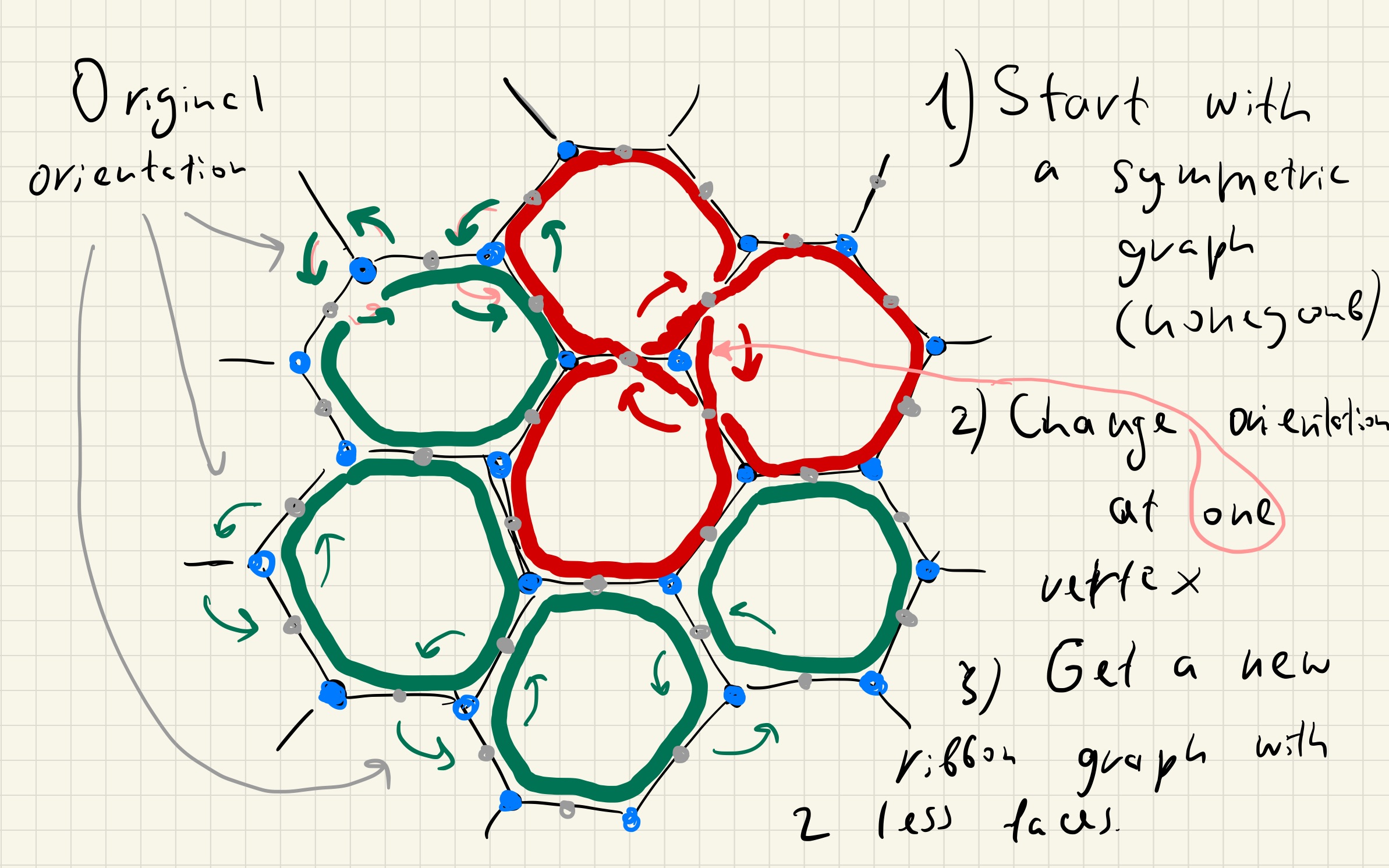
We consider a dessin d'enfant $D$ as a bipartite graph $D$ on a complex oriented surface $S$, such that the complement $S \backslash D$ is homotopic to a collection of disks.
Definition: Let an automorphism of $D$ be an orientation preserving automorphism of $S$ taking $D$ to itself.
Here is my general question: Given an arbitrary genus surface $S$, and a positive integer $k$, can we construct a a dessin $D$ on that surface whose automorphisms are a cyclic group, i.e., $\text{Aut}(D) \simeq C_{k}$?
Remark: We do not require that the dessin be regular, in fact, we don't expect it to be. For example, the genus 1 example below is not a regular dessin (i.e. isomorphic to a complete bipartite graph). [See page 6 of this paper of Jones which addresses genuses possible in the regular case.]
Motivating Examples:
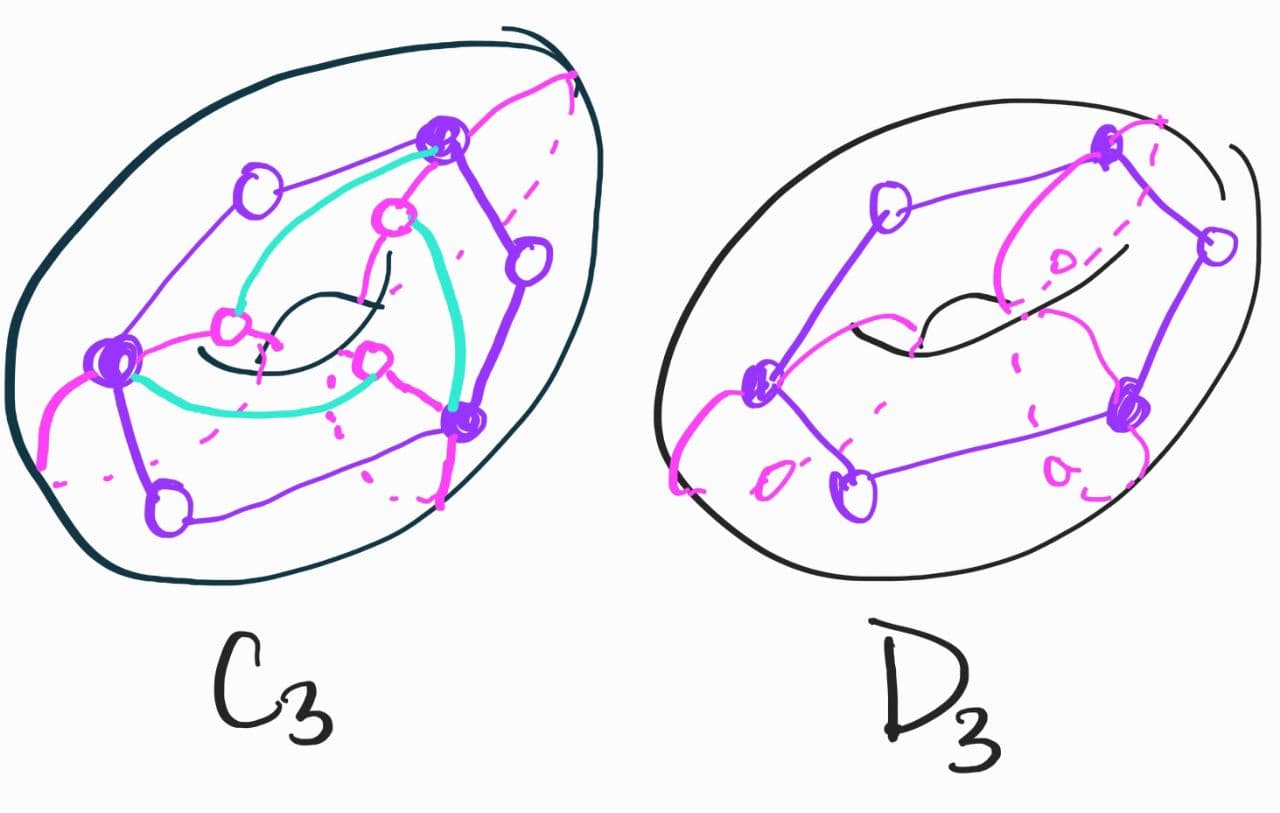
Let's stick to the examples of cyclic group $C_3$ and the dihedral group $D_3$ for simplicity. In the genus 0 and 1 cases, the $C_3$ and $D_3$ cases immediately generalize to all $C_k$ and $D_k$.
In the genus 0 case, they look like this, note that the $C_2$ action in the dihedral group comes from switching the inner and outer faces (indicated by the red arrow). 
In the genus 1 case, they look like the following picture. [Remark: the $C_2$ automorphism in $D_3$ comes by moving the purple disk out and down and then in and up to where it was, and rotating the pink loops inwards back to where it was, this swaps the faces. (The teal lines prevent this map, thus giving us $C_3$.)]
In the genus 2 case, I can only manage to create with automorphism group $C_2$ and $C_2 \times C_2$ respectively. They look like the following. 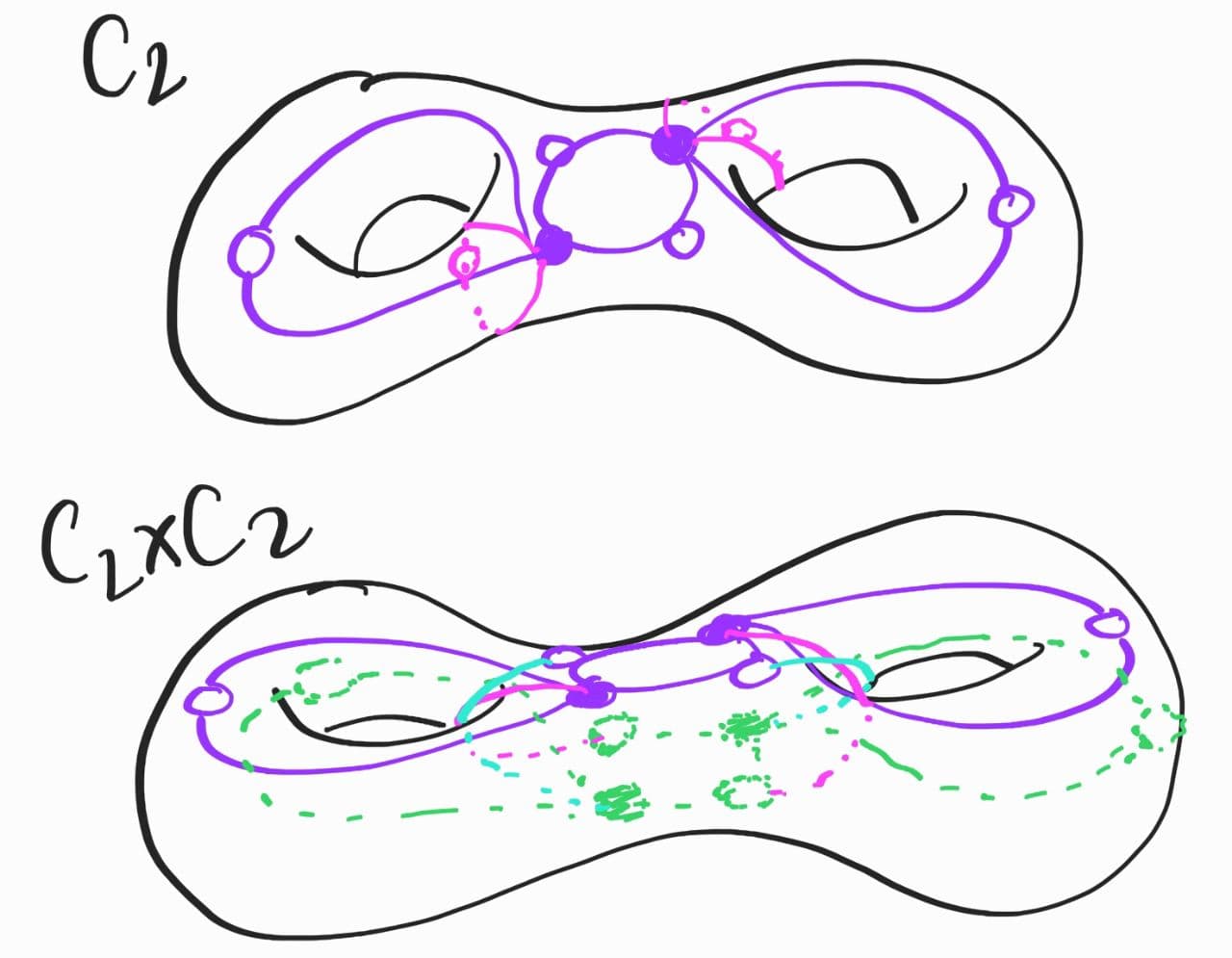 No matter how many loops I add, I haven't managed to get an automorphism group larger than this on a dessin on a surface of genus 2. Similarly, I don't see render a dessin with automorphism group $C_{k+i}$ on a surface of genus $k$ in general. Hopefully this is due to my own incompetance because it would be really cool to see what it would look like! I am quite stuck. Any and all help is deeply appreciated.
No matter how many loops I add, I haven't managed to get an automorphism group larger than this on a dessin on a surface of genus 2. Similarly, I don't see render a dessin with automorphism group $C_{k+i}$ on a surface of genus $k$ in general. Hopefully this is due to my own incompetance because it would be really cool to see what it would look like! I am quite stuck. Any and all help is deeply appreciated.