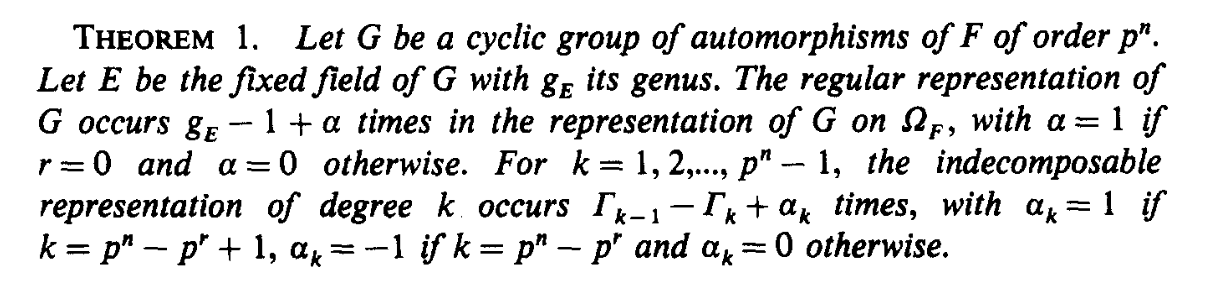I am attempting to decompose the holomorphic differentials of an Artin-Schreier-Witt curve as a $\mathbb{Z}/p^n$-representation. This is done in Theorem 1 of Madan-Valentini Automorphisms and holomorphic differentials in characteristic p. However, I seem to be unable to understand their result even in the simplest case ($n=1, p=3$).
First, I will define the quantities that show up in their theorem.
$F$ is the function field of the curve with affine model $y^3-y = x^2$. $E$ is $K(x)$ the function field of $P^1$. They use the notation $\Omega_F:= H^0(C, \Omega_C)$.
In our example, we have just one ramified prime $P'$ (the elliptic curves point at infinity) which is totally ramified over the point $P$ (infinity on the projective line).
In our case, $r = 0$. [[This is because $r := 1 - max(e_i)$, where $e_i$ is the ramification index of all primes $P_i$ in $F$ which ramify over $P$ in $E$, and $e_i = 1$, thus, $r = 0$.]]
Further, $\Gamma_k := v_{ik} := \lfloor \frac{d(P'|P) + kv_{P'}(x^2)}{p} \rfloor$, where $d(P'|P)$ is the exponent of the different of the point $P'$ over the point $P$.
Their theorem is as follows:
What I know is true:
In our example, there is only one degree 1 component when considering $H^0(C, \Omega_C)$ as a $Z/3$-representation, and no other components. This degree 1 component is generated by $dy/x$. As a sanity check, we know apriori that the holomorphic differentials of C coincide with the cotangent space of the Jacobian at its origin, that is $H^0(C, \Omega_C) \simeq T^*_e(Jac(C))$, so it should indeed be 1-dimensional since $Jac(C)$ of an elliptic curve $C$ is 1-dimensional.
Madan-Valentini result:
The two values that go into the definition of $\Gamma_{k+1} := v_{1k}$ in Theorem 1 are (1) $d(P'|P)$ the exponent of the different of the point $P$ at infinity in $K(x)$ wrt the point $P'$ at infinity in the elliptic curve, and (2) the valuation of $x^2$ at the point $P'$ in the elliptic curve totally ramified above infinity.
I calculated that (1) is 6, and (2) is 6 also.
Plugging in my values of (1) and (2), I get $v_{11} = 0$ and, $v_{12} = -2$.
But!! But, having only 1 degree one component in the representation forces the values of $\Gamma_0 := v_{11}$ and $\Gamma_1 := v_{12}$ in their theorem to have the relation $v_{11} = v_{12} + 1$. I can't find my error, however! It's driving me up the wall!
Here's my question: What have I done wrong? Why am I getting a contradiction?
I will explain how I calculated (1) and (2), I hope that someone can spot an error. The method of calculating (2) was explained to me by Jora Belousov, and all errors are mine and mine alone.
(1) calculation of the exponent of the different $d(P'|P)$
The only ramification point is at infinity which I'll call $P := (1/x)$. We consider a valuation $v_P$ on $K(x)$. If the $x$-valuation of $w \in K(x)$ is nonpositive, then $v_P(x^2 - (w^p-w)) = v_P(x^2) = -2$. If the $x$-valuation of $w$ is positive, then $v_P (x^2 - (w^p-w)) = v_P(w^p) = p v_P (w)$ which is negative and less than $-p$, so the answer is $-2$ and it is well-defined. Thus, we have that our jump at $P$ is $m_P = 2$.
Here I am using Stichtenoth, Algebraic Function Fields and Codes, Thm 3.7.8. We may then immediately calculate the exponent of the different $d(P'|P) = (p-1)(m_P + 1)$, i.e., 2*3 = 6.
(2) calculation of $v_{P'}(x^2)$ (which is called $-\Phi(1, 1)$ in Valentini-Madan)
Let $v_{P'}$ be the valuation extending the valuation at infinity of the projective line, which we've called $v_P$.
First, we homogenize the equation $x^2 = y^3 - y$:
and get $x^2z = y^3 - yz^2$.
Then, we go to the chart $x = 1$, in this chart, the function $f=x^2$ becomes $f = 1/Z^2$. Here, our equation becomes $Z = Y^3-YZ^2$ which implies $$Z = Y^3/(YZ + 1).$$
We now localize the coordinate ring $K[Y,Z]/(Y^3 - YZ^2 - Z)$ at the point $(Y = 0, Z=0)$, let's call that ring $A_P$. Since $(YZ+1)$ doesn't vanish at this point, it is a unit in $A_P$. Thus, $Z$ is contained in the ideal generated by $Y$. Because the maximal ideal of the local ring $A_P$ is the principal ideal of $Y$, the valuation that we are interested in can be computed as the $Y$-degree: $v_(Y,Z) = v_Y$. Again using the elliptic curve equation, we may express our original $f = x^2$ in our new coordinates as $f = (1/Z)^2 = (YZ+1)^2/Y^6$.
Thus, $v_{P'}(x^2) = v_{(Y,Z)}(Z^{-2}) = v_{Y} (Y^{-6}) = -6$.