Katz's paper on $p$-curvature – help with proof understanding

Question

I am studying N. Katz's paper "Nilpotent connections and the monodromy theorem: applications of a result of Turrittin" where I found a fairly good account on $p$-curvatures.

I don't understand the following proof:

---

---

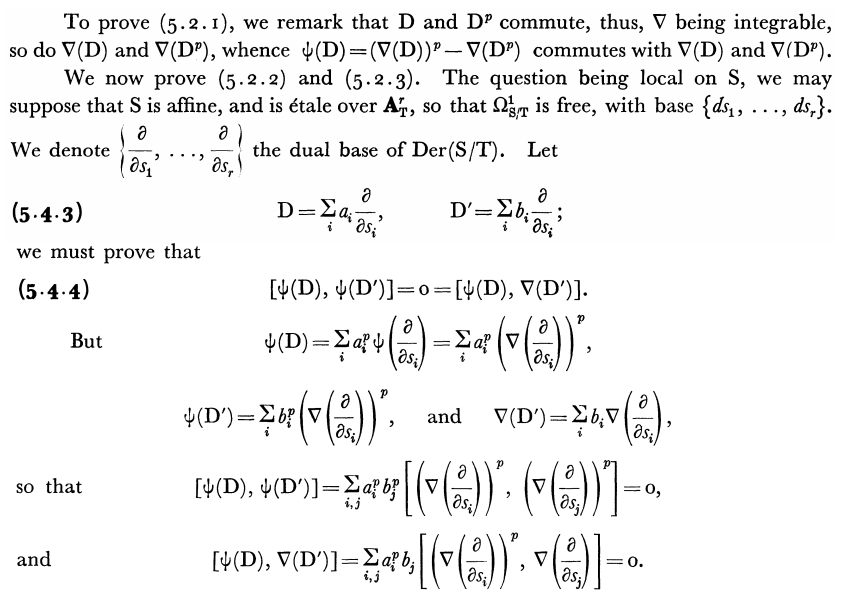
Let :

$\Psi: \operatorname{Der}(S|T) \to \operatorname{End}_T(\mathcal E)$

$D \to (\nabla(D))^p -\nabla(D^p)$

Where $\nabla: \operatorname{Der}(S|T) \to \operatorname{End}_T(\mathcal E)$ such that: $\nabla(D)(ge) = D(g)e+g\nabla(D)(e)$, $e$, $g$ and $D$ sections of $\mathcal E$, $\mathcal O_S$ and $\operatorname{Der}(S|T)$ respectively and $\mathcal E$ is a vector bundle on $S$.

1. To prove $(5.4.4)$, we have by $p$-linearity and additivity of the p-curvature:

   $$\Psi(D)=\sum_i a_i^p \Psi\big( \frac{\partial}{\partial s_i}\big) = \sum_i a_i^p \Big(\nabla\big(\frac{\partial}{\partial^p s_i}\big)\Big)^p -\sum_i a_i^p \nabla\big(\frac{\partial^p}{\partial s_i^p}\big)$$ but the term $\sum_i a_i^p \nabla\big(\frac{\partial^p}{\partial s_i^p}\big)$ disappears in the proof and I don't see why?

2. At the end of the proof, it looks like we use the fact that $\frac{\partial}{\partial s_i}$ and $\frac{\partial}{\partial s_j}$ commute, but why is that true?

Answer 1

1. The term in question vanishes because the derivation $(\partial/\partial s_i)^p$ is zero, and hence $\nabla((\partial/\partial s_i)^p) = 0$. This is because, in characteristic $p$, $(\partial/\partial x)^p(x^n) = 0$ for all $n \in \mathbb{N}$.

2. These derivations do commute, because $\partial/\partial s_i$ and $\partial/\partial s_j$ commute on polynomials $k[s_1,\ldots,s_r]$.