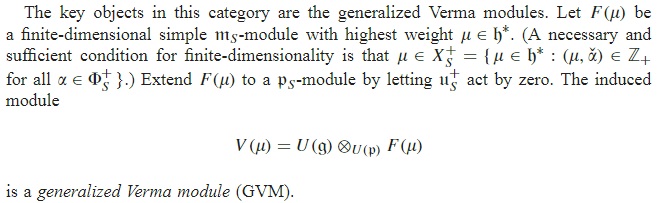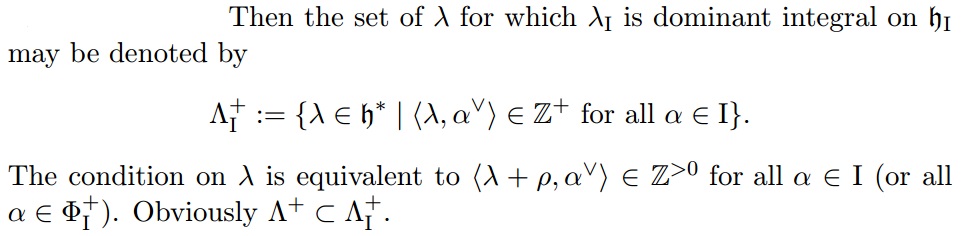I am reading this paper: Representation type of the blocks of category $\mathcal{O}_S$
While on p. 183 (Section 9.2) of Representations of Semisimple Lie Algebras in the BGG Category $\mathcal{O}^\mathfrak{p}$. Humphreys said that
Let $\Lambda_I^+:=\{\lambda\in\mathfrak{h}^*: \langle\lambda,\alpha^\lor\rangle\in\mathbb{Z}^{\ge 0} \ \forall \alpha\in I\}$ and $X_I^+:=\{\lambda\in\mathfrak{h}^*: \langle\lambda,\alpha^\lor\rangle\in\mathbb{Z}^{\ge 0} \ \forall \alpha\in\Phi_I^+\}$.
Obviously, they are talking about the same set. It is obviously that $X_I^+\subset \Lambda_I^+$.
But how to prove the other containment?
I think it is not trivial to see if $\alpha,\beta\in I$ such that $\alpha+\beta\in\Phi_I$, then $\dfrac{2\langle\lambda,\alpha+\beta\rangle}{\langle\alpha,\alpha\rangle+2\langle\alpha,\beta\rangle+\langle\beta,\beta\rangle}\in\mathbb{Z}^{\ge 0}$ for $\lambda\in\Lambda_I^+$.