A century ago, Dudeney asked to place $16$ pawns on a chessboard with no three on a line:
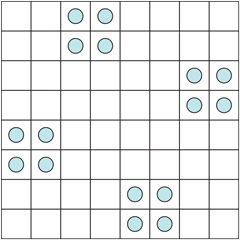
As described by David Eppstein,1 the maximum number $g_3(n)$ points that can be placed on an $n \times n$ integer grid, avoiding three points on a line, satisfies $$ \tfrac{3}{2} n - o(n) \le g_3(n) \le 2 n \;.$$
My question is:
Q. What are bounds on $g_4(n)$, the maximum number of points that can be placed on an $n \times n$ integer grid, no three on a line, no four cocircular?
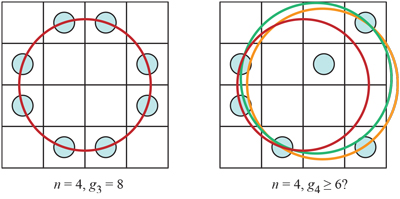
Perhaps no $4$ of the $6$ points (right figure) are cocircular?
Added: @AaronMeyerowitz shows that in fact $4$ points are cocircular.
The question can be generalized to avoiding points lying on an algebraic curve of degree $d$.
1 Eppstein, David. Forbidden Configurations in Discrete Geometry. Cambridge University Press, 2018. Chapter 9, pages 72–75. Cambridge link.
