5
$\begingroup$

Please pardon my ignorance on the subject of open books, I'm a noob. I would like to know some explicit descriptions of open book decompositions of the three torus $T^3$. Are there examples with connected binding?

Is there a direct topological proof that shows that every 3-manifold admits an open book decomposition? Ideally, such a proof might start with a Heegaard decomposition or a surgery description of a given 3-manifold, and then proceed to give an algorithm for converting this to an open book decomposition. In such a case, I suppose I could then follow such a proof to answer my first question regarding $T^3$.

Improve this question
$\endgroup$
3
  • 5
    $\begingroup$ Patrick Massot has some amazing videos of open books of $T^3$ on his webpage: math.u-psud.fr/~pmassot/en/exposition/… For the other questions I would suggest Entnyre's notes on open books. $\endgroup$
    – Marc Kegel
    Commented Oct 9, 2018 at 10:33
  • 1
    $\begingroup$ For an explicit example of an OB on $T^3$, you could take as page $\Sigma_{g=1,b=2}$ and monodromy $\tau_1^{-1}\tau_2$, where $\tau_i$ is a boundary Dehn twist for the two boundary components. Any open book can be stabilized: this yields one with $\Sigma_{g=2,b=1}$, hence with connected binding. $\endgroup$
    – KSackel
    Commented Oct 10, 2018 at 17:43
  • $\begingroup$ Ken Baker also has some nice visualizations of an open book on $T^3$. sketchesoftopology.wordpress.com/2008/11/24/the-jvhm-open-book $\endgroup$
    – Ian Agol
    Commented Oct 15, 2018 at 20:58

2 Answers 2

Reset to default
6
$\begingroup$

There is a direct proof of exactly what you ask for on page 147 of:

Calegari, Danny, Foliations and the geometry of 3-manifolds, Oxford Mathematical Monographs; Oxford Science Publications. Oxford: Oxford University Press (ISBN 978-0-19-857008-0/hbk). xiv, 363 p. (2007). ZBL1118.57002.

Here Calegari provides the details of a well-known construction. Starting from the Heegaard splitting data of a fixed manifold $M$. He explains how to build a fibered link complement $M-L$. Here $L$ is the complement of the set of curves used in Lickorish's theorem (showing every manifold is Dehn surgery on a link) plus one more 'unknotted' curve.

Calegari then answers your second question using the well-known technique of "plumbing along a Hopf band" find a new fibered link complement $M-L_1$ in $M$ with 1 fewer cusp than $M-L$. This comes at the expense of raising the genus of the fiber, but can be repeated until $M-L_n$ has just one cusp. Of course, this corresponds to an open book decomposition of $M$ with a connected binding.

If you are only interested in the case of $T^3$, you can start with the Borromean Rings (pictured here)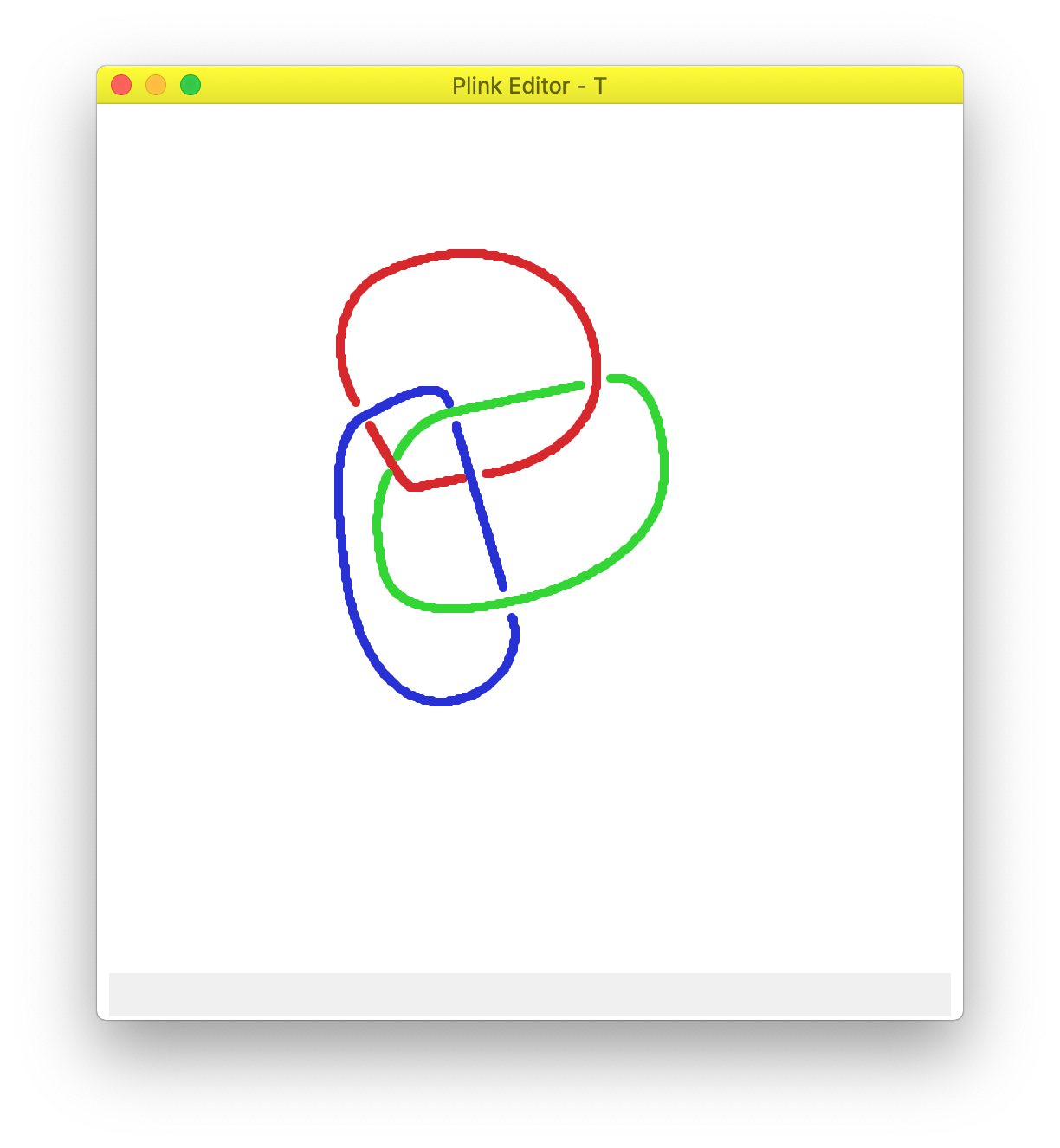Borromean rings as your fibered link (see Rolfsen's Knots and Link 338 for info on a fibration of this link complement). (0,1) surgery on each component yields $T^3$ and run Calegari's argument or use one of the two examples in the comments.

Improve this answer
$\endgroup$
4
$\begingroup$

As mentioned in a comment, Ken Baker has a nice visualization of an open book decomposition of $T^3$ due to Jeremy Van-Horn Morris.

enter image description here

One way to get open book structures is to start with a fibered universal knot or link, and then pull back the open book structure from $S^3$ to a branched cover which is branched over that link. The Whitehead link, the Borromean rings, and the figure 8 knot are all fibered links that are known to be universal.

In fact, every open book decomposition of a 3-manifold pulls back as a branched cover of $S^3$ of the standard open book decomposition of the unknot.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .