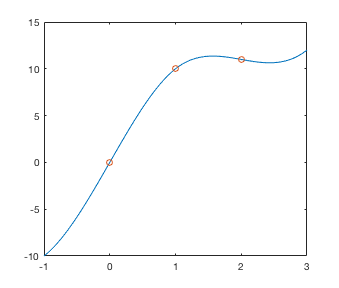
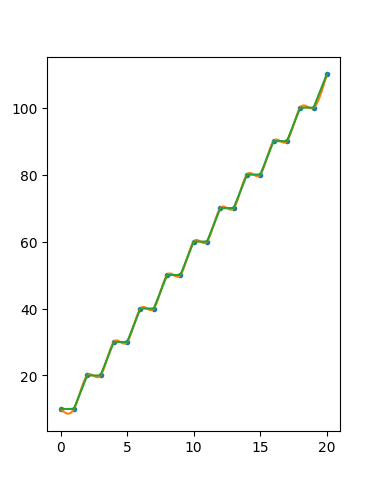
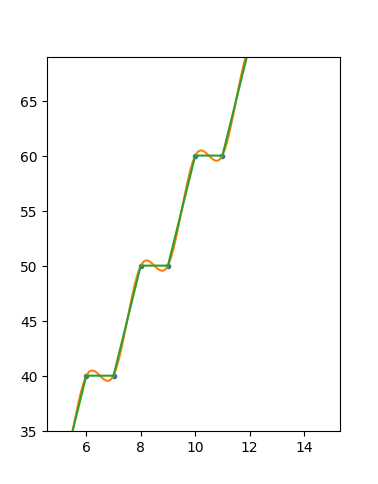
Start with a monotone nonincreasing function and sample it at finite set of points $x_0, ..., x_n$, $x_i<x_{i+1}$ so that $f(x_i)<f(x_{i+1})$. If you approximate $f$ with a linear spline then the resulting piecewise-linear approximation will certainly preserve monotonicity.
The question is: if you approximate $f$ with natural cubic spline will monotonicity be preserved as well?