Transport tubes in a sphere

Question

Let $S$ be a unit-radius sphere in $\mathbb{R}^3$.

Q0. Where should one place $3$ disjoint lines intersecting $S$ to minimize the maximum distance between any two points in $S$, where distance is measured as follows. Distance off the lines is Euclidean distance, but the distance between any two points on one line is zero.

The lines are like very fast transportation tubes. (I have in mind Star Wars' Coruscant.)

With no tubes, the maximum distance is $2$, realized by any antipodal pair. One tube, or two tubes, do not help reduce the maximum, but three tubes seem to help. In particular, I believe if one selects the three tubes to be the $x,y,z$ axes, slightly displaced to satisfy disjointness, then the maximum distance is $2 \sqrt{\frac{2}{3}} + \epsilon \approx 1.63$, illustrated below.

Q1. Is there a better arrangement of the three tubes?

---

         
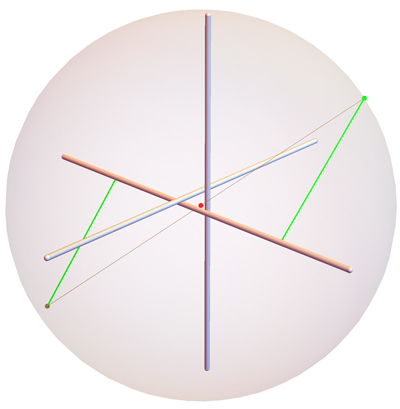
          ^{The red point is the origin/center of the sphere. The point $(1,1,1)/\sqrt{3}$ is $\sqrt{\frac{2}{3}}$ (green) from each axis.}

---

The natural generalization is: $d$ tubes intersecting a unit-radius sphere in $\mathbb{R}^d$.

Answer 1

Thanks to Ilya Bogdanov, I have faced this problem more squarely. He (gender presumption) had me convinced that my cylinder reformulation was not equivalent. It may not be, but I think it helps solve the posted problem.

Note that the metric stated in the problem always involves the distance from a point on the sphere to a tube. Two points which normally might be close on the sphere will be about distance 2R apart, where R approximates the distance from either point to the nearest tube.

An earlier non-visible attempt at this analysis considered the distances between tubes, and attempted to account for the differences. However, for most configurations of tubes, we can dispense with these transfer point distances by observing that there are 2^d many points on the d sphere that are equidistant from all (also assuming a sufficiently small tube radius) tubes. (One can find tube configurations where this is not true, however I conjecture that none of these provide an optimal arrangement.) Then there is a pair of points which is at least (and in fact exactly) R+S apart, where I pick R and S as the two largest of these 2^d equidistances.

I now conjecture that for any configuration that has these distances, an optimal configuration has R=S. We now have moved into covering the sphere by cylinders of constant radius. At this writing, I leave the numerics to others, as well as resolving the conjectures.

Gerhard "Has No Computer Geometry System" Paseman, 2017.09.21.