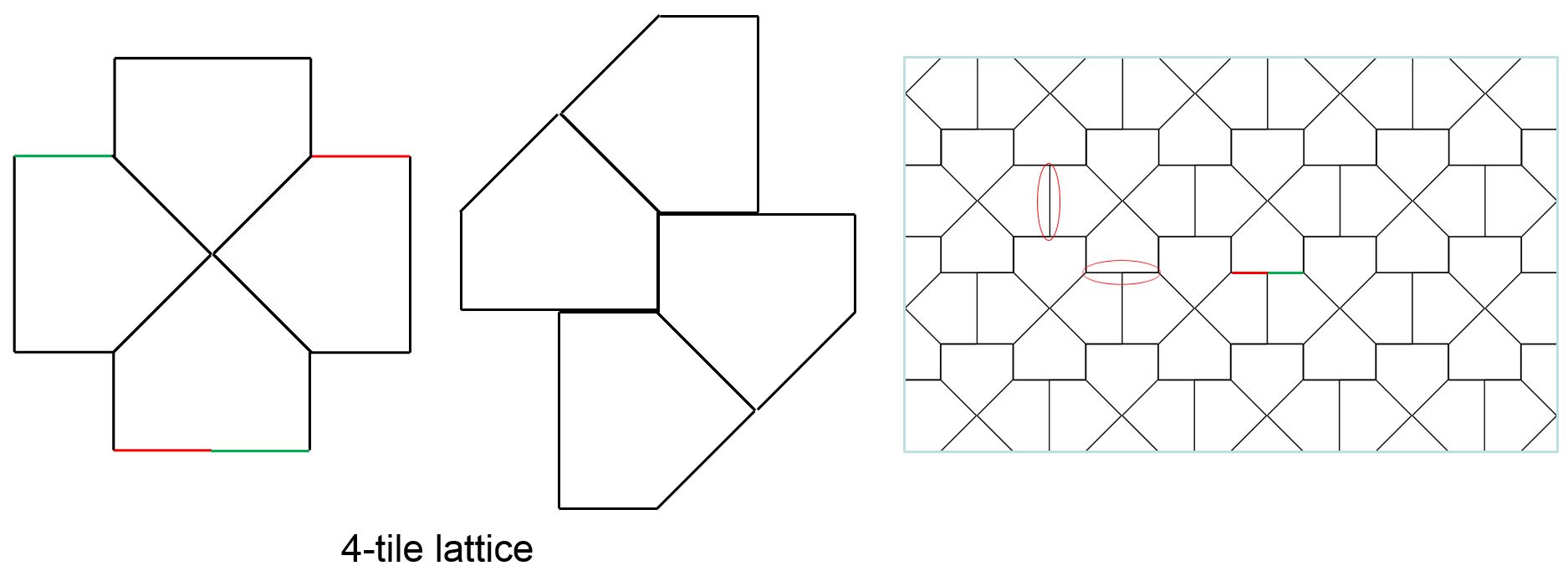
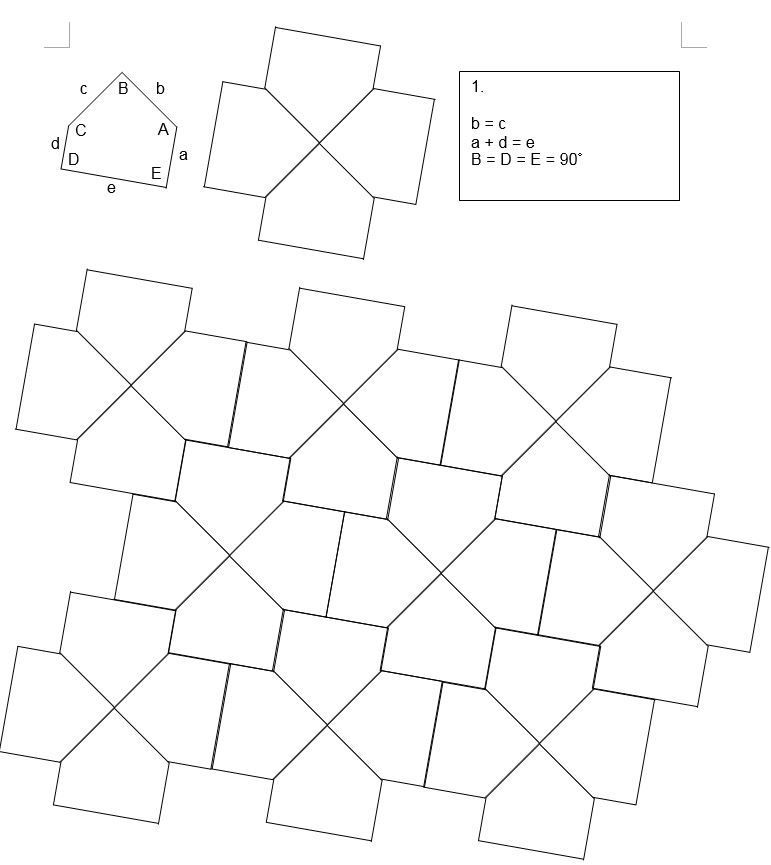
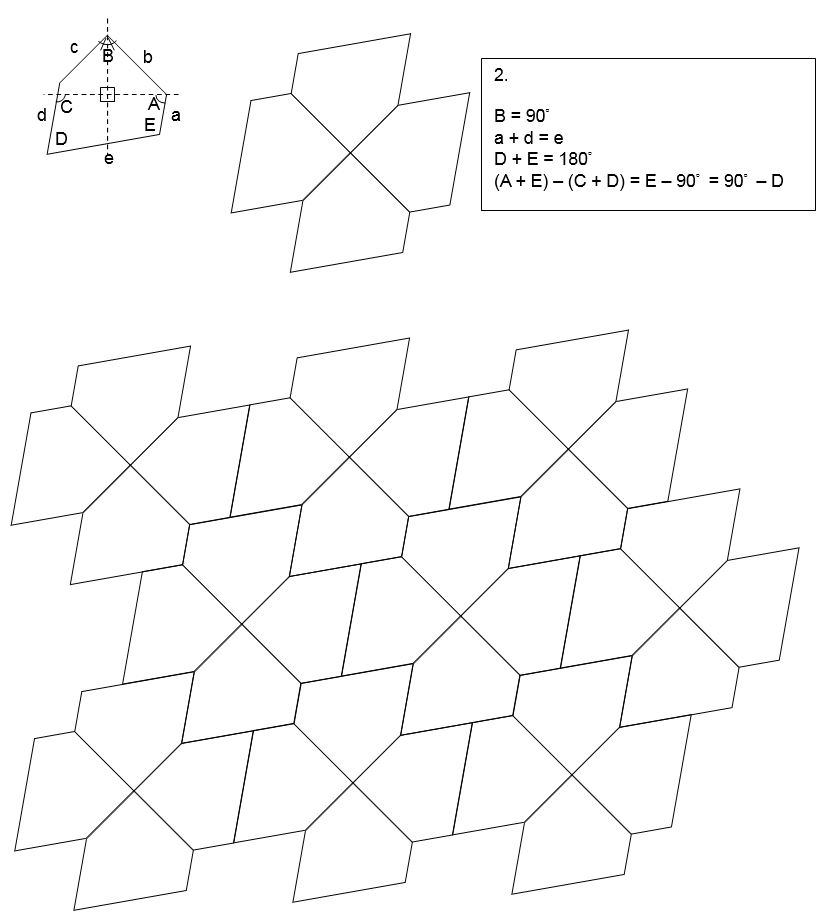
Inspired by Wikipedia's article on pentagonal tiling, I made my own attempt.
I believe this belongs to the 4-tile lattice category, because it's composed of pentagons pointing towards 4 different directions. According to Wikipedia, 3 out of the 15 currently discovered types of pentagonal tiling belongs to the 4-tile lattice category (type 2, 4 & 6).
https://en.wikipedia.org/wiki/Pentagonal_tiling
I don't think my attempt is an instance of type 4 or 6, since in both type 4 & 6, any side of all pentagons overlaps with only one side of another pentagon, while in my attempt, half of the long sides overlap with two shorter sides.
At the same time, I can't figure out how my attempt is an instance of type 2... Please kindly offer your insight.