Under $H_0$, the sequence of the (random) processes $(T_n(\lfloor n\,\cdot\rfloor)/\sqrt n)_{n=1}^\infty$ (you forgot to divide by $\sqrt n$) converges to the Brownian bridge $B^\circ(\cdot)$ in distribution.
Suppose that (as a specification of $H_1$) $h_n\sqrt n\to h\ne0$ and $k^*/n\to\kappa\in(0,1)$, where $h_n:=EX_n-EX_1$.
The distribution of the sequence $(X_1,\dots,X_n)$ under $H_1$ is the same as that of the sequence $$(Y_1,\dots,Y_n):=(X_1,\dots,X_{k*},X_{k^*+1}+h_n\dots,X_n+h_n)$$
under $H_0$, so that $Y_j=X_j+h_n I\{j>k^*\}$, where $I$ is the indicator function.
So, the distribution of the process $(T_n(\lfloor n\,\cdot\rfloor)/\sqrt n)_{n=1}^\infty$ under $H_1$ is the same as that of of the process $(\tilde T_n(\lfloor n\,\cdot\rfloor)/\sqrt n)_{n=1}^\infty$ under $H_0$, where, with $k:=\lfloor nr\rfloor$,
$$\frac{\tilde T_n(\lfloor nr\rfloor)}{\sqrt n}:=\frac1{\sqrt n}\sum_{j=1}^k(Y_j-\overline{Y})$$
$$=\frac1{\sqrt n}\sum_{j=1}^k(X_j-\overline{X})
+\frac{h_n}{\sqrt n}\sum_{j=1}^k\Big(I\{j>k^*\}-\frac1n\,\sum_{i=1}^n I\{i>k^*\}\Big)
$$
$$=\frac1{\sqrt n}\sum_{j=1}^k(X_j-\overline{X})
+h_n \sqrt n\, \psi_{k^*/n}(k/n)
$$
$$=\frac{T_n(\lfloor nr\rfloor)}{\sqrt n}
+h \psi_\kappa(r)+o(1),
$$
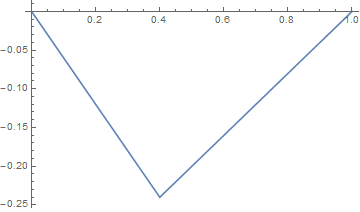
where in turn $\psi_u(s):=(u-1)s$ if $0\le s\le u$ and $\psi_u(s):=u(s-1)$ if $u\le s\le 1$.
It follows that, under the mentioned specification of $H_1$, the sequence $(T_n(\lfloor n\,\cdot\rfloor)/\sqrt n)_{n=1}^\infty$ of the processes converges to the process $[0,1]\ni r\mapsto B^\circ(r)+h \psi_\kappa(r)$ in distribution (say in the Skorokhod space $D[0,1]$), where $I$ is the indicator function. Below is the graph of the function $\psi_\kappa$ for $\kappa=0.4$.