A somewhat fanciful "application."
Suppose nodes represent museum guard stations, and arcs represent lines
of sight between stations.
The labels represent shift hours: hours at one station before a
guard is replaced by a fresh guard.
Then a prime labeling ensures that when there is a change of guard at one
station, there is not simultaneously a change of guard at all the adjacent
stations, until the lcm of the labels in the neighborhood
is reached.
So those guard(s) can monitor the change and ensure coverage.
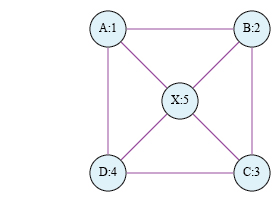
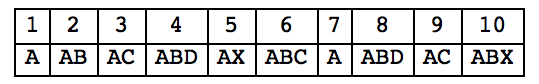
This schedule fails at $t=2 \cdot 3 \cdot 5 = 30$, when
no guard covers $B$.
Alternatively, the nodes can represent employees and the arcs
employees with similar skills, so again when a shift change occurs,
the skill set is covered during the switch.


