Ellipses on spheres (and other surfaces)

Question

Define an ellipse $E$ on a sphere as the locus of points whose sum of shortest geodesic distances to two foci $p_1$ and $p_2$ is a constant $d$. There are conditions on $\{ p_1, p_2, d \}$ for this definition to make unambiguous sense, but assume those conditions hold. (Added: Ian Algol specifies: $p \in E$ should not be a conjugate point of $p_1$ or $p_2$, i.e., the Jacobi field of a geodesic from a focus to a point $p$ of $E$ should not vanish at $p$.)

---

         

---

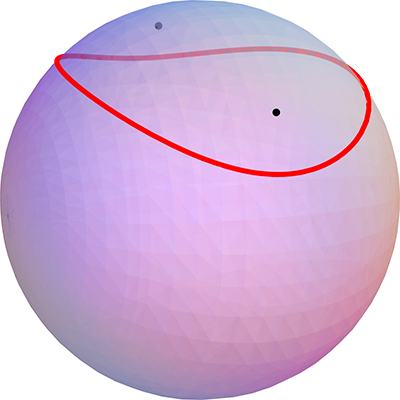
I am interested in billiard-reflection properties of ellipses on spheres, and on other curved surfaces. My initial searches for literature has turned up empty, although there seems little doubt the topic must have been studied. Here are a few questions.

Q1. For an ellipse $E$ on a sphere, does a geodesic ray from $p_1$, reflecting by angle-of-incidence = angle-of-reflection from $E$, necessarily pass through $p_2$?

Q2. If not, is there some other curve $C$ that has this property? In other words, could an ellipse be defined as a curve $C$ with the reflection property, rather than the sum-of-distances property? What is the relationship between the two possible definitions?

Q3. What are the properties of an ellipse (defined by the sum-of-distances property) on other curved surfaces? Constant negative curvature? Arbitrary smooth surfaces?

Thanks for ideas and/or pointers to the literature!

---

Answered by Ian Algol: The answer to Q1 is Yes, not just for spheres, but—remarkably—for any smooth surface. So this answers Q2 and Q3 as well.

Answer 1

The equiangular property follows from the properties of Jacobi fields. If we consider a point $p\in E$ on the ellipse, and geodesics $\alpha, \beta$ from $p$ to the foci $p_1, p_2$ respectively, then for a tangent vector $V$ to $E$ at $p$, we may decompose it uniquely as $V= A^T+ A^\perp = B^T+B^\perp$, where $A^T$ is tangent to $\alpha$ at $p$, $A^\perp$ is perpendicular to $\alpha$ at $p$, and similarly for the $B^T, B^\perp$ with respect to $\beta$. Then $V$ extends uniquely to Jacobi fields for the geodesics $\alpha$ and $\beta$ vanishing at $p_1$ and $p_2$ respectively (assuming $p$ is not a conjugate point of $p_1$ or $p_2$, which presumably is built into your unspoken assumptions). These Jacobi fields represent the derivatives of the (parameterized) geodesics connecting points on $E$ to $p_1, p_2$ as one varies the point along $E$ in the direction $V$. Since the sum of the lengths is preserved, one has that the variation of $\alpha$ has length changing as the negative of the change of length of $\beta$. But the Jacobi field associated to $A^\perp$ and $B^\perp$ does not change the length of $\alpha$ or $\beta$ (edit: this fact also works on any surface, essentially by the Gauss Lemma). So the variation in length is determined by the magnitude of $A^T$ and $B^T$. In particular, these are of the same length, and point in opposite directions along $\alpha$ and $\beta$ respectively. Then we compute the angle: $$\cos(\angle V A^T)=\langle V, A^T\rangle / (|V||A^T|) = \langle A^\perp + A^T, A^T\rangle /(|V||A^T|) = \langle A^T,A^T\rangle / (|V||A^T| )= |A^T|/|V|=|B^T|/|V| = \cos(\angle V B^T).$$ Thus, the two angles are equal.

Answer 2

I'd like to add that the same holds not only for Riemannian, but also for Finsler metrics: E. Gutkin, S. Tabachnikov. Billiards in Finsler and Minkowski geometries J. Geom. Phys. 40 (2002), 277-301. Of course, the law of reflection should be defined appropriately.

My explanation of the optical property of ellipses is as follows (tested on students). Let A and B be the foci. Consider the distance functions to A and to B. The gradients of these functions at point X are the unit vectors along the geodesics AX and BX. The sum of these unit vectors is orthogonal to the ellipse, a level curve of the sum of the functions. This implies that the angles are equal, and the ray AX reflects to the ray XB.