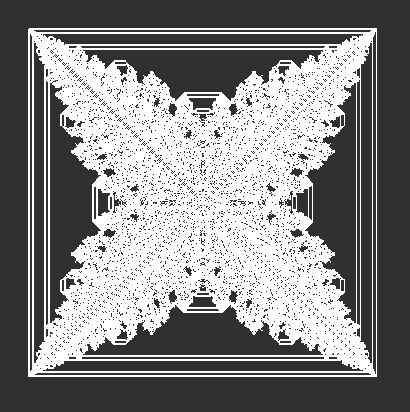
In Conway's game of life, take the initial position to be two infinite diagonal lines of live cells, with a single cell in common. Does this thing converge to a stable configuration? I.e., is the state of each cell (or finite region) eventually periodic?
1
-
5$\begingroup$ What kind of experimental evidence do you have for large finite approximations? $\endgroup$– Joel David HamkinsCommented Jun 4, 2013 at 2:01
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
What I get from an X of size $11121\times11121$ at just around the point where information travels to the tips.
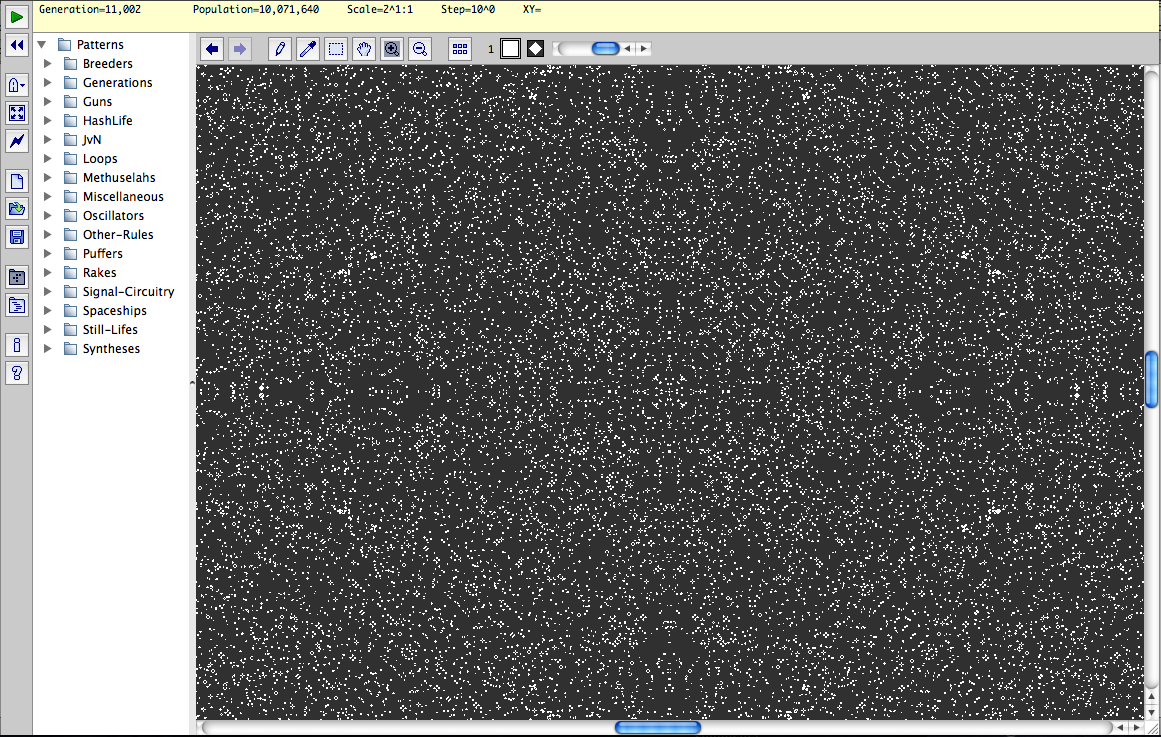
Even from Xs ten times as long, there is Methuselah-like ebbing and flowing of debris near the center amid a pool of still lifes and blinkers still thousands of generations on.
Just going from experience working on the Busy Beaver of 5, I would imagine this question might be enormously difficult to settle, owing to the globally fractal and locally random nature of the picture.
