distance between powers of 2 and powers of 3

Question

I expect this is a classical question, so feel free to point me to classical answers: what is the fastest-growing function $f(t)$ for which we know that $$ |2^t - 3^{t'}| \ge f(\min(t,t')) \;? $$ In particular, do we know that the gaps between powers of 2 and powers of 3 get exponentially large as $t,t'$ increase? Do we know anything like this for any other pair of integers besides 2 and 3?

Answer 1

What you need is the theory of lower bounds for linear forms in logarithms. A good place to start reading about this is the following article by Evertse:

www.math.leidenuniv.nl/~evertse/dio2011-linforms.pdf

In particular, Corollary 1.8 of the article (a Corollary to a famous theorem of Matveev) gives

$$ \lvert 2^a-3^b \rvert \ge \frac{\max(2^a,3^b)}{(e \max(a,b))^{C}} $$ where $C$ is a positive constant (that is easily computable--see the proof and also the statement of Theorem 1.7).

Answer 2

I guess you expect $t$ and $t'$ to be integers. In this case, having a small $2^p-3^q$ is related to having a small $\frac{\log 3}{\log 2} - \frac{p}{q}$. So it's Diophantine approximation, and this is very well studied. The first result in Diophantine approximation is that there exists an infinity of rational $p/q$ such that $$ \left|\frac{\log 3}{\log 2} - \frac{p}{q}\right| < \frac{1}{q^2}. $$ In which case it's not hard to compute that $$ \left| 2^p - 3^q \right| = \mathcal{O}\left( \frac{3^q}{q} \right). $$ This is valid of course for all $2$'s and $3$'s.

If now you want lower bounds, then you will need to know a upper bound for the irrationality measure of $\frac{\log 3}{\log 2}$, which is hard to get, but hopefully someone did it. Do you want more details ?

EDIT

Let $\epsilon = p \log 2 - q \log 3$. In particular, $|\epsilon| < \frac{\log 2}{q}$. We compute that $$\left| 2^p - 3^q \right| = 3^q \left| 1 - \exp(\epsilon) \right|$$

As $q\to \infty$, we have $\epsilon \to 0$ so $1-\exp(\epsilon) = \mathcal{O}(\epsilon) = \mathcal{O}(q^{-1})$. Thus $$ \left| 2^p - 3^q \right| = \mathcal{O}\left( \frac{3^q}{q} \right). $$

Answer 3

I was a little hesitant to post the following thing after the very thorough answers and references, yet it contains a concrete inequality, and may be of interest as a first elementary approach towards the full complexity of the problem.

The idea is that if $2^t$ and $3^ {t'}$ are too close to each other, then $2^{t+1}$ is close to $2\cdot3^{t'}$, hence it is roughy in the middle between $3^ {t'}$ and $3^ {t'+1}$, and therefore far from any power of $3$. To make this into a more quantitative form: assume that $t$ and $t'$ satisfy $$|2^t -3^ {t'}| < \frac{1}{5} 2^t\, .$$ Then it follows plainly

$$ 3^ {t'} + \frac{1}{5} 2^t < 2^{t+1} < 3^ {t'+1} - \frac{2}{5} 2^t \, .$$ Therefore the closest power of $3$ to $2^{t+1}$ is either $3^ {t'}$ or $3^ {t'+1}$, in any case not closer than $ \frac{1}{5} 2^{t+1}$. This tell us that the inequality $$\min _ {t'\in\mathbb{N}} |2^t -3^ {t'}| > \frac{1}{5} 2^t$$ holds for at least one out of two consecutive integers $t$ and $t+1$. So at least half of the powers of $2$, in a density sense, have a distance from the powers of three of at least one fifth of their size.

Answer 4

Just to satisfy the curiosity of @FelixGoldberg and other cursory readers. Here is a heuristic which pointed me to try to use $C=1.06$ for an example.

We look at the distances

$$\left|1-{3^b\over2^a}\right| \overset{???}\ge { 1\over (e \cdot a)^C} $$

with $a \gt b$ and $2^a \gt 3^b$ (fixing the $\max()$-terms.

In the table $w=\log_2\left|1-{3^b\over2^a}\right| $ and $u=-\log_2 (e a)$. The quotient $w/u$ should give an impression of the missing factor $C$, and in this table for all except $2$ cases ( idx=15,idx=21 ) a value of $C=1.06$ suffices to make the inequality true.

The table reports the cases according to the continued fraction of $ß=\log_23)$ so only the best possible approximants (with $2^a \gt 3^b$) are displayed (the convergents, each second of them)

  idx   b     a     log2(b)  log2(a)      w       u         w/u      1.06*u
----------------------------------------------------------------------------
   3      1      2  0.E-201  1.00000  -2.00000  -2.44270  0.818768  -2.58926
   5      5      8  2.32193  3.00000  -4.29956  -4.44270  0.967782  -4.70926
   7     41     65  5.35755  6.02237  -6.45514  -7.46506  0.864714  -7.91297
   9    306    485  8.25739  8.92184  -9.93479  -10.3645  0.958537  -10.9864
  11  15601  24727  13.9294  14.5938  -15.7461  -16.0365  0.981894  -16.9987
  13  79335  SSSSS  16.2757  16.9401  -18.0579  -18.3828  0.982323  -19.4858
  15  NNNNN  SSSSS  17.5397  18.2042  -23.8860  -19.6469   1.21576  -20.8257
  17  NNNNN  SSSSS  23.3620  24.0265  -26.2877  -25.4692   1.03214  -26.9973
  19  NNNNN  SSSSS  27.3572  28.0217  -29.0580  -29.4644  0.986209  -31.2322
  21  NNNNN  SSSSS  28.5666  29.2311  -33.1373  -30.6738   1.08031  -32.5142
  23  NNNNN  SSSSS  32.6169  33.2814  -36.5236  -34.7241   1.05182  -36.8075
  25  NNNNN  SSSSS  37.0009  37.6654  -40.0173  -39.1081   1.02325  -41.4546
  27  NNNNN  SSSSS  42.2986  42.9630  -43.7861  -44.4057  0.986046  -47.0701
  29  NNNNN  SSSSS  43.3957  44.0601  -46.1400  -45.5028   1.01400  -48.2330
  31  NNNNN  SSSSS  48.6152  49.2797  -49.4134  -50.7224  0.974193  -53.7657
  33  NNNNN  SSSSS  52.3527  53.0172  -53.0620  -54.4599  0.974331  -57.7275
  35  NNNNN  SSSSS  56.8562  57.5206  -58.9521  -58.9633  0.999810  -62.5011
  37  NNNNN  SSSSS  58.4640  59.1284  -62.5155  -60.5711   1.03210  -64.2054
  39  NNNNN  SSSSS  62.0089  62.6734  -65.0073  -64.1161   1.01390  -67.9630
  41  NNNNN  SSSSS  64.5731  65.2376  -66.4207  -66.6803  0.996108  -70.6811
  43  NNNNN  SSSSS  66.0744  66.7389  -67.9931  -68.1815  0.997236  -72.2724
  45  NNNNN  SSSSS  67.4786  68.1431  -73.2504  -69.5858   1.05266  -73.7609
  47  NNNNN  SSSSS  74.7217  75.3861  -81.0514  -76.8288   1.05496  -81.4386
  49  NNNNN  SSSSS  80.5354  81.1999  -82.0659  -82.6426  0.993022  -87.6011

---

The Pari/GP script is

fmt(200,8) \\ internal precision 200 dec digits, user-procedure
{e=exp(1);l3=log(3);l2=log(2);ld3 = l3/l2;
cf = contfrac(ld3);
cvgts= mkContFracConvergents(cf,50) ; \\ user-procedure
listlogs=vectorv(50);ix=0;
forstep(i=3,50,2,
          a=cvgts[1,i];      \\ ===> a > b  and also 2^a > 3^b
          b=cvgts[2,i];   
          ix++; listlogs[ix]=[i,
                 if(b<100 000,b,'NNNNN),      if(a<100 000,a,'SSSSS),
                 log(b)/l2,                   log(a)/l2,
                 w=log((1.0-2^(ld3*b-a)))/l2, u=-log(e*a)/l2,
                 w/u      ,                   1.06*u];
        );
 listlogs=Mat(VE(listlogs,ix))}

Remark: a bit more introduction and tables and graphs for $b \to 10^{10800} \approx 2^{36000} $ can be found at my pages . Note, that I use $N$ for what we use $b$ here, and $S$ for what we use $a$ here, thus discussing $2^S-3^N$.

---

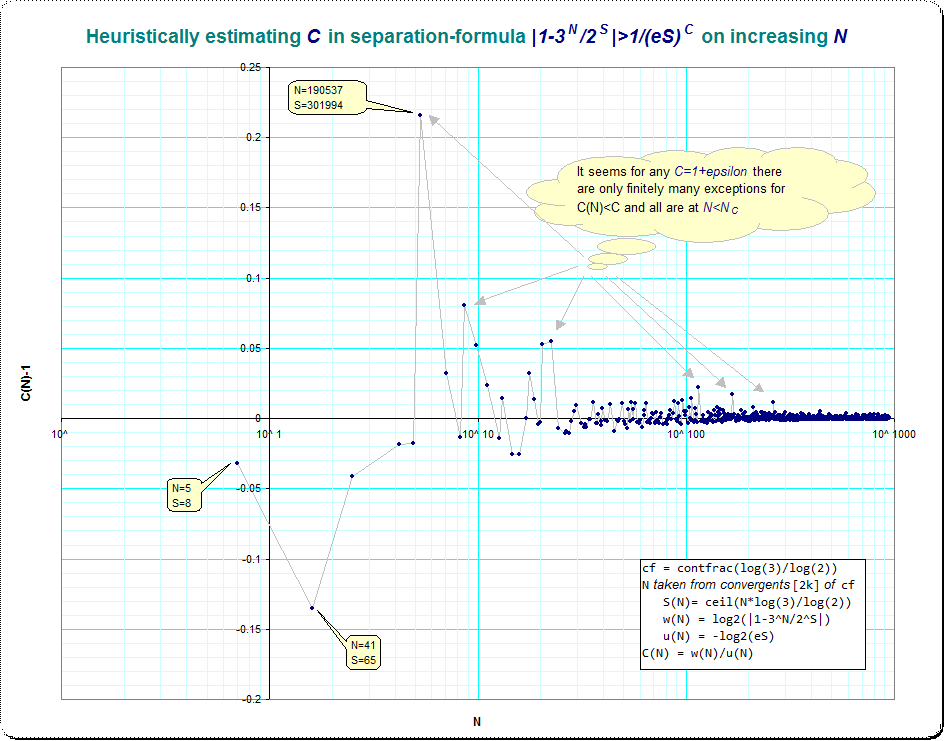
update A better visualization of the properties of selecting some constant $C=1+\epsilon$ using up to $b =10^{1000}$ taken from the convergents of the continued fraction of $\log(3)/\log(2)$
I show how empirically the values of $C(b)$ were when $a,b$ are inserted in the formula and $C(b)$ is computed. The image shows, that the empirical $C(b)$ are except in two cases smaller than $C=1.06$ and moreover, that possibly we can choose any $C=1+\epsilon$ and getting only finitely many cases where not $C(b) \le C$
Legend: In the picture I used my standard-notation $N$ for $b$ here and $S$ for $a$ here.